Method Transfer in HPLC
Many HPLC analyses could be performed at lower expenditure. This could involve a combination of reducing the analysis time, reducing the resolution between critical peaks, and lowering the consumption of mobile phase. Successfully optimizing the method in such instances - as well as in situations where it is necessary to transfer the method to another laboratory that lacks the same selection of columns - can save the analyst time and money.
For a successful method transfer column dimensions and flow-rates need to be considered. It may be necessary to re-calculate them. The mathematics are simple and should not present an obstacle, although some transfers are more complicated than others. Let us begin with the simple ones. The starting point is a method that has been successfully developed, but has perhaps been "over-solved" because the resolution is higher than is required.
Shorter Columns
For quantitative analysis it is best to look for baseline resolution which means that R should be 1.3 or higher. For peaks that differ greatly in size a high resolution of 2.0 or more is needed.1,2 However, if the resolution of the peaks to be quantified is much higher than required it is possible to use a shorter column (with an identical stationary phase and packing quality).

Keynotes
The resolution is proportional to the square root of the theoretical plate number N (this is explained in every HPLC textbook), and N is proportional to the column length Lc. Therefore:

Example 1: The critical resolution (the lowest resolution of a peak pair to be quantified) is now 3.2 but it can be lowered to 2.0 without a problem. So if a 15 cm column was initially used:
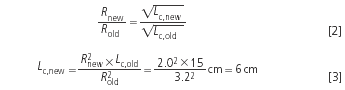
The new column is much shorter, yielding shorter analysis times and less solvent consumption.
If a shorter column is used, the extra-column volumes of the HPLC instrument need to be evaluated critically because the peaks are now eluted in a smaller volume. If the former instrument/column configuration was at its limit with regard to these volumes, the peaks will now be broader than expected. The volumes to be considered are the injection volume,3 the connecting capillaries,3,4 and the detector cell.3 For gradient separations the dwell volume5 is also important.
Thinner Columns
A column of 4.6 mm inner diameter dc offers no advantages in terms of resolution, but it is thirstier than a thinner one so consumes more solvent. As explained previously, if an HPLC instrument with adequate extra-column volumes is used, it is possible to use a narrower column without any loss of separation performance. When looking at tubes of different inner diameters it is obvious that the flow-rate, F, needs to be adapted to get identical retention times. The relationship varies with the square of the diameter because the area of the respective column profile is a circle:
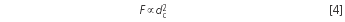
Example 2: The 4.6 mm columns used so far were replaced by 3.0 mm columns (this is not a revolutionary step). The flow-rate was 1.2 mL/min.
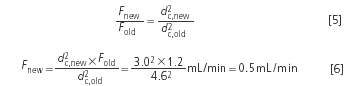
The saving in solvent consumption is almost 60%!
Smaller Particle Diameters
If a column with an identical diameter and length, but a smaller particle diameter is used, an increase in the theoretical plate number and the pressure will be observed. The chromatographic resolution will be slightly different because the new conditions will result in another position of the separation system within the van Deemter curve. To benefit from the smaller particles it is highly recommended to increase the flow-rate. The relevant parameter is the so-called reduced velocity ν (dimensionless), which is calculated from the linear flow-rate, u (measured in, for example, mm/s), the particle diameter, dp, and the diffusion coefficient, Dm, of the analyte molecules in the mobile phase.6

The reduced velocity is the mathematical representation of the fact that the analyte molecules travel into and out from the stationary phase particles by diffusion and not by flow. With a coarse stationary phase the mean resident time within a particle will be longer than with a fine one, therefore, the flow-rate should be low when large-diameter particles are in use and vice versa. The flow-rate of the eluent can — and should be — increased when the method is transferred to a column with finer packing. The goal is to keep the reduced velocity constant.
If the old method used a 5 μm packing in a 4.6 mm column at a flow-rate of 1.2 mL/min, the linear flow was approximately 1.9 mm/s (depending on the total porosity of the packing).7 Together with a diffusion coefficient of 6 × 10–4 cm2 /min, which is typical for small molecules in water/acetonitrile, the former reduced velocity was approximately ν = 9, although this number is a rough guess. The actual diffusion coefficient depends on the properties of the analyte, on the composition of the eluent and on the temperature. In addition, the shape and the actual minimum of the van Deemter curve also depends on the properties of the column packing. (Note that the van Deemter optimum is at ν = 3 in most cases; higher reduced velocities mean that the usual flow-rates are too high and that some separation power of the column is sacrificed for faster analyses).
The relationships are linear in this instance:
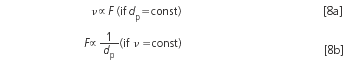
Example 3: It is planned to use 3 μm packings in the future instead of 5 μm. The column manufacturer guarantees identical packing quality. The flow-rate so far was1.2 mL/min.
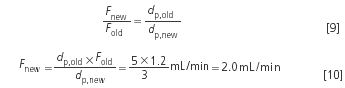
What about the pressure rise? This will depend on if the intention is to keep the column length or the theoretical plate number (and thus also the resolution) constant. If the length is unchanged a higher plate number and a markedly higher pressure Δp is obtained as a result of the two relationships:
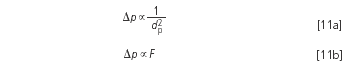
Example 4: The pressure was 80 bar for the 5 μm phase. Now a 3 μm column of identical length is used. The flow-rate is increased as calculated by Equation 10:
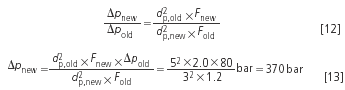
However, it only makes sense to use a longer column with smaller particles if the old separation was unsatisfactory. If the goal is to keep the plate number and thus also the resolution constant it is now possible to use a shorter column. The relevant relationships are:
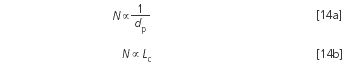
Example 5: The critical resolution is intended to be constant at 2.0. So far the separation was performed with a 12 cm column. If the theoretical plate numbers are identical with both the 5 μm and the 3 μm phases, then the resolution is also identical:
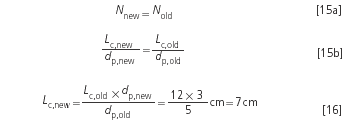
The new column is shorter by a factor 3:5 = 0.6. This means that also the pressure drop is only 0.6 times the value as calculated with Equation 13, namely 370 × 0.6 bar = 220 bar.
A decrease of the particle diameter is always "paid" with a remarkable increase of the pressure drop. This fact is even observed if the flow-rate is not increased as recommended above (as a result of the shorter diffusion paths) and calculated with Equation 10. The relationships are explained in the following.
The Clever Use of Pump Pressure
This strategy is the fine art of method transfer. The simplest way to decrease the analysis time is to increase the flow-rate. The pressure drop will increase linearly, a well-known fact of everyday laboratory work. In most instances some loss of resolution will be observed because the new conditions are further away from the van Deemter optimum. The analysis will be completed in a shorter time and the amount of eluent needed is constant.
To transfer the original resolution to the new conditions — and to get more efficient working conditions — it is best to decrease the particle size, to use a shorter column and to increase the flow- rate as well. The pressure will increase but the analysis time drops by the same factor and the amount of eluent decreases. Thus the strategy "smaller particles, shorter column" generates true added value from the available pressure because less mobile phase is needed. The pressure will increase linearly with both the decreased particle diameter and the increased flow-rate, therefore, it is the square root of the pressure ratio, PR, that is needed to calculate the new conditions:
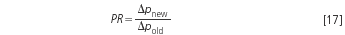
The new particle size is obtained by:
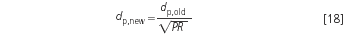
The result implies the new flow-rate, as given with Equation 10:
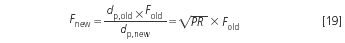
The length of the new column is obtained in a similar way to the new particle size:
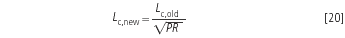
and if the column diameter is unchanged, the savings in eluent volume, V, are:
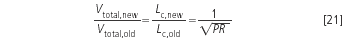
Table 1 shows an example with a doubling of the pressure (√PR = √2 = 1.4). The calculated numbers are rounded and in real life the users will select the new conditions according to the column and particle sizes available on the market. Figure 1 presents a seven-fold gain in speed with a seven-fold increase of the pressure (PR = 7) and without a loss in performance. Similar comparisons have been published by researchers8 and by HPLC companies9,10 (without pressure discussion). Ultra-high pressure LC (UPLC) offers even the possibility of working at pressures up to 1000 bar.11,12

Table 1: Change of the parameters when it is possible to work with doubled pressure. The column diameter remains unchanged.
If the column length and the particle diameter are changed simultaneously, the pressure ratio is:
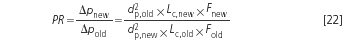
This relationship is always valid and does not depend on whether the separation was altered towards an optimization of pressure, as described, or if the changes were unreflected or even nonsensical.
If, at the same time, a narrower column is used (see Figure 1), the flow-rate needs to be decreased even more (as shown by Equation 6).
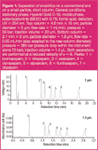
Figure 1
Consider Ruggedness!
When methods are optimized or transferred, one must not forget the importance of ruggedness. A method is rugged if its results are not influenced by small variations of the separation conditions (such as temperature or pH).13 If a method has been optimized with the goal of running at the lowest reasonable resolution of the critical peak pair, then the separation will deteriorate soon as a result of the inevitable ageing of the column. It is highly recommended to keep some extra-resolution in all methods described in standard operating procedures (SOPs).
Is it Necessary to Re-validate?
Usually a re-validation is not needed if a formerly validated method is transferred as described above. Passing the system suitability test14 should do. In addition, the transfer steps and the considerations involved need to be documented and explained to understand the transfer process. Calibration curves or response factors must be determined again as a matter of course! It would be totally wrong to use them again (in the same way that it is wrong to perform quantitative analysis series without an updated calibration).
Stationary Phase Factors?
If two stationary phases have exactly the same commercial name it is reasonable to assume that their chromatographic properties are independent of the particle diameter. When transferring from 5 μm to 3 μm one can expect almost (or actually) identical separations (under identical reduced velocity). This observation is no longer true if another material is used. Even a change of the pore width may be seen in the chromatogram. Another product, which may have a similar name such as "RP-18", can have completely different properties.15 If a certain stationary phase will do the job nicely there is no reason to use another material. A change of the stationary phase is no longer a "method transfer" but it leads to a new method which needs to be validated again.
Gradient Separations
If the column dimensions or the particle size of gradient separations are going to be changed, the consequences for the gradient profile should not be forgotten. As simple rules of thumb we may note three different scenarios:16
Shorter column: The analysis time is shorter and the gradient run time must be adapted proportionally.
Thinner column: The run time of the gradient remains unchanged. The flow-rates of both eluents must sum up to the calculated Fnew.
Smaller particle size: The flow-rate should be increased, as explained previously. In addition, the set-up "shorter column" is attractive, (i.e., the procedure as described under "The Clever Use of Pump Pressure"). The gradient must be steeper to obtain the final %B conditions within the calculated Vtotal.
Gradient separations are more intricate than isocratic ones. The samples to be investigated have a more complex composition (otherwise no gradient would be needed). It is very possible that a complete re-validation has to be performed because a simple system suitability test does perhaps not reveal all the method properties such as broad-range linearity or detection limit.
Davy Guillarme gained his PhD from the Univeristy of Lyon, France in 2004 and is currently maitre assistant in the Laboratory of Pharmaceutical Analytical Chemistry of the University of Geneva and the University of Lausanne, Switzerland. His interests include the development of new approaches to perform fast and ultra-fast separations in liquid chromatography and the possibility to hyphenate these techniques with alternative detection modes.
Jean-Luc Veuthey obtained his PhD from the University of Geneva, Switzerland in 1987. He is now a full professor in the Department of Pharmaceutical Sciences, University of Geneva and University of Lausanne, Switzerland. His interests include the development of LC and CE hyphenated to several detection modes for the analysis of drugs and metabolites. The sample preparation and the validation of the procedures are also particularly studied in his laboratory.
Veronika R. Meyer is a specialist in HPLC and measurement uncertainty. She obtained her PhD in 1989 from the University of Bern, Switzerland and now works as a research scientist at EMPA St Gallen, Switzerland, as well as teaching at the University of Bern. She is known as the author of "Practical High-Performance Liquid Chromatography", 4th edition 2004, and "Pitfalls and Error Sources of HPLC in Pictures", 2nd edition 2006 (both at Wiley).
References
1. L.R. Snyder, J. Chromatogr. Sci., 10, 200–212 (1972).
2. V.R. Meyer, J. Chromatogr. Sci., 33, 26–33 (1995).
3. M. Martin, C. Eon and G. Guiochon, J. Chromatogr., 108, 229–241 (1975).
4. R.P.W. Scott and P. Kucera, J. Chromatogr. Sci., 9, 641–644 (1971).
5. L.R. Snyder and J.W. Dolan, LCGC Int., 3 (10) 28–39 (1990).
6. P.A. Bristow and J.H. Knox, Chromatographia, 10, 279–289 (1977).
7. V.R. Meyer, Practical High-Performance Liquid Chromatography, Fourth Edition, Paragraph 8.3 (Wiley, Chichester, UK, 2004).
8. R.W. Stout, J.J. DeStefano and L.R. Snyder, J. Chromatogr., 261, 189–212 (1983).
9. R.J. Jonker and G.P. Rozing, Hewlett-Packard Journal, April 1984, p. 4; also shown as Figure 7.3 in Ref. 7.
10. D.M. Diehl et al., LCGC Eur. Appl. Book, Sept. 2003, 20–21.
11. D.T.T. Nguyen et al. J. Sep. Sci., 29, 1836–1848 (2006).
12. R. Lake, The Restek Advantage, 4, 2007, 10–11.
13. J.W. Dolan, LCGC Eur., 19, 268–272 (2006).
14. J.W. Dolan, LCGC Eur., 17, 328–332 (2004).
15. B.A. Olsen and G.R. Sullivan, J. Chromatogr. A, 692, 147–159 (1995), as just one example.
16. L.R. Snyder, J.J. Kirkland and J.L. Glajch, Practical HPLC Method Development, Second Edition, Chapter 8.4 (Wiley-Interscience, New York, USA, 1997).
LCGC’s Year in Review: Highlights in Liquid Chromatography
December 20th 2024This collection of technical articles, interviews, and news pieces delves into the latest innovations in LC methods, including advance in high performance liquid chromatography (HPLC), ultrahigh-pressure liquid chromatography (UHPLC), liquid chromatography–mass spectrometry (LC–MS), and multidimensional LC.
Using LC-MS/MS to Measure Testosterone in Dried Blood Spots
December 19th 2024Testosterone measurements are typically performed using serum or plasma, but this presents several logistical challenges, especially for sample collection, storage, and transport. In a recently published article, Yehudah Gruenstein of the University of Miami explored key insights gained from dried blood spot assay validation for testosterone measurement.