When Peaks Collide, Part III

The previous installment in this series looked at how moderate peak tailing affects resolution and degree of peak overlap. When peaks tail, even moderately, extra time between them is required to attain the same resolution as for nontailing peaks due to the overall increase in peak widths caused by tailing. Even when the same resolution is attained, however, the degree of area overlap between tailing peaks is greater than for nontailing peaks, and more so for the amount of excursion of the first peak into the second. Thus, additional resolution is required for tailing peaks to achieve the same degree of peak area overlap as a pair of nontailing symmetrical peaks.

The amount of area overlap of one peak into another can be measured as the percentage of a peak's area that lies underneath the adjacent peak, where the peaks are separated with a specific degree of resolution, as in the previous two installments in this series (1,2). In that case, where the peaks were of equal size, resolution of 2.0 was needed to bring the degree of overlap of a first tailing peak into the second down to about the same level seen with symmetrical peaks at resolution of 1.5, at around 0.5%. This was despite the moderate increase in peak separation required to keep the measured resolution the same for tailing peaks as for nontailing ones.

The degree of peak overlap can be calculated easily enough with the theoretical peak model being used here, but it isn't a realistic measurement of the effects that less-than-ideal peak separation has on actual area measurement of peaks that are co-eluted in a chromatogram. With nonselective detectors, it isn't possible to measure the two peaks independently, as was done here to obtain the degree of overlap. Even with a mass spectrometer or other selective detector, there often isn't sufficient selectivity to obtain 100% discrimination between overlapping peaks, so questions of the effects of peak overlap on quantitative measurements are not answered by overlap calculations.

In some cases, mathematical peak deconvolution can be used to tease apart two partially merged peaks, but practically speaking, for routine applications, we have to fall back on the integration parameters available in chromatography data processing systems. To test how well merged tailing peak areas are estimated, I exported a series of synthesized chromatograms with moderately tailing peaks using asymmetries (A_S) of 1.4 in which the peak resolution was set at 1.0, 1.5, and 2.0. Three common peak integration methods were selected for the estimation of peak areas: a vertical drop from the valley point between the peaks down to the baseline; a tangential skim drawn from the valley out to the down-sloping side of the second peak; and an exponential skim underneath the second peak. Somewhat unrealistically, no noise was added to the generated peaks, but in this case, I was more interested in the measurements themselves and not the degree of uncertainty across multiple measurements. These results show how one particular data processing system performed. Others would vary, but I expect they might produce very similar results.

Table I enumerates the results of this tailing peak faceoff, and Figure 1 shows what the separations looked like at resolution = 1.0. As was the case with symmetrical peaks (1), increasing peak separation and resolution improved the accuracy of the area measurements significantly. And the influence of the area determination method influenced the measurements in much the same manner. The best results were obtained with a simple vertical drop from the valley between the peaks to the baseline below; the measured values had less than a 4% error in all cases. The exponential skimming technique performed well except for the second, smaller peak when the resolution was less than 1.5. In this case, the error went from about 6% up to nearly 35%. The tangential method delivered a comparably large error of over 8% for the second of two relatively well-separated peaks, and it produced very large errors for the second peak in the cases of the less-resolved peak pairs.

Table I: Area measurement of partially resolved tailing peaks. Peak 1 is 10× larger than peak 2; both have asymmetries of 1.4. The appearance of the peaks at resolution = 1.0 is illustrated in Figure 1, to which the letters (a), (b), and (c) refer.

Overall, at resolution 1.0, the valley baseline error for the second, smaller peak was actually somewhat less for the unequal-sized tailing peaks than for the symmetrical peaks, due perhaps to the slightly larger separation of the tailing peaks, while errors for the tangential and exponential methods were consistently larger with unequal-sized tailing peaks than for symmetrical ones. This seems to say that the increased separation between tailing peaks that was required to maintain a degree of area overlap originally seen with nontailing peaks is insufficient to reduce peak area integration errors to similar levels in both cases.

Figure 1: Influence of integration method on the measured areas of unequal sized asymmetrical peaks. Peak pair with Rs = 1.0 (a) Vertical drop from valley to baseline; (b) tangential skim underneath the second peak; (c) exponential skim off the first, larger peak.

Another observation gave me second thoughts about peak integration in these situations. In the default state, chromatography data handling software has a number of settings that control various automatic thresholds for the onset of exponential skimming versus the vertical drop or other integration methods. These settings were left at their default values for this short study, which caused the automated selection of the area measurement method to change from a vertical drop in the case of resolutions equal to 1.5 and 2.0 to the exponential skim method at resolution of 1.0. The result was a sudden "automatic" decrease in the second peak's area when the resolution dropped off to 1.0, as determined without any intervention, a change that would have resulted in a -34.4% error if the system were allowed to run unobserved. As it was, I forced the use of the vertical drop method to observe its effects. Although I did not explore the effects of changing the relative sizes of the peaks to values other than 1:1 and 10:1, changes in peak size also can trigger unintended shifts in the integration method selected automatically by any data handling system. Therefore, in low-resolution situations, careful examination of the results over the entire range of intended magnitudes of all affected peaks, both analyte and matrix components, must be performed while taking positive control of the integration settings.

Measurements Related to Resolution

The aforementioned issues with peak area determination and resolution arise partially from the fact that peak resolution does not depend upon the relative sizes of the peaks. Thus, even though the area measurements of two peaks of equal size, tailing or not, can be quite different compared to measurements of two peaks of significantly different sizes, the resolution of the two pairs of peaks is identical: it depends only upon the peak widths measured at base, or on the widths at half-height — as are sometimes easier to measure — converted to base widths.

A number of measurements related to resolution have been described in the chromatography literature, including the peak capacity, which is the number of additional peaks of a specified resolution that could fit between two well-separated peaks. For example, if two peaks have resolution R_S = 6, then three additional peaks of the same width would fit between them if the added peaks were spaced exactly evenly in-between, for a peak capacity of 3. A similar term, the effective peak number, refers to the peak capacity in the special case where the resolution between added evenly spaced peaks equals 1.0. The separation number, or Trennzahl, uses the peak widths at half-height to calculate the number of peaks that would fit between our well-separated pair with R_S = 1.177 and usually is applied to consecutive members of a homologous series of compounds such as n-hydrocarbons in retention index calculations. Details of these measurements can be found in reference 3 as well as in many other chromatography textbooks.

Another resolution-related measurement is of interest to the current discussion. As stated earlier, none of these calculations takes into account the effect of differing peak sizes on peak area overlap and integration errors. The discrimination factor, however, does incorporate peak height and it can be a more meaningful metric for evaluation of these concerns (4). The discrimination factor is related to the separation power originally described by Kaiser (5, see also reference 3 for more information) and is calculated graphically as shown in Figure 2 from the relative heights of the smaller peak and the valley above the baseline.

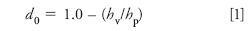
In Figure 2a, the discrimination factor is d₀ = 0.72 in the case of equal-sized symmetrical peaks and in Figure 2b with unequal-sized symmetrical peaks, d₀ = 0.30. In both cases the resolution equals 1.0, but the smaller discrimination factor of the peak pair with a 10:1 height ratio in Figure 2b reflects more accurately the reduced degree of separation available when the peak sizes are significantly different.

Figure 2: Measurement of the discrimination factor: (a) peaks of equal height; (b) peaks of unequal height.

In the case of tailing peaks of equal heights at resolution 1.0 (1), the discrimination factor also equals 0.72. The discrimination factor does not distinguish between tailing and symmetrical peaks with the same size and resolution, but remember that the tailing peak pair are separated in time by a few more seconds to keep the same resolution as the nontailing peak pair. In the case of the unequal size tailing peak pair with a 10:1 size ratio (Figure 1a), the discrimination factor drops down to 0.04. Compared to a value of 0.30 for the unequal-sized symmetrical peak pair, the effect of peak tailing on the discrimination factor is dramatic, perhaps even overstated. Nevertheless, the discrimination factor does provide a useful indication of how much a larger peak will affect the quality of separation with an adjacent peak both in terms of the peak size and the presence of peak tailing.

Conclusion

The effects of peak size and peak tailing on the degree of area overlap and measured peak areas are significant. Changes in peak separation, resolution, and symmetry strongly affect the accuracy of peak area measurements. Conventional resolution measurements do not take into account the effects of disparities in peak size, nor do they completely accommodate the effects of peak tailing. The peak discrimination factor provides some indication of how well a given separation discriminates adjacent peaks from each other and does take into account the effects of peak size and asymmetry.

Careful evaluation of the data processing and area measurements of disparate, partially merged, or tailing peaks can identify potential pitfalls that can be imposed by changes in concentration, peak retention time drift, and asymmetry incurred as chromatography components age and are replaced.

John V. Hinshaw John V. Hinshaw "GC Connections" editor John V. Hinshaw is senior Research Scientist at Serveron Corp., Hillsboro, Oregon, and a member of LCGC's editorial advisory board. Direct correspondence about this column to LCGC, Advanstar Communications, 485 Rt. 1 S, Bldg F, 1st Floor, Iselin, NJ 08830, or contact the author via e-mail: lcgcedit@lcgcmag.com

References

(1) J.V. Hinshaw, LCGC North America 28(7), 524–529 (2010).

(2) J.V. Hinshaw, LCGC North America 28(10), 874–878 (2010).

(3) L.S. Ettre and J.V. Hinshaw, Basic Relationships in Gas Chromatography (Advanstar, Cleveland, 1994), pp. 75–83.

(4) M.Z. El Fallah and M. Martin, Chromatographia 24, 115–122 (1987).

(5) R. Kaiser, Gas-Chromatographie (Akademische Verlagsgesellschart, Leipzig, 1960), p. 33.