Are Method Requirements Reasonable?

I recently received an inquiry from a reader complaining about the difficulty he was having meeting system suitability for a liquid chromatography (LC) method that had been transferred into his laboratory. The system suitability test called for a column plate number, N, of ≥{8000 and a tailing factor, TF, of {≤1.5. The column was a 150 mm × 4.6 mm reversed-phase column packed with 5 µm diameter particles. The mobile phase was acetonitrile–buffer, with a buffer of triethylamine adjusted to pH 5.3 with dilute phosphoric acid. He could meet system suitability with a new column, but after fewer than 50 injections, the method would no longer pass. His inquiry had to do with what he could do to improve things without changing the method so that it needed to be revalidated.

As I look at the system suitability requirements, I'm not surprised that the method fails. Neither of the required performance criteria seem to be too demanding on the surface, but let's pull back and examine the potential for problems with this method. To do this, we have to look at what is reasonable and ideal performance for a column in terms of plate number and then how the tailing factor plays into this. The bottom line is that I think the only way out of his predicament is to live with short column life or rework the method.

Column Performance

Most of us are familiar with the column plate number, or column efficiency, which we abbreviate as N. The traditional way to measure N is by drawing tangents to the sides of the peak and measuring the peak width at the baseline between where the tangents intersect, as indicated in Figure 1. Then N is calculated based on this width, w_bl, and the retention time, t_R, as:

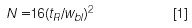
Another way to calculate N is based on the half-height method:

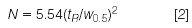
where w_0.5 is the width at the half-height. The half-height method is more convenient, because tangents don't need to be drawn. The value of N obtained at the baseline and half-height should be about the same, because the tangents usually coincide with the curve at the half height.

Sometimes you will see N converted into the plate height H, which can also be referred to as the height-equivalent of a theoretical plate, HETP. This is done by dividing N into the column length:

Although H can be useful when comparing the performance of columns of different lengths, it does not help much when comparing columns of different particle size. One technique that is used to compare column performance for columns of different particle sizes and lengths is to use the reduced plate height, h. This is calculated by dividing the plate height by the particle diameter, d_p:

The reduced plate height also gives us an idea of how well a column is performing relative to the ideal or theoretical performance. A very well-packed column operated under ideal conditions with well-behaved analytes, such as toluene or naphthalene, will give h ≈ 2. More realistically, real LC methods with real compounds will generate reduced plate heights of 3 or more.

So how well would the 150 mm × 4.6 mm, 5 µm column do under ideal and realistic conditions? After rearranging equation 4, we can see that h = 2 gives H = 0.010 mm or h = 3 gives H = 0.015 mm. We can rearrange equation 3 to calculate that N = 150 mm/0.010 mm = 15000 for the h = 2 case and N = 10000 for the h = 3 case. (Be sure to keep careful track of the units when you make these calculations: micrometres, millimetres and centimetres seem to get mixed up if you aren't careful!) Similarly, we can calculate that the value of N = 8000 for the system suitability test corresponds to h = 3.75, which should not be too hard to obtain with most compounds if we are careful. So far so good.

Tailing Factor

In the pharmaceutical industry, peak asymmetry is calculated as the tailing factor, TF (also called the USP or EP tailing factor based on instructions in the United States Pharmacopoeia or European Pharmacopoeia) as shown in Figure 2, where the half-widths of the peak are measured at 5% of the peak height:

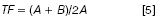
where A is the front half-width and B is the back half-width. If we rearrange equation 5, we can calculate that B = 2A in the limiting case, where the system suitability limit is TF = 1.5. That is, the back half-width is twice as wide as the front-half-width with a tailing factor of 1.5. For the rest of the discussion, we'll assume that A is unchanged from the perfectly symmetrical peak and all the tailing comes as a result of an increase in B. This is unlikely to be completely true, but it simplifies the discussion.

The Influence of TF on N

If we want to see how the tailing factor influences the plate number, things get a little more complicated. Although we could deal with this based upon some involved calculations, perhaps the simplest way to see what is happening is to look at some typical examples. For a symmetrical peak, we assume that the shape is a Gaussian curve, but this rarely occurs in real chromatography — the peaks almost always tail a little. Peak tailing can originate from several different causes, and different causes can result in different looking tails. However, the most common way to model a tailing peak is to use an exponentially modified Gaussian curve. There is a good description of this in reference 1, and a bonus is that this book comes with a CD containing an Excel spreadsheet designed to calculate LC separations with different amounts of tailing and overlap.

In Figure 3, I've shown three examples of tailing peaks using the method of reference 1, where TF = 1.24 [Figure 3(a)], 1.42 [Figure 3(b)] and 1.58 [Figure 3(c)]. I have also included an overlay of all three peaks [Figure 3(d)], because visual comparisons sometimes are difficult for stand-alone peaks. In Figures 3(a–c), I've drawn tangents to the peak to illustrate the intersection of the tangents with the chromatogram at the half-height. Thus, the plate number calculated from equation 1 or 2 should be equivalent; I'll use the half-height method here, because it is more convenient. You'll also notice that the tailing dramatically increases near the baseline, as is observed in real chromatograms.

To compare the influence of the plate number, we need to calculate N with and without the presence of tailing. If we assume that the tailing is all in the back half of the peak, we can double the front-half-width and calculate N based upon this for the non-tailing case (w = 2A) and compare it to the full peak-width method (w = A + B). In Table 1, I've summarized the results of my calculations based on measurements of the peaks in Figure 3. The plate number based on the nontailing peak is the same in each case, N ≈ 8850, as expected. For the TF = 1.24 peak of Figure 3(a), I can't measure any difference in the front and back half-widths measured at the half-height, although this is likely a limitation of my measurement technique. In any event, it looks like a tailing factor of less than approximately 1.25 will have little influence on the plate number. But as the peak tailing increases to 1.42 or larger, we can no longer pass the plate number requirement.

The examples of Figure 3 illustrate the interaction of the plate number and tailing factor, but are by no means absolute limits. They do, however, show how you can get into trouble if the system suitability requirements for a method are not well thought out.

Another Problem

There is another, perhaps more serious problem with the method that also deserves comment and that is the pH of the mobile phase. The buffer comprises triethylamine and phosphoric acid. Recall from your first year chemistry class that buffers are effective ±1 pH unit from their pK_a values. Triethylamine has pK_a = 11, and phosphate has three pK_a values: 2.1, 7.2 and 12.3. Note that the pH of the mobile phase (5.3) is well away from the buffering range of either of the buffer components. In other words, the "buffer" really isn't acting as a buffer. If buffering is needed to control ionization of the analyte and as a result, likely needed to help to control peak tailing, I would expect to have problems with the current mobile phase conditions.

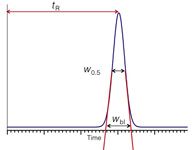
Figure 1: Measurement of column plate number at the peak baseline and half-height (equations 1 and 2).

Another red flag for me in the method is the presence of triethylamine at all. Triethylamine was a common additive to reduce peak tailing with the older, low-purity, type-A silica columns, but is rarely used with today's higherpurity, type-B silica columns. The column mentioned by the reader was a type-B column, so I suspect that the triethylamine is not needed. This also suggests that either the triethylamine as added for good measure or because "we've always done it that way." Or perhaps the method has migrated over the years from an older column to a newer one. Although the triethylamine is not likely to cause problems, it also is not likely to be needed. And I'm a strong adherent to the KISS (Keep It Simple, Stupid) principle when it comes to mobile phases — the more stuff you put in a mobile phase, the more likely you'll have problems.

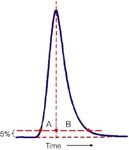
Figure 2: Calculation of peak tailing factor at 5% of peak height (equation 5).

So you can see that there are some potential problems with the mobile phase. I would be interested to see how the method performed with a real buffer in the system. One option would be to reduce the pH to the 2 < pH < 3 region, where phosphate buffers. Another option would be to use acetate (pK_a = 4.8) as the buffer, because it would provide true buffering at pH 5.3.

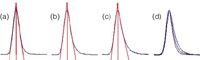
Figure 3: Peak tailing model with exponentially modified Gaussian peaks. Tailing factor (TF): (a) 1.24, (b) 1.42, (c) 1.58, and (d) overlay of a, b and c.

Conclusion

While the preceding discussion might be more than you want to know about the relationship between peak tailing and plate number, I hope the illustration gives you some ideas about how to examine data to see if the requirements of the method are realistic or not. In this case, a system suitability requirement of N { 8000 and TF { 1.5 each seemed reasonable, but in combination, the requirements may be a bit too stringent for a routine method with this column.

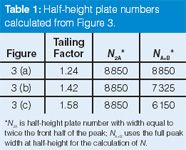
Table 1: Half-height plate numbers calculated from Figure 3.

Similarly, it is important to critically examine the method conditions to see if they make sense from a chemical and chromatographic point of view. In the present case, the pH of the mobile phase is outside of the buffering region of either of the buffer components, so there is little if any buffering going on. Because peak tailing is a significant problem with this method, adequate buffering is likely to be an important component of the method.

Finally, there is a bit of a conundrum about how to proceed with this method. It appears that the method works with a new column, but it fails system suitability much earlier than one would expect with a robust method. Any changes to the method would have to be validated, creating more work than would be required to transfer a previously validated method. So one has to balance the cost of method modification and revalidation against the cost of method transfer, early failure of the column and possibly required sample analysis repeats because of failure of system suitability during a run. If the method has a long projected lifetime, it is likely to be best to fix the problems and revalidate.

"LC Troubleshooting" editor John W. Dolan is vice president of LC Resources, Walnut Creek, California, USA; and a member of LCGC Europe's editorial advisory board. Direct correspondence about this column to "LC Troubleshooting", LCGC Europe, Poplar House, Park West, Sealand Road, Chester, CH1 4RN, UK, or e-mail Alasdair Matheson at amatheson@advanstar.com

References

1. U.D. Neue in Quantification in LC and GC, H.J. Kuss and S. Kromidas, Eds. (Wiley-VCH, 2009), Ch. 4.