Comparison Techniques For HPLC Column Performance

In the development of a chromatographic method, chromatographers often are required to determine the best column and operating conditions to address the problem at hand. With so many columns and operating conditions to choose from, it is sometimes a challenging task to choose the optimum column parameters (for example, particle size, column length, and column internal diameter) and optimum operating conditions (for example, mobile phase components, buffers, flow rates, and temperature). Often, a given is the high performance liquid chromatography (HPLC) instrumentation at hand, with its maximum pump pressure and flow rate capability and a column that can be installed in the instrument or in the drawer.

Ronald E. Majors

For most chromatographers, analyzing HPLC column performance consists of measuring the following four key performance parameters: the column efficiency (H or HETP) (as a function of the mobile phase flow rate), the column permeability, K_v0 (or pressure drop), the retention capacity (k value), and the column selectivity (α) for important pairs of analytes. Whereas the former two essentially are reflecting the packing quality and the mass transfer kinetics of the column, the latter two depend upon the dimensions and the chemistry of the stationary phase (1). Although the overall column quality also is determined by other factors, such as lifetime, chemical inertness, and mass loadability, the present contribution will focus on the first four parameters (H, K_v0, k, and α) because these are of a more general nature than the latter three, which depend strongly upon the chemistry and the economics of the application.

Effective Performance (Absolute Plots)

Traditional column comparison techniques: In a typical performance report or research paper, the main column performance parameters (H, K _v0 , k, and α) often are treated and discussed separately. Figure 1 shows such an example. The column efficiency usually is represented by the column Van Deemter curve (Figure 1a). To investigate the pure column performance, the reported plate height values preferably should be corrected for the extracolumn band broadening because these features only detract from a fair column comparison but are particularly important for the smaller particle columns that are being used today. The column permeability usually is assessed from a plot of the column pressure P (preferably also corrected for the extracolumn pressure drop) versus the flow rate or the interstitial-, the superficial-, or the t₀-marker velocity (Figure 1b). The retention capacity usually is represented as a plot of ln k' versus the fraction of organic modifier (φ) for one or more given test components (Figure 1c), while the information regarding the column selectivity usually is contained in a table giving the retention factor ratios of close-eluted pairs of components (Figure 1d).

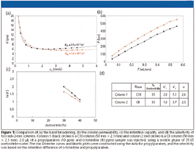
Figure 1

The example data shown in Figure 1 were obtained by comparing two particle types (similar sub-2-μm size, but bonded with different stationary phases, C8 and C18). Each particle type was evaluated by testing two purchased columns.

Most of the main performance parameters considered in Figure 1 are subjected to a tradeoff. Column efficiency and column permeability make a good example. Their tradeoff is best imagined by comparing an open-porous monolithic column (lower flow resistance but higher plate heights) with a sub-2-μm particle packed column (higher flow resistance but lower plate heights). Another example of this tradeoff is the choice between the two particle types considered in Figure 1. Figure 1a shows that the C18 column has a lower plate height in the C-term region of higher velocity (red data points), while Figure 1b shows that this same column has the disadvantage of having a substantially lower permeability (higher pressure drop). Which one of the two support types provides the best tradeoff between permeability and plate heights is difficult to tell, especially not if considering that the answer depends upon the plate number needed for the application (vide infra, Figure 3b). Neither the van Deemter curve in Figure 1a or the pressure–flow rate curve (Figure 1b) tells you which column would be the best choice for a particular separation.

There also can be a tradeoff between permeability and retention capacity. This again can be best illustrated by comparing reversed-phase monolithic and packed columns. An open-porous monolithic column certainly will offer a higher permeability, but might, because of its lower phase ratio, lead to a reduced retention capacity. Thus, to increase the retention capacity, it might be necessary to use a more viscous mobile phase (that is, with a higher aqueous fraction). Another example is the temperature tradeoff between the higher retention capacity but also higher C-term band broadening of an ambient temperature operation versus the lower C-term band broadening but also lower retention capacity of a high-temperature operation.

Without guidelines on how these tradeoffs should be made, column performance comparisons seldom can go further than just making a qualitative statement of the advantages and the disadvantages of the different systems being compared. The approach that usually is adopted to go beyond this qualitative comparison is to compare the efficiency, resolution, and analysis time of two representative chromatograms, one obtained with a reference system (standard column and standard operating conditions) and one with the new system (new column or new operating conditions). These chromatograms then are considered as the ultimate proof of the supposed superiority of this new system. However, the reference system is in many cases used under nonoptimized conditions so that the claimed gain in separation performance usually refers to a specific set of conditions. This approach sometimes is used by manufacturers to show that their column is superior to another column and is not a fair comparison.

The kinetic (absolute kinetic plots): A straightforward, practical, and economical tradeoff criteria very relevant to today's column technology, in which the individual importance of the four major column performance parameters: is properly weighted is the minimal analysis time needed to obtain a given efficiency, resolution, or peak capacity. As has been demonstrated by Desmet and colleagues (2), the calculation of this minimal analysis time can be done readily, without the need for any computerized optimization algorithm, by simply rearranging the data in a measured Van Deemter curve and the value of the column permeability (K _v0 ). This approach is the essence of the kinetic plot method of comparison.

Figure 2

The practical implementation of this rearrangement is illustrated in the spreadsheet table in Figure 2 and is based upon the two following simple equations, translating each data couple in a series of (u₀, H) data into a new series of t₀ versus N:

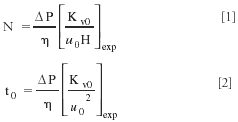
The quantities between brackets are obtained experimentally. The u₀- and H-values vary from row to row in the table in Figure 2, while the viscosity value (η), the available column inlet pressure (ΔP), and the column permeability normally remain constant throughout the table. While the column permeability (K _v0 ) is fixed experimentally, the values of ΔP and η can be chosen more freely, but it should be obvious that the most practically relevant system comparison is that for which each system is represented by its proper mobile phase viscosity and its proper available maximal pressure drop.

Figure 3 shows the graphical representation of the data rearrangement determined equations (1) and (2). Each (u₀,H) data point coming from Figure 3a (same data as in Figure 1a) is transformed into a unique (t₀,N) data point in Figure 3b. The outcome of the optimal tradeoff between permeability and plate height now can be seen at glance, for each possible required plate number. The support type with the lowest C-term band broadening (red data) can only be expected to provide superior separation speeds for separations having a t₀ of less than 0.5 min (total analysis time around 5–8 min), while the black data support should be preferred for all applications requiring a higher separation efficiency. Of course, this result assumes that the packing material (support) can be packed as well in a column that is shorter or longer than the tested column, but this assumption also is true for any column comparison discussion based upon a Van Deemter curve and a bed permeability.

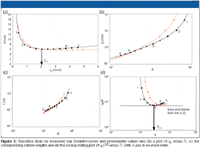
Figure 3

To really grasp the transformation between the Van Deemter curve and the kinetic plot in Figure 3b, it is important to realize that each data point of the kinetic plot relates to a separation performed at the maximal pressure (equations 1 and 2 are used with a fixed, maximal ΔP). Because each data point of the Van Deemter curve relates to a different velocity, it is therefore transformed into a separation performed in a column with a different length. Hence, whereas in a Van Deemter curve the pressure varies from point to point and the column length is constant, the opposite is true for a kinetic plot curve: the pressure remains constant, while each data point refers to a different column length. Because low velocities allow for the use of long columns, it should be obvious that the data points coming from the B-term dominated region of the Van Deemter curve transform into the long analysis time and high-resolution end of the kinetic plot (at which long columns are needed), while the data points originating from the C-term dominated region transform into short analysis time and low resolution data points (left hand side of plot). In Figure 3, this transition can be visualized easily by following the position of the numerated data points.

Because each row in Figure 1 relates to a given N value, it is straightforward to combine this value with the corresponding plate height value to obtain the corresponding column length. The column lengths thus obtained are plotted in Figure 3c, providing at a glance all optimal column lengths needed to achieve any of the possible required plate numbers. Although the kinetic plot in Figure 3b already comprises the complete performance potential of the two tested supports, another useful data representation format is one in which the y-axis values (t₀ ) are divided by the square of the corresponding N-value. Plotting the t₀ /N²-values obtained in this manner as a function of N (Figure 3d) offers two major advantages. First, the y-axis is compressed allowing for a better resolution between the different curves, and secondly, the curves now go through a minimum at an N-value that corresponds to the velocity at which the Van Deemter curve goes through its minimum (see the position of point 3). This is the point (marked by the optimal plate number value N_opt) at which the support is used under its kinetically most advantageous conditions, that is, in a column that is exactly long enough to allow the optimal mobile phase velocity to be established if the column is operated at maximal pressure. In other words, this is the point at which the support reaches its so-called Knox and Saleem (3) limit line (1). The advantage of the t₀ /N² versus N plot is that it automatically reveals the N-value for which this limit is reached (Figure 3d). If N_opt is larger than the actually required plate number, one should try to switch to structures with a smaller feature size (but with the same packing quality), whereas the opposite holds if N_opt is smaller than the required plate number.

Plots of time versus plate number for the comparison of different chromatographic support morphologies or different physicochemical operating conditions have been used since the early days of chromatography. In 1965, Giddings already presented such a plot to compare the performance limits of LC with those of gas chromatography (GC) (4) on a system-independent basis. Later Knox (3) and Guiochon (5) used the same approach to compare the performance of packed bed columns with open-tubular columns. In 1997, Hans Poppe (6) proposed to plot t₀ /N versus N instead of t ₀ versus N. Halasz and colleagues (7) also devised a method to compare columns directly with a different support morphology. This approach uses an equivalent sphere particle diameter based upon the measured column permeability. The plots obtained in this manner, however, have no direct relation to the kinetic performance of the tested columns and attribute an advantage that is too large to very open-porous systems such as monolithic columns.

The kinetic plot method provides an addition to the work of Giddings, Knox, and Poppe in the sense that the optimal kinetic performance curves presented by these authors were obtained through modeling and computer optimization, whereas the use of equations 1 and 2 now allows every analyst to establish a kinetic plot for his or her own tested column application and for his or her own specific application.

Once all t₀ - and N-values in the spreadsheet table (Figure 2) are known, it is quite straightforward to use the values of the phase retention factor k' and a measured selectivity factor to calculate the corresponding total residence time [t_R = t₀ (1 + k')], the effective plate number [N_eff = Nk'² /(1 + k')² ], the isocratic peak capacity, and the resolution R_s. As is illustrated in the schematic representation in Figure 4, plots involving these quantities can be used to extend the tradeoff between column efficiency and permeability by also including the retention capacity (plots involving t_R and N_eff) and even the column selectivity (plots involving R_s).

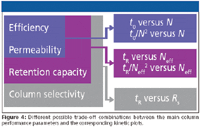
Figure 4

Figure 5 gives an example of the latter. Because the C18-bonded particles provide a higher selectivity for the analytes propylparaben and o-toluidine than the C8-bonded particles (see Figure 1d), it is not unexpected to find that the range wherein the C18-bonded particles are beneficial is in the resolution kinetic plot even broader than in Figures 3b and 3d, where only the separation kinetics were taken into account and not the selectivity of the supports. The type of kinetic plot shown in Figure 5, then, provides a more complete image of the real chromatographic quality of a tested support. The downside of this "completeness" is that the inclusion of the selectivity parameter makes the plot less general and much more application specific, but this might in many cases just be an advantage.

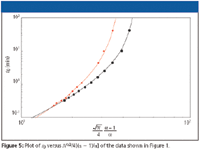
Figure 5

Intrinsic Performance (Reduced Plots)

Reduced plate height plot:Apart from the absolute column performance, it is also customary to look at the dimensionless performance of the system. This dimensionless performance usually is made to assess the intrinsic quality of a column, that is, to find out how far the column packing is from the best possible packing (Knox's theoretical "yardstick" [8]) and whether more effort should be spent to improve the packing homogeneity or not. The use of dimensionless performance parameters dates back from the work of Giddings (9) and allows one to filter out the effect of the particle (or skeleton) size on the band broadening and the column permeability.

Although this approach is indispensable in any thorough column study (it offers an in-depth understanding of the different parameters that contribute to the band broadening and provides a universal measure for the flow resistance), one should be cautious of the fact that the obtained A-, B-, and C-term constants depend directly upon the value of the employed mean particle size. The latter parameter is not as straightforward as it appears to be, because, apart from the measurement problems (different particle size measurements such as Coulter counter, laser diffraction, and scanning electron microscopy (SEM)-picture analysis tend to give different values), there are also no good rules for how the average size should be defined in the case of particles with a broad size distribution. Good suggestions have been made (10), but these are not supported by any theory. An even larger problem is finding a "fair" (or better, theoretically sound) characteristic size that can be used to assess the packing uniformity of columns with a different support shape, such as the monolithic columns. A similar particle size averaging and definition problem arises when trying to calculate the dimensionless version of the column permeability, that is, the flow resistance. Although here the theory of chemical engineering is very clear in showing that the Sauter mean diameter should be used (11), many cited flow resistance values in the literature still are based upon the number- or the volume-averaged particle size. In addition, flow resistance values also can depend heavily upon the intraparticle porosity. Without going into detail, it can be shown (11) that if the flow resistance is based upon the t ₀ -marker velocity (the most frequently employed velocity in the field of HPLC), its value will not only depend upon the external porosity (that is, the packing density), but also on the intraparticle porosity. The latter is a bit unfortunate, because, on a practical basis, one usually desires to use the flow resistance as a measure for the packing density and quality. The contribution of the intra-particle porosity tends to bias this measure. Superficial velocity-based and interstitial velocity-based flow resistances do not suffer from this bias (11).

Reduced kinetic plots: Absolute kinetic plots such as the ones shown in Figure 3 and Figure 5 depend upon the size of the support, the applied pressure, and the viscosity and diffusivity of the mobile phase used. To obtain a "pure" measure of the kinetic performance, that is, a measure solely reflecting the kinetic quality of the packing and the support (both external shape and internal pore network), a size-independent plot is needed.

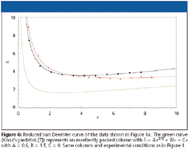
Figure 6

As shown by Billen and colleagues (12), such a size-independent kinetic plot can be obtained by plotting the separation impedance E (13) (originally established by Golay) versus the ratio of N/N_opt. As shown in equation 3 and Figure 2, it is straightforward to calculate the separation impedance for each data point of the measured Van Deemter curve:

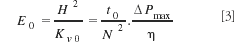
Equation 3 shows that the vertical difference between two E-curves is still equal to the difference in required analysis time. Because N _opt can be assessed directly from a plot of t ₀ /N² versus N, it is also quite straightforward to calculate the value of N/N _opt for each velocity entry in the spreadsheet table. Figure 7 shows the corresponding reduced kinetic plot curves for the two presently considered columns.

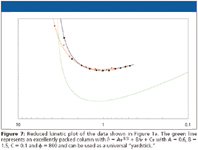
Figure 10

Without going into detail, it can be shown (12) that a plot of E ₀ versus N _opt /N depends only on the dimensionless variables h, n, and f. Hence, a reduced kinetic plot can be used for similar purposes as a reduced Van Deemter plot. Packings with the same packing quality and the same retention factor but a different size will yield coinciding curves. The lower this curve, the better packed is the column. The reduced kinetic plot, however, has two important advantages over the reduced Van Deemter plot. The first one is that it also incorporates the column permeability information and, hence, reflects the quality of the tradeoff that is made between column permeability and column efficiency. The second one is that it can be established without having to define or measure a reference diameter, or without having to know the mobile phase diffusivity, thereby providing a way to circumvent the problems mentioned previously.

Turning now to the two supports considered in the previous figures, the reduced kinetic plot in Figure 7 shows that the slightly larger permeability of the C18 column apparently compensates for its slightly steeper C-term and higher H_min, as can be witnessed from the fact that both supports have a nearly perfectly overlapping reduced kinetic plot curve. This overlap allows to conclude that both considered systems display the same intrinsic kinetic quality. Hence, the earlier question that was posed when considering the original plate height and permeability data in Figure 1a (that is, which support performs best or is to be preferred?) can now be answered unambiguously as follows: both support types have the same intrinsic kinetic quality, but they reach their optimal kinetic performance at different values of required N (see Figure 3d). However, given the fact that both curves are relatively remote from the best possible packed column line (the green curve in Figure 7), it also should be concluded that, although the two supports perform equally well, they perform relatively poorly.

Use of the Kinetic Plot Method to Compare Some State-of-the-Art HPLC Supports and Methods

The data shown in Figure 8 have been obtained on several commercial packed columns filled with particles with different size (each curve is the average of two tested columns). The data are represented as a plot of the total analysis time (t_R) versus the isocratic peak capacity. The columns also were tested at two different temperatures (30 °C and 80 °C). During these experiments, it was observed that the two tested 3.5-μm columns performed, in relative terms, not as well at 80 °C (most probably by coincidence) than the sub-2-μm particles and the 5-μm particles. This behavior can be observed by comparing the data in Figures 8a and 8b, showing that the 3.5-μm particles are to be preferred over a much broader range of N-values at 30 °C than at 80 °C.

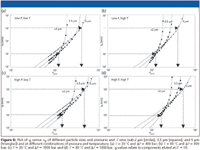
Figure 11

The following main conclusions can be drawn from the kinetic plot curves shown in Figure 8. Provided every support is used in a column with an adapted length, the advantage of an increased temperature is relatively small (compare Figures 8a and 8b). This conclusion might very well be biased by the unexpectedly poor performance of the 3.5-μm particles at the elevated temperature, but for the columns studied presently, the conclusion clearly holds. Larger gains in peak capacity can be obtained by switching to higher pressures (compare Figures 8a and 8c), although the gain is certainly not dramatic, and perhaps not worth the additional investment. Comparing Figures 8a and 8c with Figures 8b and 8d also shows that the advantage of small particles diminishes if columns are run at elevated temperatures. Another conclusion that can be drawn from Figure 8 is that sub-2-μm particles only have an advantage for very short separations, lasting less than 10 s (40 s if 1000 bar pressure is available).

All these conclusions can be made at glance, while they would have been nearly impossible to make if the same data would have been plotted in the Van Deemter curve format.

How to Go About Establishing a Reliable Kinetic Plot — Some Practical Considerations

Equations 1 and 2 are based upon the t₀ -marker velocity (u₀), because analysts are always most interested in a prediction of the total analysis time, which is most conveniently determined as the product of the t₀ -time and the (1 + k')-factor. Given that significant differences between the elution time of different t₀ -markers can be observed, it is preferable for one should to always test different t₀ -markers to find out which gives the most reliable value. Once a good t₀ -marker is found, it is also important to correct the obtained t₀ -value for the extra-column volume. Traditionally, this is done by removing the column and replacing it by a zero-dead-volume connection piece to obtain the system dead-time t_sys, which then can be used to calculate the t₀-time of the column only. The observed variances also should be corrected for the extracolumn band broadening, for a kinetic plot can be established correctly only if the plate height values are either corrected for the extracolumn band broadening or were not influenced by it. The extracolumn band broadening correction can be carried out using the following expression:

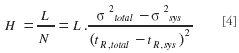
To be consistent, all parameters in equations 1 and 2 should be defined in the same unit system.

Ideally, the variances should be calculated using the method of moments, for this method automatically and properly accounts for any asymmetry in the peaks. Hence, using the method of moments, columns leading to strongly tailing peaks can be penalized properly for this. Determining the plate heights using the peak width at half height, however, is easier and less prone to measurement errors, but might in the case of asymmetrical peaks lead to significant errors.

It also goes without saying that the data used to establish the kinetic plot should be obtained with a component that is relevant for the application one has in mind. If desired, kinetic plot curves obtained for a mixture of different components can be combined in one single average curve (14). It also is possible to incorporate the limitations (such as flow rate limitation, detector sampling rate limitation, and so forth) of the instrument directly (15) in the kinetic plot curves, but in this case, the mathematics are more complex.

Acknowledgment

Deirdre Cabooter is thanked for her critical help in the preparation of the manuscript.

Ronald E. Majors

"Column Watch" Editor Ronald E. Majors is business development manager, Consumables and Accessories Business Unit, Agilent Technologies, Wilmington, Delaware, and is a member of LCGC's editorial advisory board. Direct correspondence about this column to "Column Watch,"LCGC, Woodbridge Corporate Plaza, 485 Route 1 South, Building F, First Floor, Iselin, NJ 08830, e-mail lcgcedit@lcgcmag.com

References

(1) G. Rozing, LCGC 26(S4) (2008).

(2) G. Desmet, D. Clicq, and P. Gzil, Anal. Chem. 77, 4058–4070 (2005).

(3) J.H. Knox and M. Saleem, J. Chromatogr. Sci. 7, 614–622 (1969).

(4) J.C. Giddings, Anal. Chem. 37, 60–63 (1965).

(5) G. Guiochon, Anal. Chem. 53, 1318–1325 (1981).

(6) H. Poppe, J. Chromatogr., A 778, 3–21 (1997).

(7) I. Halász, R. Endele, and J. Asshauer, J. Chromatogr. 112, 37–60 (1975).

(8) J.H. Knox, Ann. Rev. Phys. Chem. 24, 29–49 (1973).

(9) J.C. Giddings, in Dynamics of Chromatography — Part I (Marcel Dekker, New York, 1965), pp. 56–60.

(10) U.D. Neue, in HPLC Columns: Theory, Technology, and Practice (John Wiley & Sons, New York, 1997), pp. 81–86.

(11) D. Cabooter, J. Billen, H. Terryn, F. Lynen, P. Sandra, and G. Desmet, J. Chromatogr. A 1178, 108–117 (2008).

(12) J. Billen, D. Guillarme, S. Rudaz, J.-L. Veuthey, H. Ritchie, B. Grady, and G. Desmet, J. Chromatogr., A 1161, 224–233 (2007).

(13) P.A. Bristow and J.H. Knox, Chromatographia 10, 279–289 (1977).

(14) D. Cabooter, A. De Villiers, D. Clicq, R. Szucs, P. Sandra, and G. Desmet, J. Chromatogr., A 1147, 183–191 (2007).

(15) G. Desmet, P. Gzil, D.T.-T Nguyen, D. Guillarme, S. Rudaz, J.-L. Veuthey, N. Vervoort, G. Torok, D. Cabooter, and D. Clicq, Anal. Chem. 78, 2150–2162 (2006).