Understanding the Science Behind Packing High-Efficiency Columns and Capillaries: Facts, Fundamentals, Challenges, and Future Directions

Despite the theoretical promise of reduced plate heights (h) less than 1, most modern ultrahigh-pressure columns can only deliver plate heights in the range from 1.4 to 2.5 when packed with small particles. The improved design of current packing procedures, a better understanding of the colloidal properties of particle suspensions, and the study of the relationship between the rheological behavior of packed beds and the final bed structure should lead us to the practical solutions that could double the column efficiencies to overcome the current resolution limits. This installment of "Column Watch" describes the important practical features of packing procedures, their link to the observed bed structure and column performance, and the challenges that are still faced for the packing of highly efficient analytical and capillary columns with fully porous particles (FPPs) and superficially porous particles (SPPs). This work demonstrates that the next generation of packing methodologies to be developed toward the ultimate performance of the infinite diameter column will eliminate the uneveness of the stress applied to the packed particles across the column diameter during bed consolidation.

Ultrahigh pressure liquid chromatography (UHPLC) relies on the packing of very small fully porous particles (FPPs), superficially porous particles (SPPs), or nonporous particles (NPPs) with various surface chemistries into confined tubes. One challenge in separation science is to produce mechanically stable beds through which the analyte will travel with a very narrow velocity distribution under pressure-driven flow. At best, randomly packed beds consisting of NPPs, SPPs, and FPPs can produce reduced plate heights h (equal to the theoretical plate height divided by the particle diameter, H/d_p) as low as 0.5, 0.7, and 0.9, respectively (1–4). For instance, the maximum plate counts of 5-cm-long columns packed with 2-µm particles should be in theory ~50,000, 36,000, and 28,000 with NPPs, SPPs, and FPPs, respectively. At this point, it is worthwhile to dispel the myth that h ≈ 2 is the practical limit. A reduced plate height of 1.0 has been achieved in analytical and capillary columns (5,6). In most cases for 5-cm columns, plate counts > 15,000 are quite rare irrespective of the particle nature, confirming that research is still needed toward a better understanding of column packing from both practical and fundamental viewpoints.

Packing modern analytical or capillary columns is nothing but a high-pressure filtration of a suspension (stationary phase) inside a cylindrical tube (column). In the slurry reservoir (Figure 1), the stability of the colloidal suspension plays a vital role (7). Before bed consolidation, the slurry suspension is in a state of motion and can be assimilated to a continuous fluid. Most stationary-phase suspensions are non-Newtonian-that is, their viscosity changes as a function of shear rate (depending on the pressure and flow applied) and particle concentration (7,8). The clustering or alignment of the particles in the flow field (shear thickening or thinning effects, respectively) determines the suspension viscosity and, ultimately, the pressure-stress distribution in the column volume. When the particles come in contact with surrounding ones, the bed begins to consolidate and their displacement becomes extremely limited: It is governed by the stress-strain rheological behavior of the compact packing. The interparticle shear forces, the friction between the particles and the column wall, and the axial and radial stress distributions eventually dictate the quality of the final packed bed. Particles are finally immobile and form a stable bed. The following sections discuss the most relevant experimental requirements for packing highly efficient columns, establish the relationship between bed structure and column performance, and lay out the future directions of this research field.

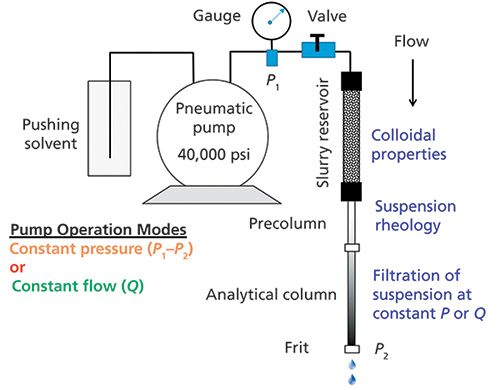
Figure 1: Schematic of a research-grade analytical packing system showing the major components required for high efficiency packing. Most slurry chambers are custom designed by certified high-pressure manufacturers.

Hardware Design Is Critical for Achieving High Efficiencies

Figure 1 shows the major elements for packing analytical columns of length L_bed (with internal diameters of, for example,
1 to 4.6 mm). A stationary-phase suspension is introduced into a slurry reservoir, the remaining volume is topped up with either the pushing solvent or the slurry solvent. Column packing begins when the slurry reservoir is pressurized in either constant-pressure (P) or constant flow rate (Q) mode. Pneumatic pumps (see Figure 2 for an actual picture) are designed to maintain the pressure constant during packing. Pressure per unit length decreases during packing as the bed is formed. Pneumatic pumps can be converted to constant-flow pumps by an electronic feedback system (9). The pressure required to keep the flow rate constant during packing varies as L_bed/(d_p²d_c²), where d_c is column internal diameter, and P per unit length is maintained constant. More axially uniform beds are expected in constant-flow than in constant-pressure packing mode. Haskel pumps conveniently generate up to 40,000 psi pressure differential between the slurry chamber inlet (P₁) and the column outlet (P₂) as shown in Figure 1. The precolumn internal diameter should match the column internal diameter to avoid sudden changes in the suspension velocity as it enters the column tube (Figure 1). The slurry chamber temperature can also be controlled with a water jacket to mitigate non-Newtonian viscosity effects (7).

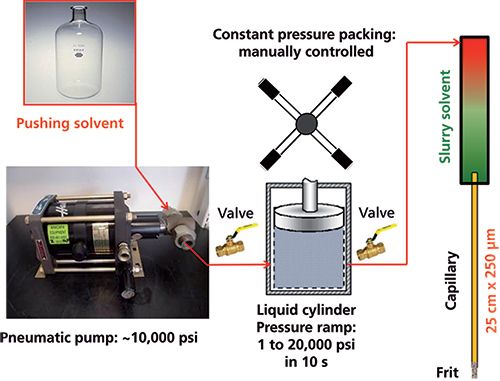
Figure 2: Schematic of a research-grade capillary packing system (see text for details).

Packing highly efficient capillaries is challenging because of their small internal diameter and very high pressure requirements from 20,000 to 60,000 psi for the preparation of 25-cm to 1-m-long capillaries. Figure 2 shows a research-grade capillary packing system: A slurry chamber is connected to a manual screw pump, which can generate up to 10,000–60,000 psi. The pump inlet is fed by a pneumatic pump. The "ship-wheel" is manually turned to create a pulse-free pressure ramp in the cylinder connected to a slurry chamber to pack the capillary. This design enables easy control of the pressure at the top of the bed during packing. The chamber design should prevent particle clogging at the inlet during packing, which can be achieved by inserting the capillary inlet slightly up above in the slurry chamber (downward packing), stirring the slurry suspension, or using an upward packing chamber (the latter approach is used by James Jorgenson's group) (8). The real pictures of packing systems are provided in a recent publication (8). Semirigid polymeric materials and wide-pore silica are generally packed at lower pressures depending on the manufacturer's instructions.

Colloidal Aspects Required for Making High Efficiency Columns

The critical properties of the suspension solvent include its wettability (surface tension), viscosity, density, and dispersing ability. A comprehensive set of slurry solvents and a flow chart for choosing an optimum slurry are available (8). Herein the term dispersion implies microscopically separated and independently moving particles in a given solvent system. The dispersion of the particle suspension dictates the success or failure of the packing process for both analytical and capillary columns. In dilute suspensions, the particles remain separated and move independently (~10–50 mg/10 mL). Even for concentrated suspensions, the particles are still independent, albeit their motion is restricted (8). Unlike common belief, the settling rate does not guarantee suspension dispersion since particles can be aggregated and yet settle very slowly because of high solvent viscosity whereas a comparison of settled bed height (in Wintrobe tubes) is often useful (7). For instance, sub-2-µm silica SPPs settle quickly in 85:15 (v/v) acetone–dichloromethane (10); however, microscopically they are well dispersed-~280,000 plates/m (h < 2) could be obtained in 1 cm × 0.30 cm columns. To generalize this concept, Figure 3 shows the chromatographic results after packing 1.9-µm FPPs in 5 cm × 0.3 cm columns (8). Using a low slurry concentration of a dispersed suspension often leads to fronting peaks, which could suggest an improperly chosen solvent. When a narrow fronting peak is observed, it is actually a good sign that the chosen slurry will produce high efficiency because only the slurry concentration needs to be increased. As the particle concentration is increased from 10% to 23% w/v, the same solvent mixture produces h ≈ 2.35. Analytical columns packed with aggregating solvents produce broad tailing peaks with polymers, chiral–achiral chemistries on silica and even bare silica FPP and SPP. Fronting peaks are never observed with an aggregating solvent system, and with packing pressures of 12,000 psi beds eventually settle, which creates a void at one column end (8). It is clear that beds formed from aggregated suspensions in analytical columns are difficult to compress at these pressures. Whether aggregating suspensions packed at extreme pressures (20,000–30,000 psi) produce acceptable analytical columns is still an open question. These concepts have been experimentally demonstrated on FPPs, SPPs, and NPPs of various materials (7,8).

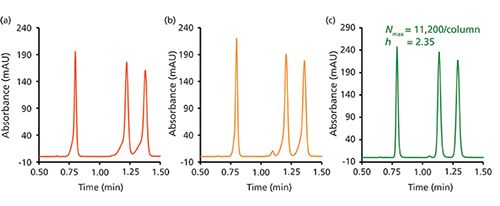
Figure 3: Effect of varying slurry concentrations on peak shape and efficiency of 5 cm × 0.3 cm columns packed with 1.9-µm FPP silica using a dispersed slurry of chloroform-2-propanol: (a) 3.5%, (b) 10%, and (c) 23% w/v slurry concentrations. Pressure gradients of 0–8000 psi followed by 8000–11,000 psi were applied for 15 min each. Sample: a mixture of uracil, adenine, and cytosine (in the order of elution). Note the half-height efficiencies of the last peak are almost similar. (Data from reference 8.)

Jorgenson's and Tallarek's groups have extensively studied the packing phenomenon in capillaries with FPPs and SPPs (6,11,12). It is striking that agglomerated suspensions produce remarkably high efficiencies in capillary formats. Combining an agglomerating solvent (acetone) with 80-kHz ultrasonication produced h ≈ 1 in 1 m × 75 µm capillaries packed with 1.9-µm BEH-C18 particles at a packing pressure of 30,000 psi (6). Optical microscopy (see Figure 4) showed that in fused-silica capillaries, agglomerates pile up like snowflakes and reduce particle size segregation and particle rearrangement during preconsolidation and bed consolidation, respectively. During preconsolidation of the bed, dilute suspensions allow the particles to roll off toward the walls from the capillary center and lead to lower capillary efficiencies (13). Figure 4 is a snapshot of a video showing a capillary being packed at 10- and 100-mg/mL slurry concentrations. Slurry aggregation observed for very large slurry concentrations (up to 200 g/L) is indeed beneficial-such suspensions prevent radial particle size segregation during preconsolidation and particle rearrangement during consolidation. However, this slurry aggregation may lead to an excessive number of large voids. Thus, an experimental compromise has to be found for an optimum slurry concentration, or sonication can be applied during packing. Whether agglomerated suspensions are universally beneficial for polymers, silica, or polar silica-bonded phases need further exploration.

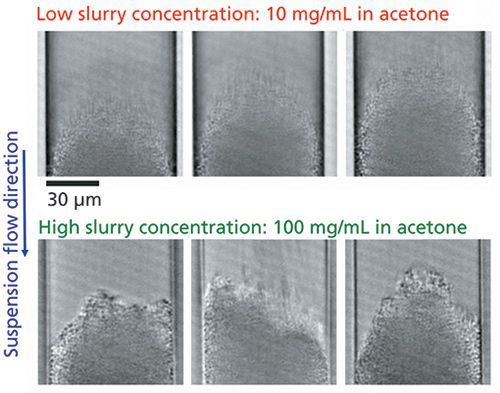
Figure 4: Snapshots of videos taken during the formation of the column beds in 100-µm i.d. capillaries for two slurry concentrations (10 and 100 mg/mL) of 1.9-µm BEH-C18 particles. The frames are random snapshots. At 10 mg/mL (top row), the bed is formed by a stream of individually arriving particles resulting in a parabolic shape of the bed front. At 100 mg/mL (bottom row), particles reach in large patches causing fluctuations in the form of the actual bed front. (Images obtained from A. Reising; joint work by Reising, Godinho, Jorgenson, and Tallarek groups. Adapted from reference 13.)

Slurry Temperature as an Underutilized Packing Variable

Temperature changes the non-Newtonian behavior of suspensions by delaying the onset of shear thickening and reducing suspension viscosity (7). The temperature parameter should be used with caution. At higher temperatures, silica-based phases can either agglomerate in organic solvents or be dispersed depending on the surface chemistry as seen with 3-µm C18 particles suspended in acetone–hexane (33–77%) (14). Capillaries packed with 3-µm C18 FPP at 70 °C under sonication yielded higher plate counts than those of capillaries packed at room temperature (14). Similar results are seen with high efficiency 4.4-µm NPP ion-exchange phases that actively show shear thickening effects in aqueous slurries (7).

Constant-Flow or Constant-Pressure Packing: Circumventing Axial Heterogeneity in the Column Bed

The column-bed density is often non-uniform along its length, which results in local plate-height variation along the column (15). Axial heterogeneities arise from the variations of the local pressure gradient per unit length as the bed height is increasing. In the constant-pressure mode of packing, the slurry chamber is suddenly pressurized, which initially creates a dense bed. As the bed height increases, both the pressure gradient and the bed density decrease. In contrast, in the constant-flow mode, the applied pressure gradient per unit length remains constant along the entire bed length, and the plate-height distribution is expected to be more uniform along the column than for the constant-pressure mode (16).

Flow-reversal experiments have revealed the axial heterogeneity of relatively long beds packed at constant pressure (see Figure 5) (17). An injected band is first detected before it enters the column and brought up to an arbitrary axial position (z), at which time the flow is suddenly reversed; the band is then re-detected after it just exits the column at the same end it entered from. For instance, a band brought up at z cm from the inlet will travel a total distance 2z cm. Figure 5a shows the variation of the net plate height with increasing z when packing a 15 cm × 0.3 cm column at constant pressure with 2.5-µm BEH C18 particles. The slurry chamber was pressurized to 15,000 psi quickly. The net plate height along the distance 2z strongly depends on the reversal position z, which reveals an axially heterogeneous bed. This result was also confirmed by testing three physical sections of a 15-cm-long column of SPPs, the inlet and bottom portions typically show the worst peak shape and efficiency (8,15). Figure 5b shows the same graph as in Figure 5a, except for constant-flow packing of the same column up to 15,000 psi. In constant-flow packing, the 15-cm-long column appears almost axially uniform, as the net plate height remains constant irrespective of the reversal position z. The hybrid approach of constant flow and pressure is to use a pseudoconstant-flow mode (8). In this method, the pressurization starts from 0 psi to the final desired pressure in 10–30 s. This process ensures that the bottom section of the column does not pack at extremely high velocities. One can obtain extremely high plates using this approach, such as h ≈ 1.8 (1.9-µm SPP) with stable beds.

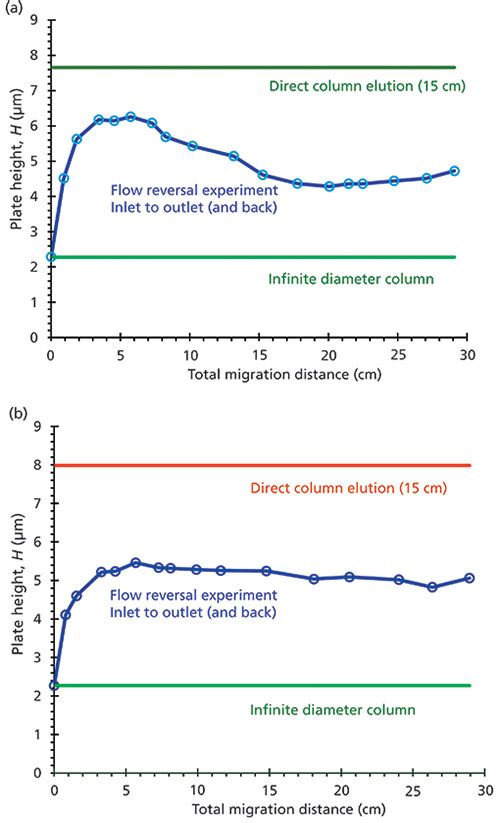
Figure 5: Flow reversal experiments showing the axial heterogeneity of a 15 cm × 0.3 cm column packed with 2.5-µm BEH-C18 particles. The sample is injected at the column inlet (z = 0) and reaches variable axial positions (0 < z < 15 cm) along the column when the flow is suddenly reversed. The total migration distance is then 2z. The corresponding net plate heights are represented by the connected blue empty circles. The red segment locates the usual column plate height measured at elution at the column outlet in absence of flow reversal. The green segment locates the plate height of the infinite diameter column (IDC). Uracil was used as a probe with 80:20 (v/v) acetonitrile–water mobile phase at room temperature. Flow rate: 0.5 mL/min; pressure drop: 3000 psi. (a) Constant-pressure packing at 15,000 psi. (b) Constant-flow rate packing up to a maximum pressure of 15,000 psi. In both cases, the packing methods were not optimized for maximum column efficiency.

Fundamental Problems Recognized Very Early

At pressures greater than 10,000 psi, the solvents and the stationary phase are compressed. The column tube also expands under severe stress in both axial and radial directions (18). Most importantly, the bed volume does not experience a uniform stress field (14). Rheological analysis has shown that the stress varies from the center to the wall region of the column. Indeed, the particles in the slurry do not move at the same rate across the entire column internal diameter during the preconsolidation step. In Figure 6, silica and alumina layers are alternately placed in a glass tube. In carbon tetrachloride, silica is transparent and alumina is not. When the piston compresses the suspension (upward motion), the strain and the stress are smaller and larger, respectively, in the wall region than in the center of the column (19). Therefore, after the bed is compressed, during bed consolidation, the particles are more densely packed in the wall region (where the stress is higher) than they are in the center (where the stress is smaller).

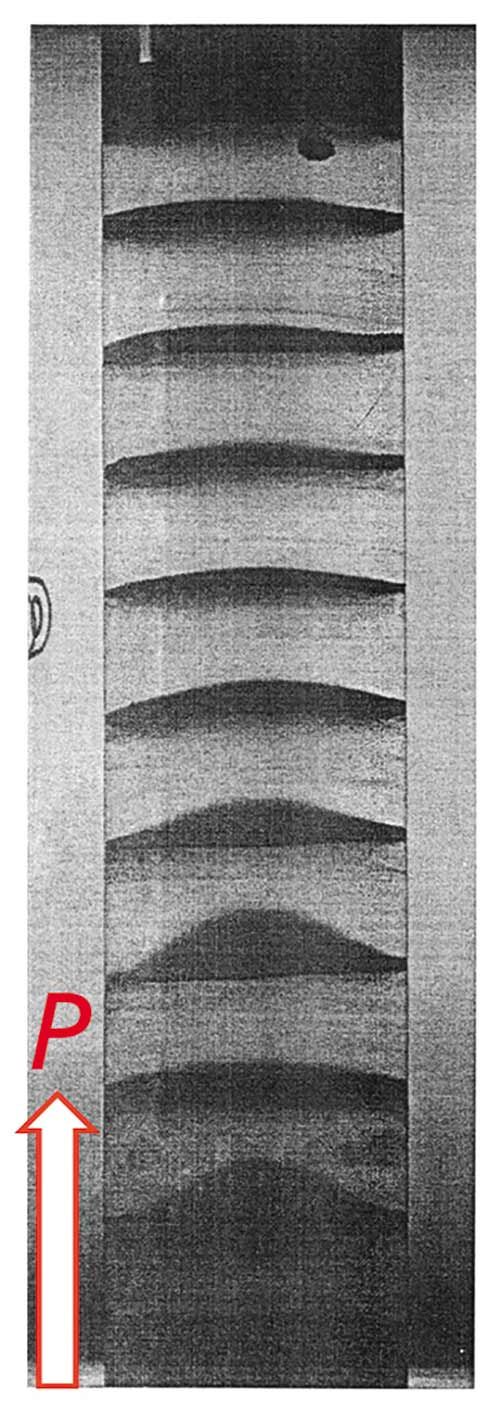
Figure 6: Stress and strain distribution in an axially compressed column (the piston moved upward from the bottom before bed consolidation). The axial stress applied was 5.0 MPa (725 psi). The layers of a C18 silica slurry ~1 cm thick were interspersed with thin marker layers of a carbon tetrachloride slurry of alumina particles. In carbon tetrachloride, only alumina layers are visible (dark color). The upward motion of the particles is slower at the wall than at the center because of wall effects and higher stress in the wall than in the center of the column. (Adapted with permission from reference 19.)

What Is Limiting the Efficiency of Modern Analytical Packed Columns?

The ultimate performance of a packed column is that of the infinite diameter column (IDC) free from the wall and border effects of dispersion. The expected minimum h (h_min) values of the IDC packed with NPPs, SPPs, and FPPs are 0.5, 0.7, and 0.9, respectively (1). In practice, the h_min values for 2.1–4.6 mm i.d. columns packed with SPPs and FPPs usually vary from 1.2 to 1.6 (20) and from 1.7 to 2.1 (21), respectively.

Characterization techniques such as focused-ion-beam scanning electron microscopy (FIB-SEM) (22) and microvoltamperometry (23) have revealed the actual structure of packed beds. It was confirmed that the performance of modern columns is inherently limited by the radial heterogeneity of the flow velocity across the column diameter. More accurately, FIB-SEM showed the coexistence of three concentric bed regions (see Figure 7): a thin, loose, and orderly packed layer against the column wall (1.5 d_p wide), an intermediate thick, dense, and randomly packed layer (70 d_p wide), and the randomly packed bulk region. The average velocity in the intermediate wall region is 5% smaller than the bulk velocity. The largest local velocity deficit is 25% of the bulk velocity. This center-to-wall velocity bias is entirely responsible for the gap observed between the performance of modern analytical columns and that of the IDC.

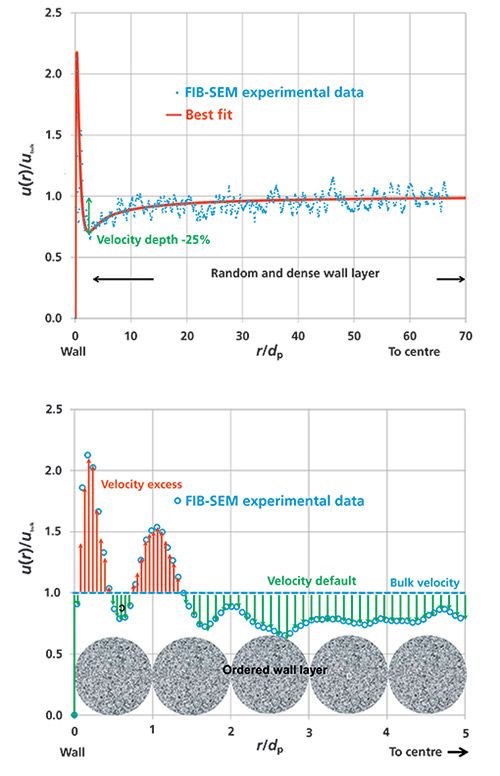
Figure 7: Top graph: Experimental interstitial linear velocity profile across the diameter of a 50 mm × 2.1 mm column packed with 2-µm particles. The x-axis represents distance in particle diameters unit from the column wall up to 70 particle diameters. Beyond, the velocity profile is flat and is equal to the bulk velocity. Note the existence of a thick (140-µm-wide), dense, and randomly packed layer in the peripheral region of the column. Bottom graph: Zoom in the left graph up to only 5 particle diameters from the impenetrable wall. Note the existence of a thin (2–3 particle diameter wide), loose, and orderly packed layer at the very wall of the column as well as the hydrodynamic boundary layer (0.15 particle diameter wide). The average relative velocity default (from the bulk velocity) is 5% more than these three wall regions. The maximum velocity default observed at 2.7 particle diameters from the wall is 25% the bulk velocity. (Adapted from the data in reference 26. FIB-SEM data are from A. Reising [Tallarek's group].)

The origin of the transverse flow unevenness inherently pertains to the packing method used to build the chromatographic beds. As the bed consolidates, the stress applied to the particles in the wall region is higher than that acting on the particles in the bulk region (15,24). Consequently, particles are more densely packed in the wall than in the bulk area causing undesirable radial velocity biases. As shown in Figure 7, the velocity deficit in the intermediate region more than compensates for the velocity excess at the very wall which limits the performance of actual columns relative to that of the IDC (25).

What Should We Do to Improve the Performance of Modern Analytical Packed Columns?

The key to improving the performance of modern chromatographic columns is to find the right combination of particle morphology, slurry solvent, slurry concentration, and packing pressure for which the velocity deficits eventually compensate for the velocity excesses. This was recently validated from a fundamental viewpoint (26). From the general dispersion theory of Aris (27) and the actual flow velocity profile shown in Figure 7, assuming that the velocity depth in the intermediate region would be reduced from 25% (experimentally observed, see Figure 7) to 20%, 15%, 10%, and 5%, then h_min would decrease from 1.70 to 1.30, 1.10, 0.95, and to the ultimate limit of 0.90 for the IDC. Figure 8 shows the evolution of the predicted plots of h versus the velocity as a function of the velocity depth.

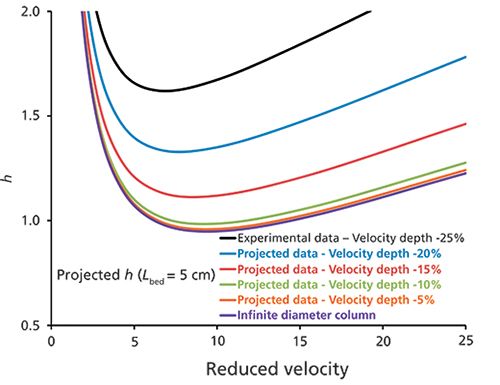
Figure 8: Calculated evolution of the reduced plate height plots as a function of the intensity of the maximum velocity default in the dense wall region shown in Figure 7. Note that the performance of the infinite diameter column would be reached within 5% if the depth of the velocity valley was reduced to only 5–10% of the bulk velocity. (Adapted from data in reference 26.)

In practice, how can we realize this velocity compensation? Two options can be anticipated:

1. Minimize the stress differential between the center and the wall of the column, which will generate uniform particle rearrangement (or strain) across the whole bed diameter during bed consolidation. This packing option has been used with partial success for sub-2-µm FPPs and sub-3-µm SPPs in 5 cm × 3 mm columns packed at pressures smaller than 10,000 psi and using relatively concentrated slurry concentrations (>15%, v/v) (8). Low packing pressure combined with high slurry concentration is undoubtedly the best option to pack analytical size columns uniformly, but their beds may end up loose and unstable, which compromises the column durability for a large number of injections at high pressure injections (>7000 psi). Eventually, FPPs can deliver an h_min value as small as 1.7 (21). This value remains far from the ultimate h_min of 0.9.

2. Despite the presence of severe stress differentials between the center and the wall of the column, the radial distribution of particle strain can be narrowed down by modifying the external morphology of the particles. Rough particles lead to increasing shear friction forces, which freezes their rearrangement during consolidation. This is typically the case for SPPs. Indeed, SEM photographs revealed a rougher external morphology for SPPs relative to that of FPPs (28) because the method used to synthethize SPPs is different from that used to prepare FPPs. There is indirect evidence that SPPs induce higher flow resistance because their Kozeny-Carman permeability constant is typically 200–250 (29), while that of FPPs is 140–180 (30). Also, their columns have higher external porosities (0.39–0.41) than those of columns packed with FPPs (0.35–0.38) because of the higher shear friction between SPPs that prevents them from forming dense beds. Eventually, SPPs will reproducibly deliver an h_min value as small as 1.4. This is also still far from the ultimate h_min of 0.7.

Remarkably, elimination of the velocity depth shown in Figure 7 can be achieved with sub-2-µm particles, but only for capillaries (11,12). In capillaries, the intermediate and dense wall region now extends over most of the column diameter making the bed structure more radially uniform than those in wider columns. As discussed above, an h of 1.05 was obtained for 1 m × 75 µm capillaries packed with 2-µm FPPs by combining slurry concentrations (200 g/L) in an 80-kHz sonication bath (6). High slurry concentrations and narrow internal diameter columns eliminate the differential particle strain while sonication likely minimizes the occurrence of undesirable large voids. This recipe, regrettably, does not apply to analytical columns because their column aspect ratio, d_c/d_p, is too large (>1000).

Why Don't Microbore (1-mm i.d.) Columns Perform Well?

There is still a puzzling observation regarding the performance of micro-HPLC columns with internal diameters of ~1 mm. The h_min values of the best-packed 1-mm i.d. columns are typically between 2 and 3. An unusually steep C-term characterizes these columns in the van Deemter plot (31). It is yet unclear whether this is because of the inadequate surface treatment of the 1-mm i.d. tubes, frit dispersion, extracolumn dispersion, or to a poorly packed bed.

In fact, the velocity profile shown in Figure 7 suggests that if the intermediate and dense wall region (140 µm thick) would persist in 1-mm i.d. columns, then the wall region would occupy about 50% of the total column volume and yield the worst wall-to-bulk volume ratios. This could dramatically affect column performance. This hypothesis was then tested from a fundamental viewpoint by deriving a simple stochastic model of trans-column eddy dispersion (32). The general formalism of Giddings regarding eddy dispersion was directly applied (33). It is assumed in the stochastic model that the linear velocity in the wall region is 5% smaller than that in the bulk region as reported in 2.1-mm i.d. columns over the entire 140-µm-wide wall region (22).

The results for the expected h plots are shown in Figure 9 for columns with internal diameters decreasing from 4.6 to 2.1, 1.5, 1.0, 0.8, 0.6, 0.5, and 0.4 mm. Remarkably, the theory predicts that the h_min values and the C coefficients of the van Deemter plots are the largest for column internal diameters in the range from 0.8 to 1.0 mm. It is because the wall-to-bulk volume ratio is close to 1 for such columns. In 4.6- or 2.1-mm i.d. columns, the wall region occupies only 13–25% of the total column volume, and its contribution to column performance is not as critical. As the column internal diameter is further decreased to less than 500 µm, the column performance is expected to improve because the wall region extends over most of the column diameter. The volume fraction of the bulk region now becomes smaller than 15% and, again, as for 2.1- or 4.6-mm i.d. columns, the column appears more uniformly packed than 1-mm i.d. columns. This result most likely explains why 250-µm capillary columns are packed as efficiently as (34) or even better than (6,11,12) 2.1-mm i.d. columns. This result has not been achieved experimentally with 1-mm i.d. columns.

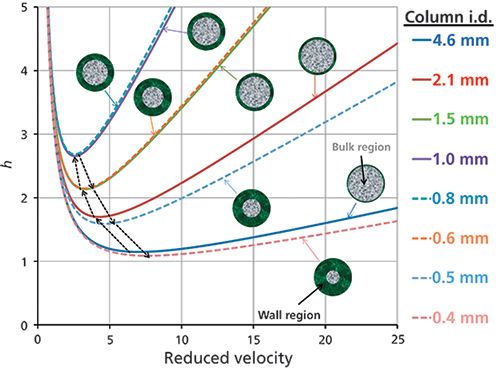
Figure 9: Prediction of the reduced plate height plots of packed columns for internal diameter decreasing from 4.6 mm to 400 µm. In the calculations, the thickness of the dense wall region of the packed bed (green zone) is assumed to remain constant at 140 µm, and the velocity in this region is 95% the velocity in the central bulk region of the bed (gray zone). Note the lowest performance of the 0.8–1 mm i.d. columns because of the enhanced impact of the center-to-wall velocity bias when the volume ratio of the wall to the bulk region is close to 1. (Adapted from reference 32.)

Future Outlook

The science of packing particles in confined tubes has yet to mature to bridge the gap between the best observed and the best theoretically expected performances of columns used in ultrahigh-efficiency chromatography. The current practice of column packing and the fundamental studies about the relationship between the rheological behavior of packed beds, the bed structure, and the column performance have provided unambiguous directions toward the design of improved packing approaches. Concerted efforts including the simulation of suspension rheology, innovative, and well-adapted packing protocols, and new particle technologies are still needed to produce the next generation of analytical columns with h < 1. Inevitably, any promising solution to be developed in the near future will eliminate the nefarious influence of the wall effect on the radial distribution of the flow velocity across the column diameter.

References

(1) A. Daneyko, D. Hlushkou, V. Baranau, S. Khirevich, A. Seidel-Morgenstern, and U. Tallarek, J. Chromatogr. A 1407, 139–156 (2015).

(2) P. Magnico and M. Martin, J. Chromatogr. A 517, 31–49 (1990).

(3) A. Daneyko, A. Holtzel, S. Khirevich, and U. Tallarek, Anal. Chem. 83, 3903–3910 (2011).

(4) A. Daneyko, S. Khirevich, A. Holtzel, A. Seidel-Morgenstern, and U. Tallarek, J. Chromatogr. A 1218, 8231–8248 (2011).

(5) F. Gritti, I. Leonardis, D. Shock, P. Stevenson, A. Shalliker, and G. Guiochon, J. Chromatogr. A 1217, 1589–1603 (2010).

(6) J.M. Godinho, A.E. Reising, U. Tallarek, and J.W. Jorgenson, J. Chromatogr. A 1462, 165–169 (2016).

(7) M.F. Wahab, C.A. Pohl, and C.A. Lucy, J. Chromatogr. A 1270, 139–146 (2012).

(8) M.F. Wahab, D.C. Patel, R.M. Wimalasinghe, and D.W. Armstrong, Anal. Chem. 89, 8177–8191 (2017).

(9) M. Martin and G. Guiochon, in Journal of Chromatography Library, J.F.K. Huber, Ed. (Elsevier, 1978), Vol. 13, pp. 11–40.

(10) M.F. Wahab and R. Deline, personal communication, Optimize Technologies Inc., OR.

(11) S. Hsieh and J.W. Jorgenson, Anal. Chem. 68, 1212–1217 (1996).

(12) J.E. MacNair, K.D. Patel, and J.W. Jorgenson, Anal. Chem. 71, 700–708 (1999).

(13) A.E. Reising, J.M. Godinho, J.W. Jorgenson, and U. Tallarek, J. Chromatogr. A 1504, 71–82 (2017).

(14) I. Leonardis, F. Capriotti, A. Cappiello, G. Famiglini, and P. Palma, J. Sep. Sci . 35, 1589–1595 (2012).

(15) R.A. Shalliker, V. Wong, B.S. Broyles, and G. Guiochon, J. Chromatogr. A 977, 213–223 (2002).

(16) I. Halasz and G. Maldener, Anal. Chem . 55, 1842–1847 (1983).

(17) N. Lambert, S. Miyazaki, M. Ohira, N. Tanaka, and A. Felinger, J. Chromatogr. A 1473, 99–108 (2016).

(18) M. Martin and G. Guiochon, J. Chromatogr. A 1090, 16–38 (2005).

(19) B.G. Yew, J. Ureta, R.A. Shalliker, E.C. Drumm, and G. Guiochon, AlChE J. 49, 642–664 (2003).

(20) G. Guiochon and F. Gritti, J. Chromatogr. A 1218, 1915–1938 (2011).

(21) M. Catani, O.H. Ismail, A. Cavazzini, A. Ciogli, C. Villani, L. Pasti, C. Bergantin, D. Cabooter, G. Desmet, and F. Gasparrini, J. Chromatogr. A 1454, 78–85 (2016).

(22) A.E. Reising, S. Schlabach, V. Baranau, D. Stoeckel, and U. Tallarek, J. Chromatogr. A 1513, 172–182 (2017).

(23) F. Gritti and G. Guiochon, J. Chromatogr. A 1217, 6350–6365 (2010).

(24) B.G. Yew, E.C. Drumm,and G. Guiochon, AlChE J. 49, 626–641 (2003).

(25) S. Bruns, D. Stoeckel, B.M. Smarsly, and U. Tallarek, J. Chromatogr. A 1268, 53–63 (2012).

(26) F. Gritti, J. Chromatogr. A 1533, 112–126 (2018).

(27) R. Aris, Proceedings of the Royal Society of London A: Mathematical, Physical and Engineering Sciences (The Royal Society, 1959), pp. 538–550.

(28) F. Gritti, A. Cavazzini, N. Marchetti, and G. Guiochon, J. Chromatogr. A 1157, 289–303 (2007).

(29) F. Gritti and G. Guiochon, J. Chromatogr. A 1252, 45–55 (2012).

(30) F. Gritti and G. Guiochon, J. Chromatogr. A 1187, 165–179 (2008).

(31) F. Gritti and G. Guiochon, Anal. Chem . 85, 3017–3035 (2013).

(32) F. Gritti, M. Savaria, and A. Felinger, J. Chromatogr. A (in preparation).

(33) C.J. Giddings, Dynamics Chromatography (Marcel Dekker New York, 1965).

(34) F. Gritti, T. McDonald, and M. Gilar, J. Chromatogr. A 1451, 107–119 (2016).

ABOUT THE AUTHORS

M. Farooq Wahab

M. Farooq Wahab is currently a research engineering scientist at the University of Texas at Arlington. He received a postdoctoral fellowship with Professor Armstrong after completing his PhD at the University of Alberta. His research interests include fundamentals of separation science, ultrahigh-efficiency phases, hydrophilic interaction chromatography, supercritical fluid chromatography, and data processing. E-mail: mwahab@ualberta.ca

Fabrice Gritti

Fabrice Gritti is a Principal Scientist at Waters Corporation. He worked with Georges Guiochon as a Research Scientist until 2014 at the University of Tennessee. He completed his PhD in chemistry and physics of condensed matter at the University of Bordeaux (France). Dr. Gritti's research interests involve liquid–solid adsorption thermodynamics and mass transfer in heterogeneous media for characterization and design optimization of new liquid chromatography columns. He has made fundamental contributions to separation science with 250 papers leading to the Chromatographic Society Jubilee Medal in 2013. Email: Fabrice_Gritti@waters.com

ABOUT THE COLUMN EDITOR

David S. Bell

David S. Bell is a manager in Separations Technology and Workflow Development at MilliporeSigma (formerly Sigma-Aldrich/Supelco). With a B.S. degree from SUNY Plattsburgh and a PhD in Analytical Chemistry from The Pennsylvania State University, Dave spent the first decade of his career within the pharmaceutical industry performing analytical method development using various forms of chromatography and electrophoresis. During the past 20 years, working directly in the chromatography industry, Dave has focused his efforts on the design, development, and application of stationary phases for use in HPLC and hyphenated techniques. In his current role at MilliporeSigma, Dr. Bell's main focus has been to research, publish, and present on the topic of molecular interactions that contribute to retention and selectivity in an array of chromatographic processes. Direct correspondence to: LCGCedit@ubm.com