Stationary-Phase Optimized Selectivity in Liquid Chromatography (SOS-LC) for Pharmaceutical Analysis

Frederic Lynen, Ravindra Hegade, Maarten De Beer, Kai Chen, Sander Delahaye, and Pat Sandra, Separation Science Group, Ghent University, Ghent, Belgium

Chromatographic method development for pharmaceutical analysis can benefit from in silico steered serial coupling of column segments containing different stationary phases of varying length. Contrary to column coupling through trial and error, in stationary-phase optimized selectivity (SOS)-based chromatography the retention of all solutes is predicted for all possible column combinations allowing a rational selection of the optimal column combination. The possibilities of the strategy now surpass the initial usage in isocratic high performance liquid chromatography (HPLC) on dedicated commercial column segments, and allow applications in gradient-, green-, preparative-, and in supercritical fluid chromatography (SFC) on conventional column hardware. Current possibilities, pharmaceutical applications, a downloadable algorithm, and weaknesses of the approach are discussed to allow broader implementation of this methodology in separation science.

Despite continuous improvements in chromatographic efficiency over recent decades, achieving sufficient resolution of all solutes in even moderately complex mixtures in high performance liquid chromatography (HPLC) remains a challenging task if robust baseline resolution is intended for all signals. This type of “larger-than-baseline” separation is preferable, especially in a regulated environment, because it allows for enhanced robustness and improved transferability of developed methods. Unfortunately, the limited peak capacity of liquid‑driven approaches inevitably leads to statistically good chances of peak overlap in a chromatogram (Figure 1[a]) (1,2). This can often be addressed through efficiency enhancements, but this strategy rarely leads to an optimal use of the available separation space (Figure 1[b]). More importantly because the resolution only increases with the square root of the efficiency, baseline resolution (Rs > 1.5) is very hard to achieve in contemporary ultrahigh‑pressure liquid chromatography (U)HPLC for all peaks when selectivity differences are limited. For “closely-eluting” peaks, when efficiency is enhanced, the user ends up with, at best, just baseline separated peaks and an elution profile sensitive to minor fluctuations in analytical or even climatic conditions. A solution to this problem can be found in the maximization of the selectivity of each relevant pair of peaks of a separation.

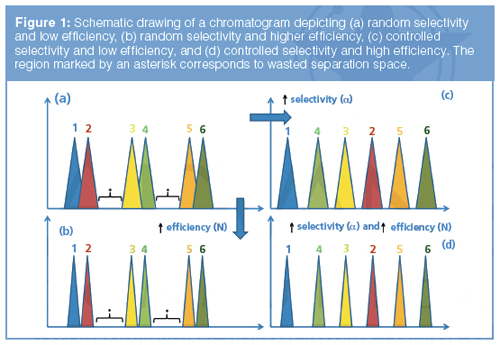
Ideally such selectivity tuning delivers a chromatogram as represented in Figure 1(c). In this case optimal use of the separation space is obtained within the available peak capacity and all solutes are maximally separated from the neighbouring one. Translation to a high-efficiency separation (Figure 1[d]) can then be achieved through columns of increased length or packed with smaller particles. Although there are many ways to optimize the selectivity, few strategies allow such optimal positioning because this requires detailed knowledge of the interplay of solute retention as a function of the modified variables. Contrary to conventional mobile phase method development, which would require a rather exhaustive trial and error approach to possibly achieve this goal, stationary‑phase optimized selectivity liquid chromatography (SOS-LC) offers an effective solution for such a problem. The stationary phase becomes the tunable parameter by connecting variable lengths of column segments with different stationary phases in various ways. The strategy allows a computer‑based prediction of the retention time of solutes on all possible combined columns and subsequent selection of the optimal column configuration, offering a solution for the separation problem at hand. The concept was introduced by Nyiredy et al. a decade ago (3,4). This article summarizes the authors practical experiences with SOS-LC and demonstrates how the strategy has broadened since it was first introduced.

Experimental

Column Hardware: The POPLC Basic Kit 250-5 (Bischoff Chromatography) was used for the coupling of columns (3 mm, 5-µm particle size). This kit contains the following stationary phases: ProntoSIL C18 SH2 (a classic C18 stationary phase), ProntoSIL C18 EPS2 (a C18 phase with embedded polar amide groups), ProntoSIL Phenyl 2, ProntoSIL C30, and ProntoSIL CN 2 (Bischoff Chromatography). For each of these phases, column segments of 10, 20, 40 (2×), 60, and 80 mm were available. The columns (2.1 mm internal diameter [i.d.], 3-µm particle size) were coupled by conventional means and were four Alltima stationary phases (Grace), including an HP C18EPS, HP C18, phenyl, and an HP cyano phase. Each stationary phase was available in various lengths (10 mm, 20 mm [2×], 50 mm and 100 mm), which enabled assembling combined columns from 10 mm to 200 mm in length. These columns were coupled through standard stainless-steel tubing of 105 mm in length and 0.12 mm i.d. (Agilent Technologies).

Software: Isocratic LC work was performed with the POPLC optimizer Software (Bischoff Chromatography) and with the SMSPOPLC optimizer (version 1.24) developed by the authors. Gradient work was also performed with the SMSPOPLC optimizer software (versions 1.23–1.25).

Materials and Methods: All the work was performed on conventional HPLC–ultraviolet (UV) and supercritical fluid chromatography (SFC)–UV instrumentation as outlined in the referred literature (8,9,13,15,18).

Isocatic SOS-LC

Chromatographers regularly couple chromatographic columns containing different stationary phases to address a particularly challenging situation where alterations in mobile phase composition or in temperature have not offered a satisfactory solution. This arbitrary coupling strategy, usually limited to two column types, can successfully separate two closely eluting solutes or increase the retention of compounds eluting too close to the void time (5,6). SOS-LC distinguishes itself from this random coupling by providing simulated chromatograms in much larger numbers (often tens of thousands) of column combinations and then subsequently only the most promising combination is effectively assembled for the intended analyses. Key to this process is that vast numbers of possible chromatograms can be predicted solely based on knowledge of the retention on the pure phases and that as the number of possible column combinations increases, the odds of finding the correct combination to offer a solution for the problem at hand become very high.

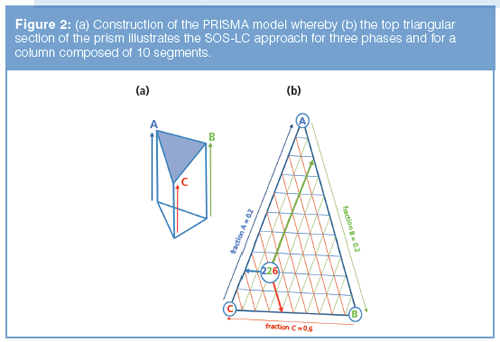
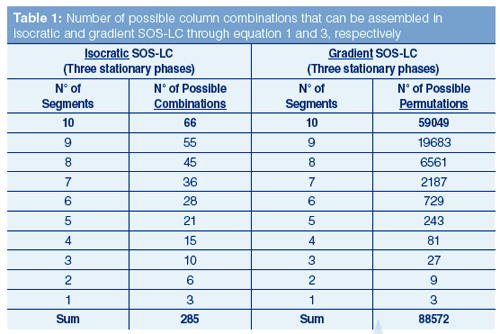
SOS-LC was introduced as an alternative to selectivity tuning through mobile phase optimization whereby the interplay of stationary phases can be represented through the PRISMA model (Figure 2[a]) (3,4). In the SOS‑LC case, the vertical sides of the prism correspond to the retentive strength of the stationary phases for a given analyte. The optimal retention for an analyte lies on a surface that is built by the upper irregular triangle of the prism (Figure 2[b]). It can be realized by a combination of the stationary phases A, B, and C. The extremities of the triangle correspond to columns composed of only one type of stationary phase and refer to the conventional situation. All intermediate positions represent possible combinations of the three phases that can be realized by using a segmented column system. In the represented case, the assumption was made that a maximum of 10 segments (or columns) can be coupled to form the combined column. Note that the length of a segment is freely selectable and that, for example, in the hardware kit available for SOS-LC the minimal segment length is 1 cm. The number of possible column combinations (F) containing 10 segments can be calculated through equation 1:

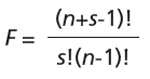
[1]

n and s therefore represent the number of stationary phases and the number of used column segments, respectively. Note that this equation provides the number of combinations when segment order is not considered relevant and when segment repetition is allowed. Hence if this calculation is performed for all column lengths from 1 to 10 segments, 285 different columns can be assembled and will all depict a different retention profile (Table 1). The retention of each solute (for three phases) can be predicted on all columns through equation 2 (7,8):

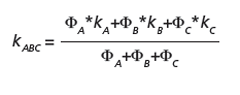
[2]

where k_A, k_B, and k_C represent the retention factors of a given compound on the pure phases and where Φ_A, Φ_B, and Φ_C correspond to the used length of each phase in each possible column combination. After conversion of the retention factors to analysis time, the predicted chromatograms can be ranked according to, for example, the quality of the separation of the critical pair. Hence the highest ranked solution offers the chromatogram depicting the largest spacing between the most challenging peaks and is therefore a very useful separation solution. In practice, the SOS-LC approach can to some extent be completed with conventional HPLC columns because manufacturers offer various lengths of columns that allow many column combinations. A dedicated SOS‑LC column hardware kit can also be used, which allows all possible combinations between 1 cm and 25 cm of five stationary phases in the reversed-phase LC mode to be assembled. The approach permits leak-free coupling of the column segments possible in conventional HPLC (up to about 300–400 bar) with a nearly zero-dead volume. In an example represented in Figure 3 using this hardware, an optimal column was predicted for the separation of 10 steroids in an 30:70 acetonitrile–water-based mobile phase in such a way that over-resolution was achieved for all solutes (9). In this case retention was measured on the five pure phases and chromatograms were predicted on the 15,503 possible column combinations up to 15 cm in length.

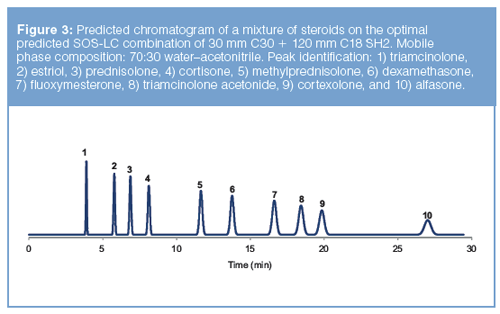
Although this example illustrates the viability of the approach, a number of drawbacks were also evident in the original SOS-LC strategy. These include the limitation to isocratic analysis, the lack of freedom in the selection of the types of stationary phases when using the dedicated SOS-LC column hardware, the concomitant limitation to the reversed-phase mode, and the time required to perform the preliminary experiments. These issues have been or can be overcome as is discussed in the following sections.

Gradient SOS-LC

The isocratic method makes the original approach inadequate for mixtures of compounds that differ significantly in polarity and hydrophobicity. In those cases, gradient analysis is needed to obtain elution within acceptable retention times. Since equation 2-suitable for isocratic SOS-LC-describes the relation between the retention factor and the same invariable mobile phase composition used for each type of stationary phase, it is not suitable to predict elution times under gradient conditions. Equation 2 also does not restrict the order in which the different stationary phases have to be coupled. For isocratic analysis, this order of stationary phases is indeed not a concern and not crucial for obtaining reliable predictions. However, for gradient analysis the order of different stationary phases (for example, a C30 and a CN phase) is relevant. A practical solution to this problem is found through the use of a discontinuous or numerical integration approach whereby a gradient is subdivided into many small isocratic levels (10,11). This allows the position and the migrated distance of a solute in a column at any time during the run to be predicted when taking the local mobile phase composition into consideration as if it were an isocratic plateau. When the sum of all travelled distances equals the column length, the retention time of the solute is obtained. This in silico strategy allows for a necessary flexibility because the process is repeatable for all solutes, stationary phase arrangements, and gradients under consideration. For this approach to be successful, the relationship between retention and the mobile phase composition must, however, be well characterized for each stationary phase (12). Solute retention needs to be measured over several isocratic mobile phase compositions (in our experience five levels allow for good SOS-LC predictions) on each stationary phase. Note that these required preliminary measurements can in principle be limited in time and automated through the use of LC–mass spectrometry (MS) for peak tracking and a column oven equipped with a selection valve.

Once the preliminary data have been obtained, the software allows the retention times of all solutes under various gradient conditions on all column combinations to be predicted and subsequently ranks these combinations to obtain the optimal separation for all solutes. A notable difference with the isocratic approach is that under gradient conditions a different order of the same column segments leads to different elution times. As a consequence, the number of combinations (which are permutations in this case) increases dramatically to:

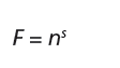
[3]

This is illustrated in Table 1 where 59,049 permutations are now possible for all columns composed of 10 segments of three possible stationary phases. Taking all the shorter columns into consideration, this allows for 88,572 different chromatographic results depending on the number of assembled columns for one particular preselected mobile phase gradient. As the number of possibilities becomes extremely large when using longer columns comprising more segments and more types of stationary phases, especially in combination with different gradient profiles which can also automatically be screened through the same process, brute force calculation methods have to be replaced by genetic algorithm-based solutions allowing the swift finding of optimal stationary phase and gradient conditions. Screenshots of this freely downloadable software (http://www.ssg.ugent.be/) and of representative chromatograms are illustrated in Figures 4(a) and 4(b).

In Figure 4(c) an early gradient SOS-LC example is represented whereby a fixed (preselected) gradient was used (8). Note that baseline separation of all solutes could not be achieved on the pure phases when using the same gradient conditions. In terms of predictive accuracy, some minor shifts in retention time are sometimes observed, as can be seen in Figure 4(c), but this does not result in a notable difference in the predicted elution profile, selectivity, and degree of resolution. The origin of these occasional residual deviations between prediction and experiments can be found in an inaccurate characterization of the solute retention as a function of the mobile phase composition, currently unaccounted effects of pressure on the retention, possibly intra-column void volume differences in the different combinations of the SOS‑LC hardware used, a “too low” number of isocratic plateaus envisaged in the gradient model (8,10,11), and in some cases frictional heating phenomena occurring in the longer assembled columns. By contrast, in many cases predictions are very accurate and it should be noted that the methodology also carries an inherent robustness because the optimally selected column combination is not necessarily the only solution to a chromatographic problem. This is illustrated in Figure 4(d) where the chromatograms obtained on the first and the 20th best proposed column combination were used for a separation of pharmaceutical solutes (13). This illustrates that baseline separation is still easily obtained even when using a less optimal column combination. Conceptually, the example illustrated in Figure 4(d) also shows that chromatographic methods can be conceived in different ways. In this particular example, it was preferred to use environmentally friendly solvents only: water, ethanol, and 0.1% formic acid. Suitable stationary phases were subsequently constructed around these constraints to optimize the separation.

SOS-LC on Conventional Columns

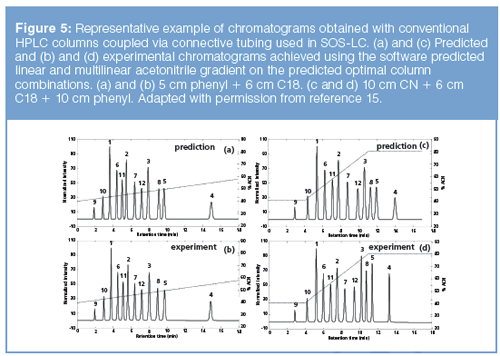
Although using the hardware kit has proven to be very effective, there is no fundamental reason why SOS-LC cannot be performed on conventional coupled LC columns. A possible concern is the influence of extra column void volumes when using conventional column couplers. Solutions to reduce these to a minimum have therefore been proposed (14). On the other hand, in our experience the issue is not necessarily particularly problematic. Figure 5 illustrates that reliable SOS‑LC predictions are also possible on columns coupled with conventional column couplers (15). Note that for maximal predictive accuracy it is important to measure retention factors whereby the system and column void volumes and the dwell time are known.

SOS-SFC

Although the same column hardware can, in principle, be used in HPLC and in SFC, only a limited number of studies describe the arbitrary coupling of columns containing different phases in SFC (16,17). The introduction of the stationary‑phase optimized selectivity supercritical fluid chromatography (SOS‑SFC) approach illustrated that the prediction of retention factors and therefore of optimal column combinations is still viable even when using compressible mobile phases with high CO2 contents such as in SFC (18). In the chromatograms depicted in Figure 6(a–c), predicted and experimental elution profiles are shown. A better correspondence is obtained when mobile phases with the same average density are used both in the preliminary experiments on the pure phases and on the finally assembled column (which is typically longer). Such isopycnic conditions can be reached through adjustment of the back pressure regulator for each column length (19). Note that pressure and density adjustments in SFC are only necessary if one wants to predict optimal columns of any possible length. If only column lengths are predicted that are identical to those used during the preliminary experiments (hence all displaying the same pressure drop and density profile), SOS-SFC can be performed in the same way as in SOS-LC. Thus far the possibilities of SOS-SFC have only been explored in the isocratic mode (18).

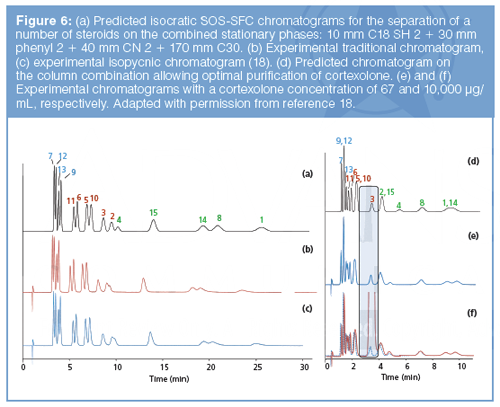
Another SOS-SFC and SOS‑LC aspect that has received little attention is the potential they offer for the faster preparative purification of solutes. In all the described examples the assumption was made that the optimal separation implies the maximal spacing (selectivity) between each peak and column combinations were therefore selected in this way. In the example represented in Figure 6(d–f) one compound was targeted for preparative purification. The spacing around that single peak was therefore maximized in SOS-SFC so that subsequently both volume and concentration overloading of the peak could be safely performed. This significantly increased the purification rate of that solute as a function of time compared to conventional SFC and HPLC approaches (18).

Conclusions

This article illustrates how the original isocratic SOS-LC approach is expanding to (multilinear-) gradient solutions, while it has also demonstrated that implementation on conventional columns, in SFC and in preparative separations, is possible. These developments are only some of the many conceivable possibilities of stationary-phase optimized chromatography and further extensions towards other separation modes such as chiral analysis, hydrophilic interaction liquid chromatography (HILIC), or other separation techniques, such as gas chromatography (GC), are under study (20). Interesting alternative solutions allowing the prediction of optimal gradient profiles in SOS-LC and other column coupling approaches have also been introduced (14,21). An important future development required for broad implementation in pharmaceutical analysis will be the development of machine-controlled approaches comprising (MS-based) peak tracking mechanisms, which will mean that the time consumption problems resulting from preliminary experiments can be addressed through automation of dedicated SOS-LC/SOS-SFC software. Such developments can be pivotal to the future development and use of chromatographic methods without the need for human intervention.

Acknowledgement

The Agency for Innovation by Science and Technology in Flanders and Pfizer are gratefully acknowledged for financially supporting part of this work.

Supporting Material

The gradient SOS-LC software can be downloaded from http://www.ssg.ugent.be/

References

1. J.C. Giddings, J. Chromatogr. A703, 3–15 (1995).
2. J.M. Davis and J.C. Giddings, Anal. Chem.55, 418–424 (1983).
3. S. Nyiredy, Z. Szucs, and L. Szepesy, Chromatographia 63, S3–S9 (2006).
4. S. Nyiredy, Z. Szucs, and L. Szepesy, J. Chromatogr. A1157, 122–130 (2007).
5. H.R. Angelo, N. Beck, and K. Kristensen, J. Chromatogr. B724, 35–40 (1994).
6. R. Sardella, M. Lämmerhofer, B. Natalini, and W. Lindner, J. Sep. Sci. 31, 1702–1711 (2008).
7. I. Gostomski, R. Braun, and C.G. Huber, Anal. Bioanal. Chem. 391, 279–88 (2008).
8. M. De Beer, F. Lynen, K. Chen, P. Ferguson, M. Hanna-Brown, and P. Sandra, Anal. Chem.82, 1733–1743 (2010).
9. M. De Beer, F. Lynen, M. Hanna-Brown, and P. Sandra, Chromatographia69, 609–614 (2009).
10. R. Marques, P. Schoenmakers, C. Lucasius, and L. Buyden, Chromatographia36, 83–95 (1993).
11. G. Vivo-Truyols, J.R. Torres-Lapasio, and M.C. Garcia-Alvarez-Coque, J. Chromatogr. A1018, 169–181 (2003).
12. P. Schoenmakers, H.A.H. Billiet, and L. de Galan, J. Chromatogr.185, 179–195 (1979).
13. K. Chen, F. Lynen, M. De Beer, L. Hitzel, P. Ferguson, M. Hanna-Brown, and P. Sandra, J. Chromatogr. A1217, 7222–7230 (2010).
14. T. Alvarez-Segura, J.R. Torres‑Lapasi, C. Ortiz-Bolsico, and M.C. Garcia‑Alvarez-Coque, Anal. Chim. Acta923, 1–23 (2016).
15. K. Chen, F. Lynen, R. Szucs, M. Hanna‑Brown, and P. Sandra, Analyst 138, 2914–2923 (2013).
16. A.J. Alexander and A. Staab, Anal. Chem.78, 3835–3838 (2006).
17. C.J. Welch, M. Biba, J.R. Gouker, G. Kath, P. Augustine, and P. Hosek, Chirality19, 184–189 (2007).
18. S. Delahaye and F. Lynen, Anal. Chem. 86, 12220–12228 (2014).
19. D.P. Poe and J.J. Schroden, J. Chromatogr. A1216, 7915–7926 (2009).
20. R. Hegade, M. De Beer, and F. Lynen, J. Chromatogr. A 1515, 109–117 (2017).
21. C. Ortiz-Bolsico, J.R. Torres-Lapasió, and M.C. García-Alvarez-Coque, J. Chromatogr. A1373, 51–60 (2014).

Frederic Lynen is a professor in the Department of Organic and Macromolecular Chemistry at Ghent University, Ghent, Belgium, and director of the Separation Science Group.

Ravindra Hegade is a PhD researcher in the Separation Science Group at the Department of Organic and Macromolecular Chemistry at Ghent University.

Maarten De Beer completed his PhD research in the Separation Science Group at the Department of Organic and Macromolecular Chemistry at Ghent University.

Kai Chen performed postdoctoral research in the Separation Science Group at the Department of Organic and Macromolecular Chemistry at Ghent University.

Sander Delahaye completed his PhD research at the Separation Science Group in the Department of Organic and Macromolecular Chemistry at Ghent University.

Pat Sandra is Chairman at the Research Institute for Chromatography (RIC), in Kortrijk, Belgium, Scientific Advisor at anaRIC biologics, in Evergem, Belgium, and Emeritus Professor at Ghent University.

Deirdre Cabooter is the editor of “Pharmaceutical Perspectives”. She is an assistant professor at the Department of Pharmaceutical and Pharmacological Sciences of KU Leuven, in Leuven, Belgium. She is also a member of LCGC Europe’s editorial advisory board. Direct correspondence about this column to the editor-in-chief, Alasdair Matheson, at alasdair.matheson@ubm.com