Static Light Scattering Detectors in GPC/SEC: How Many Angles Do I Need?

This installment of Tips & Tricks focuses on the determination of the molar mass using a light scattering detector as an absolute detection method. The influence of the number of angles is discussed and demonstrated using practical examples.

Gel permeation chromatography/size-exclusion chromatography (GPC/SEC) is a technique that separates macromolecules according to hydrodynamic volume. To obtain molar mass averages (Mn, Mw), a relation between molar mass and elution volume must be achieved. This calibration is typically established using narrow distributed standards. By using this approach, molar masses are derived that depend on the chemical structure of the standard used. Alternatively, static light scattering can be used to determine true molar mass, as well as the radius of gyration, if the molecule’s size is large enough. As the use of static light scattering makes column calibration obsolete, light scattering may provide the only option to obtain true molar masses for applications where no suitable reference materials are available.

Static light scattering detectors on the market differ in the number of available angles. Low-angle (LALS) and right-angle (RALS) light scattering detectors measure at a single angle (for LALS below 15°, for RALS 90°), while multi-angle light scattering (MALS) detectors measure at two or more angles simultaneously.

This instalment of Tips & Tricks investigates different cases and makes recommendations about the number of angles required for a given applicaiton. It also discusses how the selection of angles influences the results.

To show this, raw data of different samples were acquired with a 20 angle MALS detector. These data were evaluated using signals from all 20 angles and with two different subsets of angles with a low and a 90° angle, as well as three angles in the mid-angular range. For demonstration purposes data analysis includes all angles, even the very low ones, and all data points across the chromatogram, including low molar masses and low concentrations, resulting in fluctuations at the chromatogram's start and end.

Theory of Static Light Scattering of Macromolecules in Solution

In GPC/SEC the sample concentrations are typically low. In addition, the separation in GPC/SEC is expected to yield nearly monodisperse slices.

Under these conditions the relation between the intensity of the light scattered by a highly dilute solution of a monodisperse sample and the size and molar mass can be described by the equation below (1):

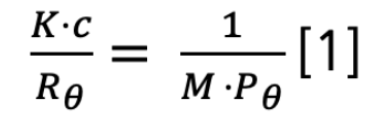
Where:

R_Θ, the excess Rayleigh ratio, is directly proportional to the intensity of the scattered light of the solution in excess of that by the pure solvent. The Rayleigh ratio is a function of scattering angle and concentration.

c is the sample concentration;

M is the molar mass of the eluting molecule;

θ is the angle of observation (scattering angle);

K is an optical constant.

K = 4π²(dn/dc)²n₀²/N_Aλ₀⁴ where:

λ₀ is the wavelength of the laser in vacuum;

N_A is Avogadro’s number;

n₀ is the refractive index of the solvent;

dn/dc is the refractive index increment of the sample.

P_Θ is the form factor and describes the angular variation of the scattered light.

a) For very small molecules that do not exhibit a significant angular dependence of scattered light (isotropic scatterers) P_Θ ≈ 1.

b) For larger molecules (anisotropic scatterers) with sufficiently low values of

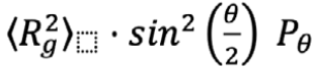
can be approximated by:

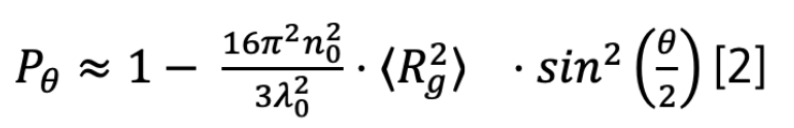
Where

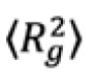
is the z-average of the mean square radius of gyration.

c) For larger values of

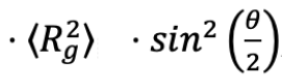
, higher terms of

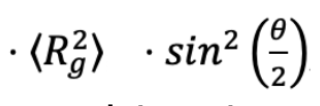
need to be taken into account, resulting in a non-linear angular dependence of the scattered light.

More about Isotropic Scatterers

If the sizes of the molecules are small compared to the wavelength of the laser used, it is assumed that the samples are point scatterers (often referred to as isotropic scatterers). For point scatterers the angular variation of scattered light is negligible (P_Θ ≈ 1) and equation 1 simplifies to:

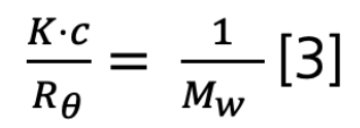
Isotropic scattering for typical light scattering detectors with a red laser is typically observed below 10 nm, which corresponds to approximately 200 kDa or random coil polymer chains, or to molar masses below 1 MDa for dense globular proteins (2).

Since the scattering intensity is independent of the angle of observation, it would be sufficient to measure at just one scattering angle. The missing angular dependence means it is not possible to determine the radius of gyration. However, even for isotropic scatterers, using data obtained from more angles can improve accuracy and precision for statistical reasons (3).

More about Anisotropic Scatterers

If the size of the molecule increases (as is the case when the molecular weight increases or when aggregates are formed) the form factor cannot be approximated by 1, and the variation of scattering intensity with scattering angle needs to be considered, as described in equation 2.

In the case of such anisotropic scatterers, the measurement at just a single, larger angle, for example 90°, would result in an underestimation of molar masses. For sufficiently small angles, where the scattering intensity is measured at an angle relatively close to zero (LALS), correct molar masses can be determined over a wider molar mass range. However, measurement at a low angle is often difficult or even impossible because of the noise close to the primary laser beam. In both cases (RALS and LALS), using a single angle does not allow R_g to be determined.

To determine the molar mass and the radius of gyration of anisotropic scatterers, typically the scattering intensity is measured by several detection angles simultaneously (MALS). A plot of on the x-axis and on the y-axis (referred to as partial Zimm plot) allows the desired molecular properties to be determined. After extrapolation

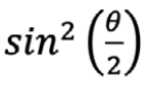
to zero, the y-axis intercept of a partial Zimm plot provides 1/Mw, while the slope is related to the radius of gyration R_g.

In principle, analyzing the scattering intensity at two or three angles should be sufficient to extract molar mass and R_g values from the Zimm plot. However, two angles do not give any information about the uncertainty in R_g in case of noise in one of the scattering signals. By using three angles, it is possible to see if one angle is an outlier and should therefore be excluded from data analysis. However, even if this is unknown, it remains unclear which of the signals need to be excluded from analysis. Therefore, four or more detector signals should be applied. It is also advantageous to have very small angles available (typically below 20°) to obtain reliable information in this region for very high molar masses as these samples may reveal a significant curvature in angular variation of scattering intensity. As the initial slope and the intercept are required to determine R_g and molar mass, extrapolation over large ranges may result in larger errors.

MALS detectors with 2, 3, 7, 8, 9, 18, or 20 angles are typically applied.

Examples for Measurements

Isotropic Scatterer

To demonstrate the influence of the number of angles available for data evaluation, polymers with varying molar mass and dispersity were analyzed using GPC/SEC with a 20 angle MALS detector and using all 20, two (12°, 90°), or three angles (44°, 90°, 132°) for data evaluation. Figure 1 shows the results of a polystyrene sample with a low dispersity.

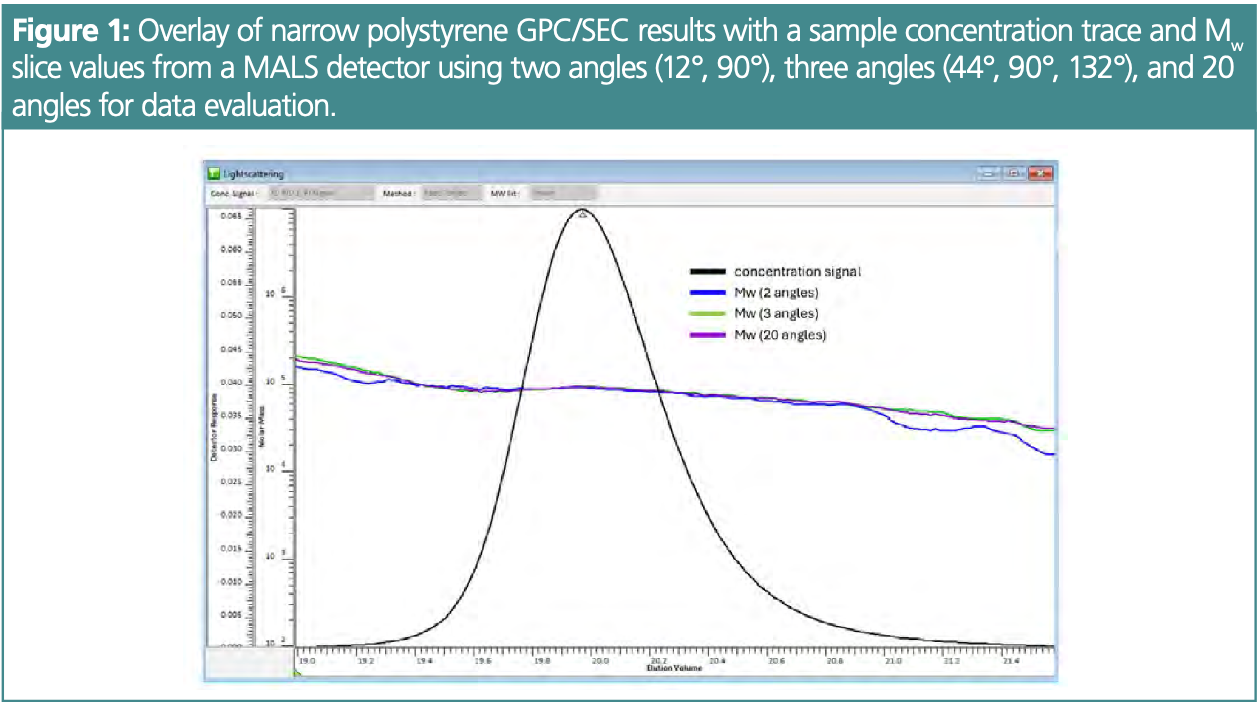
As expected for an isotropic scatterer, the molar masses obtained for all three evaluations were very similar (M_w = 85.0 kDa using two, 87.2 kDa using three, and 86.6 kDa using 20 angles). The fluctuations in the molar mass plot in the low molar mass region (higher elution volume) when only two angles were used was because a low angle was included in the analysis. Noise-free low angle signals are harder to achieve at very low angles because the incident beam is close. The effect is even more pronounced for lower concentrations.

Anisotropic Scatterer

Figure 2 shows an overlay of a broadly distributed anisotropic scatterer evaluated with different (various) numbers of angles. The overlay shows that the molar masses obtained by the different evaluation nicely superimpose on each other. At low concentrations and low molar masses (higher elution volumes) small deviations occur.

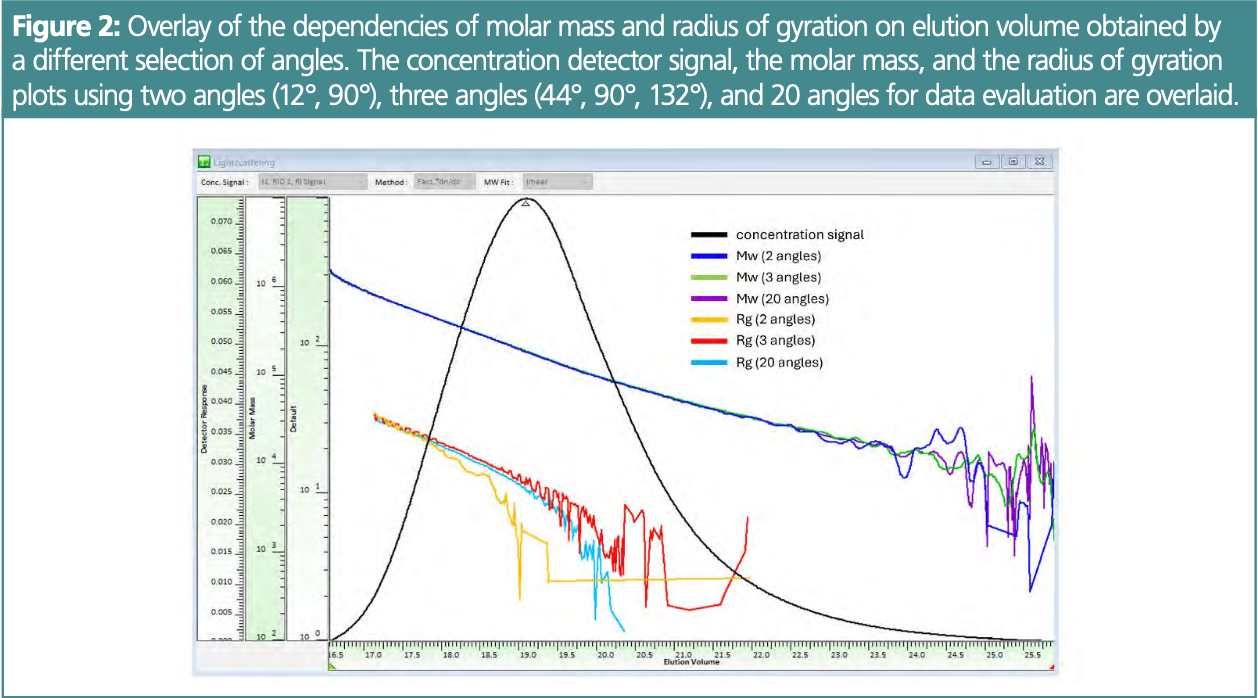
Significant angular variation of scattering intensity mean that R_g values can be determined. However, depending on the number of angles applied in the analysis, the radii of gyration at a given elution volume differ. This is also revealed in the averaged values across the sample, as shown in Table 1.

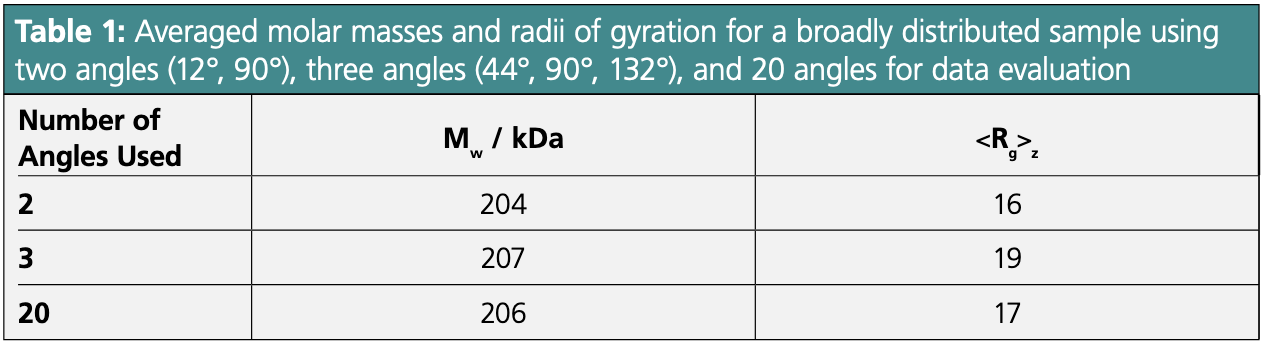
Deactivation of most of the MALS signals and selecting only the angles 12° and 90° or 44°, 90°, and 132° for data evaluation resulted in fluctuations, particularly in the case of the R_g values in the lower molar mass region where the angular dependence is weak.

The M values still superimposed with very small fluctuations in the low molar mass region, which is the result of fainter light scattering signals. In this case the two angles and three angles resulted in large fluctuations (artifacts) of R_g values in the isotropic region of the sample, where the MALS correctly showed no R_g values (no angular dependence). This clearly shows the advantage of more angles, especially when reliable radius information is required. It also shows that the R_g values are much more influenced by fluctuation of the light scattering signal than the M values are.

More about High Molar Mass Application

An anisotropic scatterer with larger values of

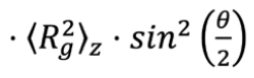
is presented in Figure 3. This shows the Mw and R_g results of a high molar mass linear polysaccharide. In contrast to the measurement of the anisotropic scatterer above, there is a significant difference not only in radii of gyration but also the molar masses (data in Table 2) obtained using two, three, or all 20 angles for data evaluation. Higher terms of

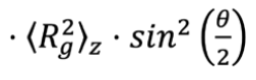
need to be taken into account. A non-linear fit (polynomial 2) was used to account for curvature in the angular variation of scattered light (Figure 4).

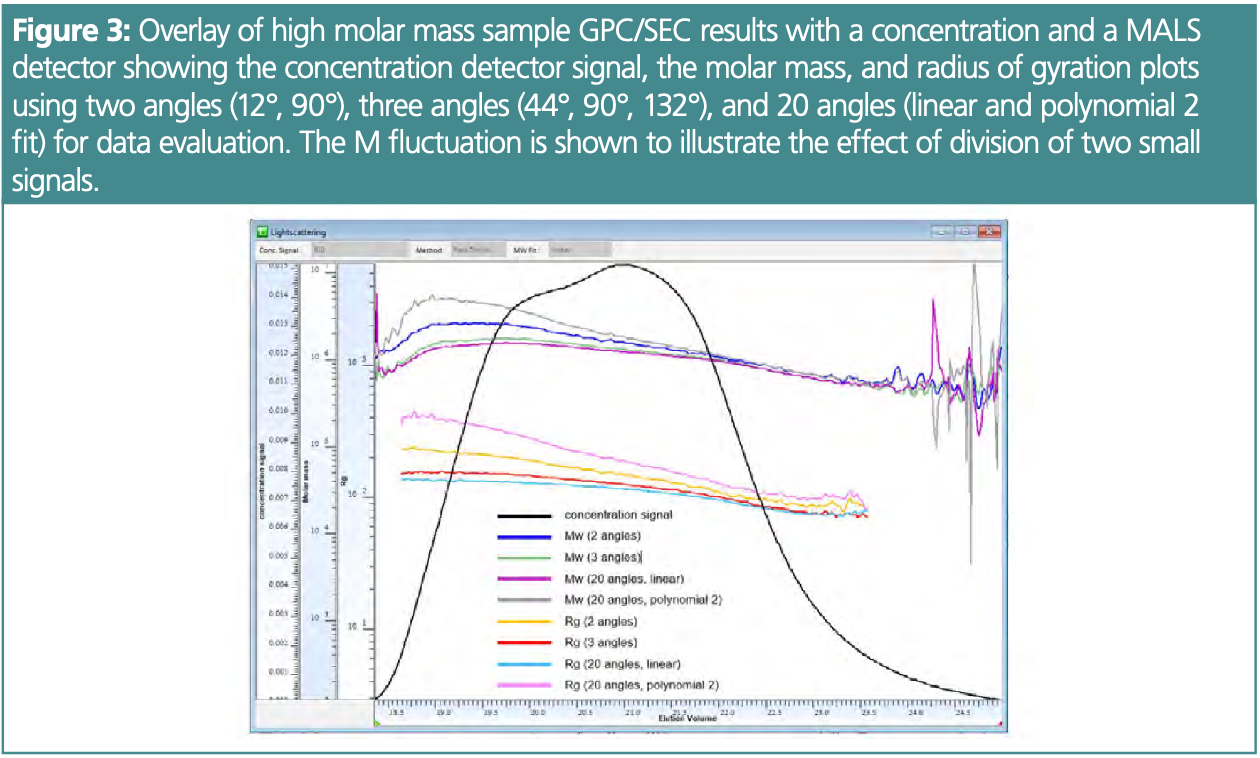
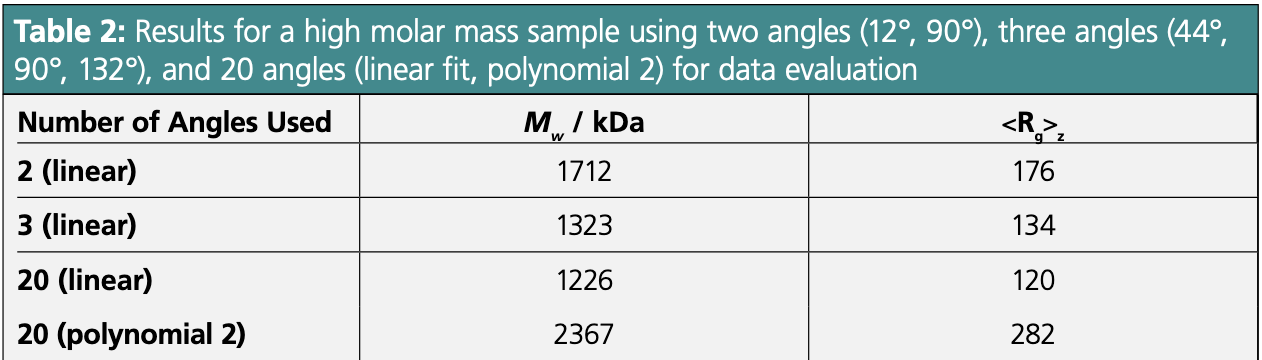
Figure 4 shows the partial Zimm plot obtained at the peak maximum of a high molar mass sample. Clearly the angular dependence reveals a significant curvature requiring either a non-linear fit to the data, or only the data of the smaller angles should be used to obtain the correct result on molar mass and R_g. This type of data evaluation is only possible with a sufficient number of angles. Small angles below 30° are important to make a good extrapolation to angle zero if higher order fit functions are used. This demonstrates the importance of having multiple angles when analyzing large macromolecules that scatter anisotropically.

Summary

• Static light scattering detectors for GPC/SEC enable the determination of absolute molar masses of macromolecules without column calibration (if the refractive index increment of the samples is known). MALS detectors allow the determination of the radius of gyration for samples that show an angular dependence of the scattering intensity. Isotropic scatterers do not show an angular dependence and therefore it is not possible to measure the radius of gyration.
• For isotropic scatterers molar masses can be determined with a single scattering angle. Additional angles reduce fluctuations because of weak signals and improve accuracy and precision.
• Molar masses of larger macromolecules that scatter anisotropically are typically determined correctly with dual- or multi-angle light scattering detectors (multi angle improves accuracy and precision).
• In some cases, very high molar mass samples need higher fit functions for the partial Zimm plots and therefore a sufficient number of angles.

References

(1) Zimm, B. H. The Scattering of Light and the Radial Distribution Function of High Polymer Solutions. J. Chem. Phys. 1948, 16, 1093–1099. DOI: 10.1063/1.1746738

(2) Mori, S.; Barth, H. Size Exclusion Chromatography; Springer, 1999.

(3) Held, D.; Kilz, P. How to Choose a Static Light-Scattering Technique for Molar Mass Determination. The Column 2009, 28–32.

About the Authors

Daniela Held studied polymer chemistry in Mainz, Germany, and works for Agilent Technologies as an R&D director in Mainz. She is also responsible for education and customer training.

Michael Krämer studied polymer chemistry in Freiburg, Germany, and works for Agilent Technologies in Mainz as a solutions specialist. He is responsible for customer support and applications.

Mathias Glaßner studied polymer chemistry in Karlsruhe, Germany, and works for Agilent Technologies in Mainz as a research scientist. He is responsible for GPC/SEC particle modification and quality control as well as customer support.