Nonlinear Predictive Modelling Enables In Silico Optimization of Chromatographic Methods for Complex Stationary Phase‑Analyte Interactions

The development of robust analytical assays for separation and analysis of complex multicomponent mixtures can often be challenging, reflecting the increased complexity of new medicine and vaccine processes. In silico liquid chromatography (LC) method development strategies for small molecules have reached a mature stage across the pharmaceutical industry. However, a straightforward approach for large molecules remains elusive because of conformational changes that can often influence chromatographic retention. Nonetheless, an excellent correlation between experimental and predicted retention time is possible by deploying the correct regression retention models in terms of ln k vs. %B and ln k vs. 1/T (Δt_R < 0.1%). Excellent outcomes generated through in silico chromatographic method development of large molecules using different chaotropic and denaturing mobile phases are illustrated. Linear and nonlinear (polynomial regression) retention models using readily available software were deployed as a function of several chromatographic parameters (gradient slope and column temperature) for a variety of proteins (12–670 kDa) and peptides.

Modern pharmaceutical R&D of increasingly challenging therapeutics and vaccines requires a commensurate level of innovation to deploy critical analytical methods across functional areas (1). Several strategies are commonly applied to the separation of biomolecules, such as ion-exchange chromatography (IEC), hydrophobic interaction chromatography (HIC), and reversed-phase liquid chromatography (LC). While reversed-phase LC is known to cause conformational changes of biomolecules under chromatographic conditions, it remains one of the most utilized techniques for purity assays, with a convenient deployment across manufacturing settings. In addition, reversed-phase LC allows for a straightforward hyphenation to mass spectrometry (MS), which is critical in enabling characterization efforts.

The use of acids, ion-pairing reagents, and chaotropic reagents in reversed-phase LC mobile phases disrupts noncovalent interactions and results in protein unfolding (2,3). In contrast to small molecule pharmaceuticals, these protein‑based targets are highly sensitive to small changes in mobile phase composition and temperature, requiring more challenging method development and optimization endeavours (4). Reversed‑phase LC method development of a protein‑based sample can benefit from one-dimensional (1D)- and two‑dimensional (2D)-LC multicolumn screenings, especially when dealing with complex mixtures (5,6). After the selection of suitable separation conditions, in silico method development software can be leveraged to facilitate an effective optimization output. During this process, it is crucial to deploy the correct retention models of ln k as a function of %B and 1/T, which are more sophisticated than those deployed for small molecules. Previous studies have demonstrated that it is vital to use second-degree polynomial fits of ln k vs. 1/T when the protein is not completely denatured (4,7,8). However, in the presence of strong chaotropic or denaturing agents, first-degree polynomial regression can deliver good retention time prediction.

Experimental

Optima-grade acetonitrile (CH₃CN) was purchased from Fisher Scientific. Trifluoroacetic acid (TFA) and perchloric acid (HClO₄) were purchased from Sigma-Aldrich, Inc. Water (H₂O) was filtered through a Milli-Q UHPLC-grade filter (Waters). All above reagents were used to prepare different mobile phases, solvents, and diluents. The protein standards were obtained from Sigma-Aldrich, Inc. The cyclic peptide (MW ~ 10–21 kDa) was synthesized by an in-house procedure. The protein mixture consisted of Cytochrome C (MW ~ 12.4 kDa), Ribonuclease A (MW ~ 13.7 kDa), Apomyoglobin (MW ~ 16.9 kDa), Albumin chicken egg grade VI (MW ~ 44.7 kDa), y-globulins from bovine blood (MW ~ 150 kDa), and Thyroglobulin bovine (MW ~ 670 kDa).
A 2.1 mm × 75 mm, 2.7-µm particles, 1000 Å Halo C4 column (Advanced Material Technology, Inc.) was selected. The following chromatographic conditions were selected for reversed-phase method chromatographic modelling and simulation of the protein separation: three eluent gradients (10–70% B in 10 min, 10–70% B in 20 min, and 10–70% B in 30 min) with a flow rate = 0.5 mL/min. All three gradients were executed at three different temperatures (20, 40, and 60 ºC) to build a 3D resolution map. For the cyclic peptide sample, the separation was modelled on a 2.1 mm × 100 mm, 2.7-µm particles, 300 Å Halo C4 column (Advanced Material Technology, Inc.) using three gradients (40–70% B in 5 min, 40–70% B in 10 min, and 40–70% B in 15 min). All three gradients were executed at three different temperatures (20, 40, and 60 ºC) to build a 3D resolution map. The resultant experimental data were processed using ACD/LC Simulator 2015 Release (Version L10R41) (Advanced Chemistry Development, Inc. [ACD]). Linear and polynomial regression retention models using ACD/Labs software were built as a function of gradient slope, column temperature, and mobile phase buffer.

Results and Discussion

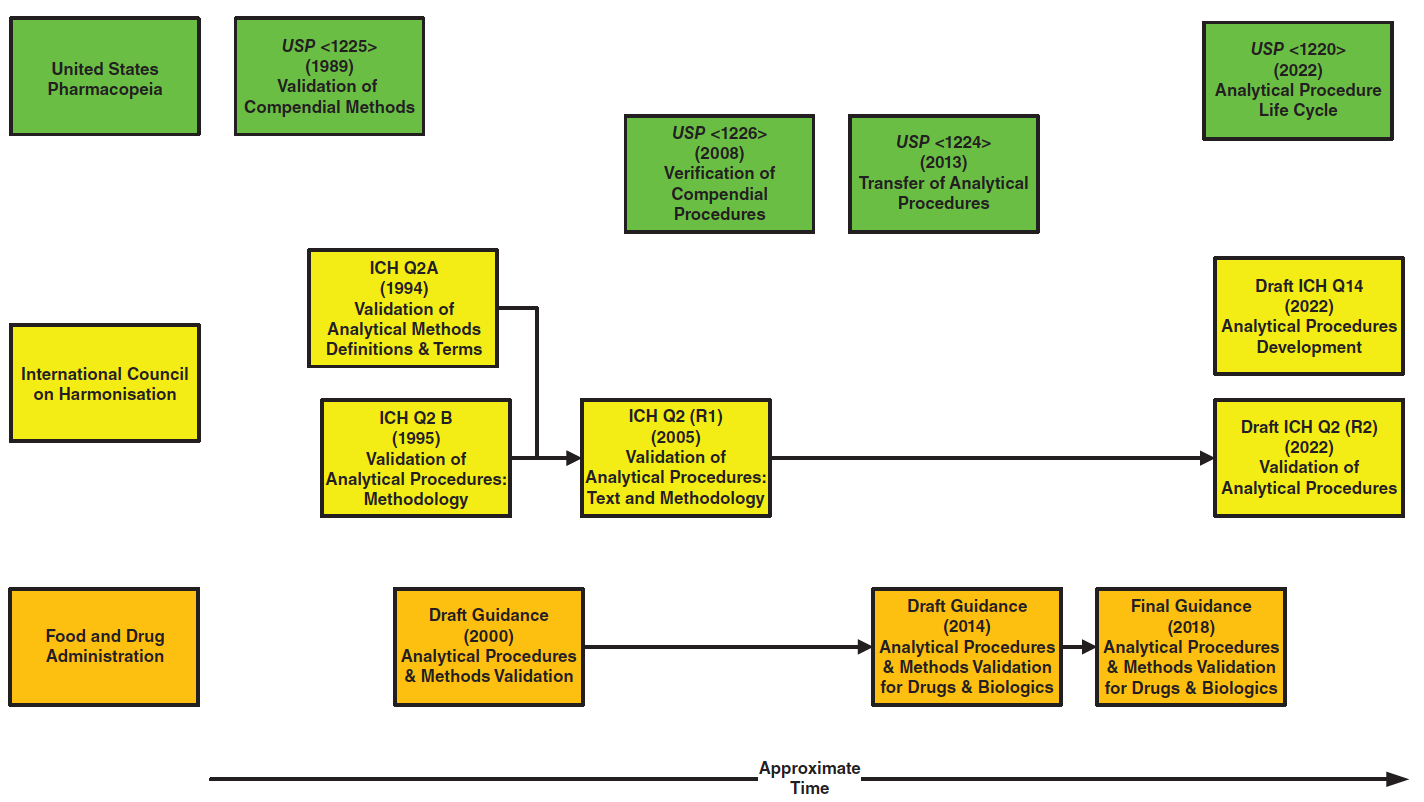
The importance of deploying a second-degree polynomial fit of ln k vs. 1/T when modelling the separation of proteins in the absence of strong chaotropic or denaturing reagents is illustrated in Figure 1. In this example, a mobile phase composed of 0.1% trifluoracetic acid in water and acetonitrile was used to separate a mixture of six proteins (Cytochrome C, Ribonuclease A, Apomyoglobin, Albumin chicken egg, y-globulin, and Thyroglobulin bovine). The resolution map obtained using this nonlinear fit indicated the following optimum conditions: 10–70 %B in 30 min at 50 ºC, as shown in Figure 1(a). Under these conditions, the experimental chromatogram was compared to the predicted chromatograms when first‑ and second‑degree polynomial fits of ln k vs. 1/T were used to build the chromatographic model. Figure 1(b) highlights an excellent accuracy of the retention time of the proteins predicted using the second-degree polynomial fit (%, Δt_R < 0.1). A significant difference between predicted and experimental retention time can be observed when a first-degree polynomial fit was used.

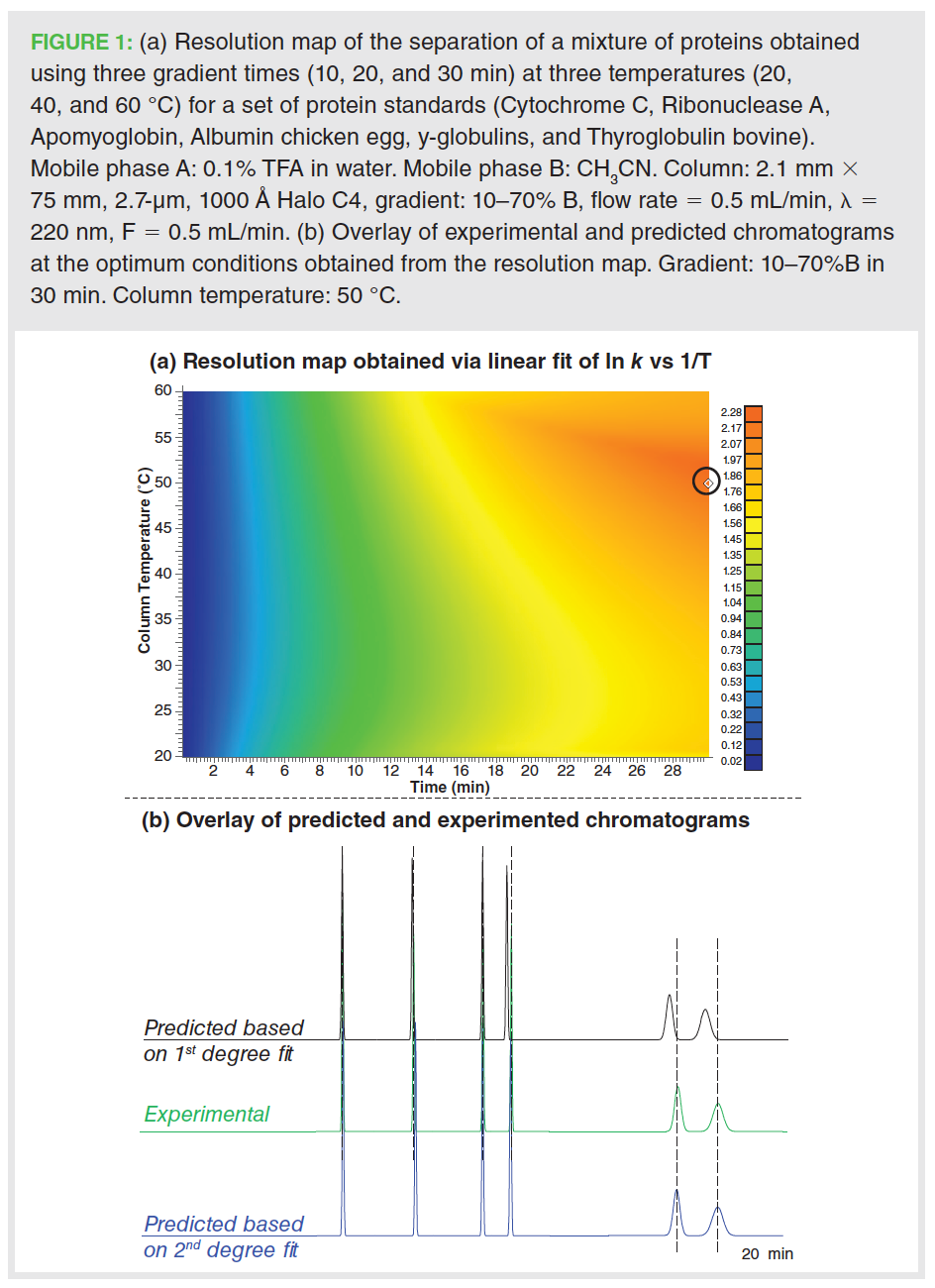
To further understand this difference, the predicted and experimental retention time of Cytochrome C are compared in Figure 2 under 21 conditions (three gradients, seven temperatures). When a first-degree polynomial fit was used for establishing ln k vs. 1/T model (left graph), the retentions times at each of the three gradients show a defined curvature as a function of temperature. These results are indicative of an improper fit of modelled retention time vs. temperature (Figure 2[a]). However, a linear trend appeared when a second-degree fit was deployed (Figure 2[b]). This suggests that such deviation of the experimental retention times from the predicted ones was due to an incorrect polynomial fit in the equation relating ln k vs. 1/T, which could be easily addressed during software simulation.

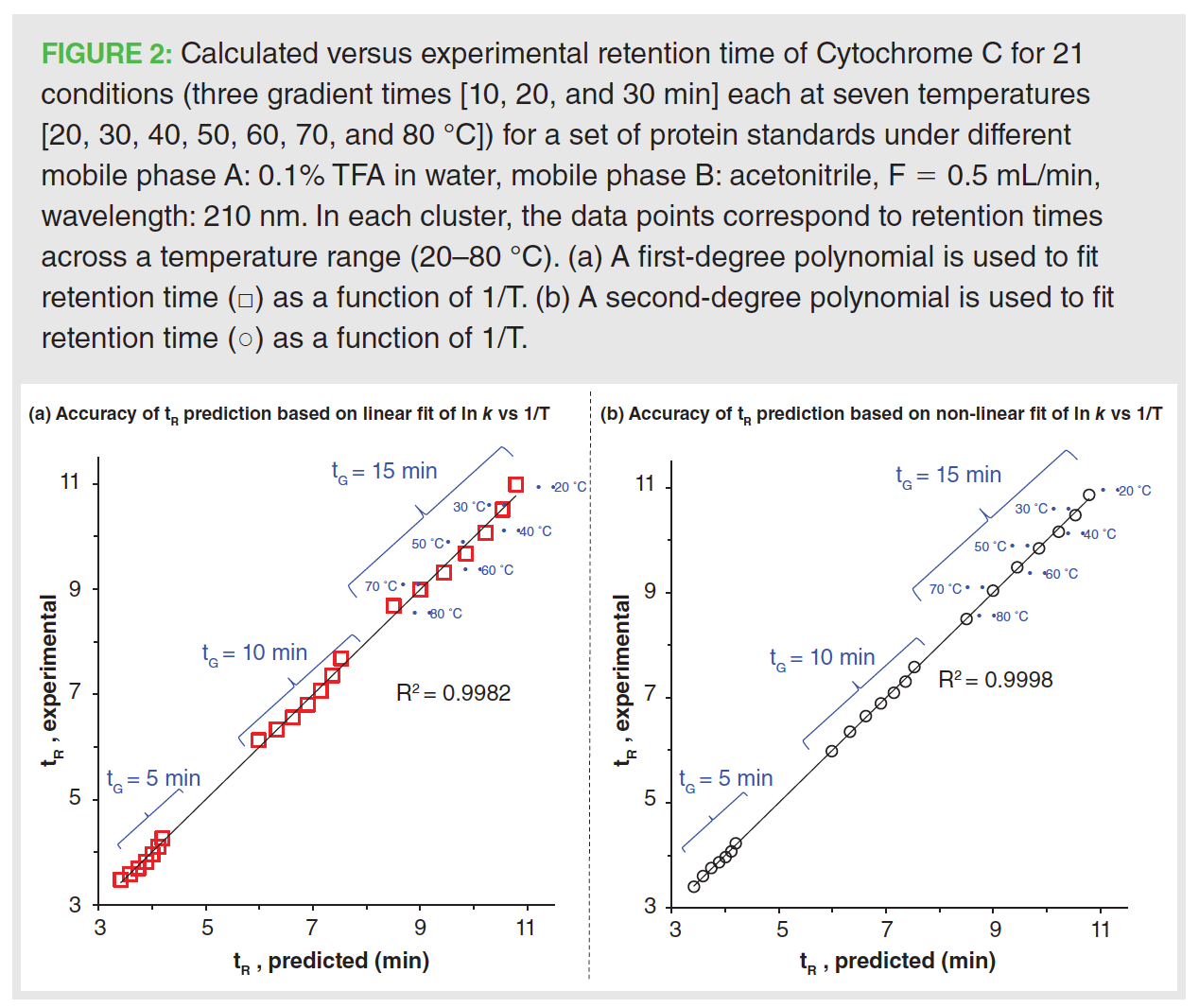
In the presence of a chaotropic reagent (perchloric acid), the accuracy of the retention time modelled using a first-degree fit was significantly enhanced. Figure 3(a) shows the resolution map for the protein mixture modelled using 0.1 M perchloric acid, a stronger chaotrope than TFA. The following optimum conditions (10–70%B in 30 min at 50 ºC) were extracted from the resolution map to investigate the accuracy of the modelling when different polynomial fits were used to model retention coefficient and temperature. It should be noted that a very good accuracy (%, Δt_R < 0.5) between predicted and experimental retention times was observed regardless of which regression model was employed during modelling. Nonetheless, the second-degree fit (ln k vs. 1/T) resulted in a better prediction of retention time when compared to the first-degree one. This conclusion agrees with previous studies reporting the effect of chaotropic and denaturing conditions on the retention modelling of such complex molecules.

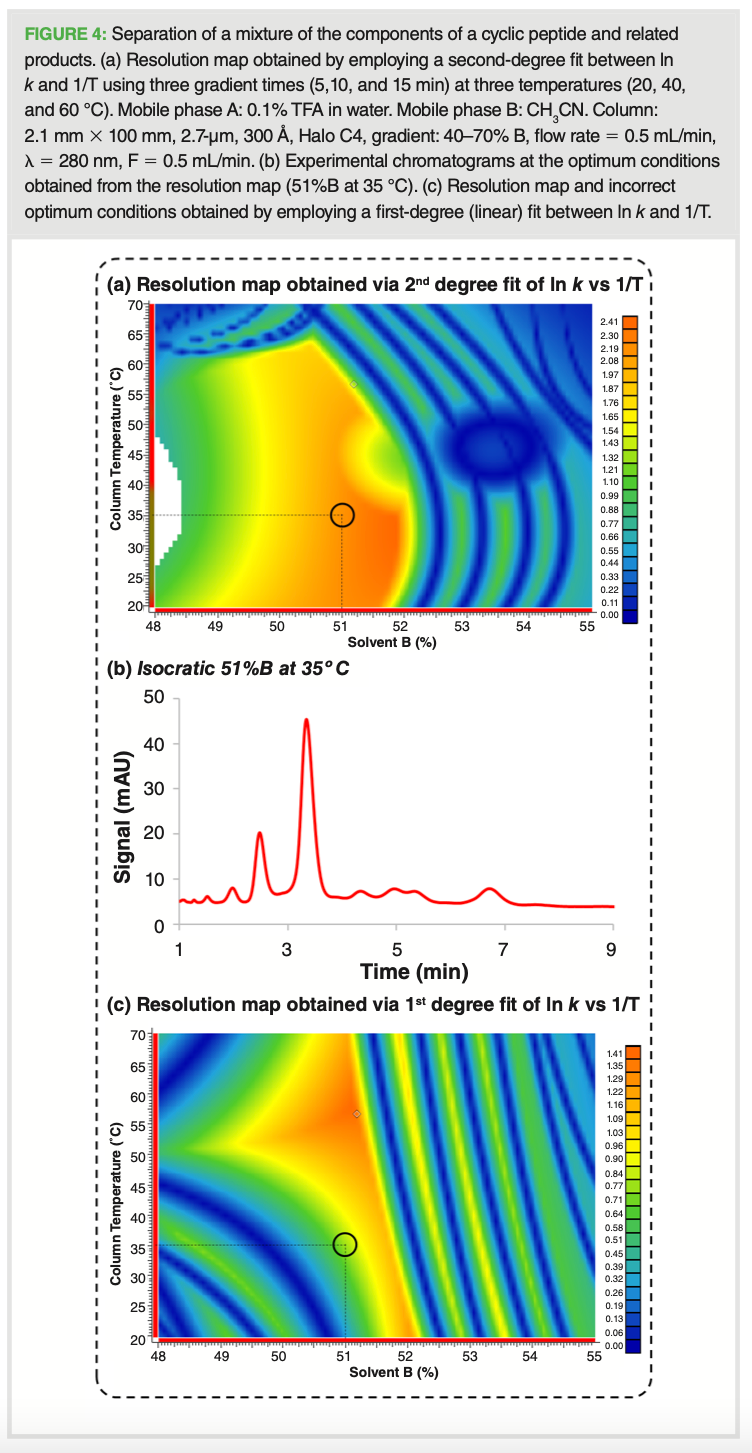
Using the correct equations (ln k vs. %B and ln k vs. 1/T) to model the retention of proteins was essential to establish an accurate and precise computer‑assisted simulation for method development purposes. The separation of a pipeline sample composed of a cyclic peptide (MW ~ 20 kDa) and related components (10–21 kDa) was modelled on a 2.1 mm × 100 mm, 2.7-µm column using 0.1% TFA in water as mobile phase A and CH3CN as mobile phase B. The resolution map obtained highlighted how sensitive the quality of the separation was, specifically in relation to minor changes in percentage composition and temperature (see Figure 4[a]). The optimum conditions obtained from the resolution map, which were established using a second‑degree fit between ln k and 1/T, were localized in a very defined spot. This served to illustrate the importance of computer‑assisted modelling to map the entire separation landscape of a given mixture. The experimental chromatogram under the conditions obtained from the resolution map (51%B, 35 °C) is shown in Figure 4(b). To further assess the effect of using a first‑degree polynomial fit rather than a second‑degree one, the separation was simulated using a first-degree fit, as shown in Figure 4(c). The
risk of using the incorrect model to build retention time must be highlighted as this can lead to incorrect optimum conditions, as demonstrated in Figure 4(a) and 4(c) when comparing the two resolution maps.

Conclusions

Although recent instrumentation and column technology advances have facilitated chromatographic method development for biomolecules, in silico modelling of retention behaviour has been less straightforward because of conformational changes of biomolecules under chromatographic conditions.
To reach satisfactory results in terms of accurate retention time prediction, new in silico optimization approaches must be considered, as illustrated in this article. The choice of the correct polynomial fit is highly important in order to better model the retention time of protein‑based targets. In the absence of chaotropic and denaturing reagents, second-degree polynomial fits of ln k vs. 1/T demonstrate better correlation of experimental and predicted retention time.

References

1. E.L. Regalado, I.A. Haidar Ahmad, R. Bennett, V. D’Atri, A.A. Makarov, G.R. Humphrey, I. Mangion, and D. Guillarme, Acc. Chem. Res. 52, 1990–2002 (2019).
2. A. Makarov, R. LoBrutto, and Y. Kazakevich, J. Liq. Chromatogr. Rel. Technol. 31, 1533–1567 (2008).
3. A. Makarov, R. LoBrutto, and P. Karpinski, J. Chromatogr. A 1318, 112–121 (2013).
4. I.A. Haidar Ahmad, R. Bennett, D. Makey, V. Shchurik, H. Lhotka, B.F. Mann, R. McClain, T. Lu, X. Hua, C.A. Strulson, J.W. Loughney, I. Mangion, A.A. Makarov, and E.L. Regalado, J. Chromatogr. B 1173, 122587 (2021).
5. C.J. Pickens, I.A. Haidar Ahmad, A.A. Makarov, R. Bennett, B.F. Mann, and E.L. Regalado, Anal. Bioanal. Chem. 412, 2655–2663 (2020).
6. H. Wang, H.R. Herderschee, R. Bennett, M. Potapenko, C.J. Pickens, B.F. Mann, I.A. Haidar Ahmad, and E.L. Regalado, J. Chromatogr. A 1622, 460895 (2020).
7. P. Petersson, J. Munch, M.R. Euerby, A. Vazhentsev, M. McBrien, S.K. Bhal, and K. Kassam, Chromatography Today 15–18 (2014).
8. P. Petersson, M. Euerby, J.K. Field, and B.O. Boateng, LCGC Europe 31, 120–143 (2018).

About The Authors

Imad A. Haidar Ahmad received his Ph.D. from Florida State University under the mentorship of AndrŽ Striegel. He completed his postdoctoral research with Peter Carr at the University of Minnesota. He is currently Associate Principal Scientist and Scientific Supervisor in the Analytical Enabling Capabilities Group within MRL’s Analytical Research & Development Department.

Gioacchino Luca Losacco received his PharmD from University of Rome “Sapienza” and his Ph.D from University of Geneva under the mentorship of Jean‑Luc Veuthey and Davy Guillarme. He is currently Postdoctoral Fellow in the Analytical Enabling Capabilities Group within MRL Analytical Research & Development Department, under the supervision of Erik L. Regalado.

Erik L. Regalado is currently the scientific leader of the Method Screening and Purification Laboratories in MRL’s Analytical Research & Development Department, where he previously completed his postdoctoral research with Christopher J. Welch. He obtained his Ph.D. working with Olivier P. Thomas at the University of Nice Sophia Antipolis, France, and Clara Nogueiras at the University of Havana.

About The Column Editor

Adrian Clarke is the editor of “Pharmaceutical Perspectives”. He is the analytical network leader in Technical R&D at Novartis Pharma, in Basel, Switzerland. He is responsible for identifying the function’s strategic direction and technological needs in analytical chemistry. His main interests are liquid-phase separations (LC, 2D-LC, SFC), GC, method development strategies, and also analytical control and regulatory strategies. He has authored many peer‑reviewed articles and presented at multiple conferences on his research activities in separation sciences and pharmaceutical analysis. He is also a member of LCGC Europe’s editorial advisory board. Direct correspondence about this column should be addressed to the editor‑in‑chief, Alasdair Matheson, at amatheson@mjhlifesciences.com