The Long Distance Run

In this month's installment, we examine long-distance running as a metaphor for gas chromatography separations. For those readers who cannot stop thinking about work while on vacation, here is a light treatment of the separation process and a proposal for a chromatography "fun run."

Summer is in full swing this month. Many chromatographers, myself included, look forward to participating in some of the plethora of 10-km, half-marathon, or full-marathon long-distance running events offered worldwide. Besides the obvious benefits to health and fitness, long-distance running offers opportunities for free-running thoughts that can turn the imagination to musing upon seemingly disparate and otherwise unnoticed phenomena.

Long-distance runners often experience the sensation of traveling along a narrow passage — the "tunnel-vision" effect — with reduced awareness of their surroundings beyond a few meters. This is a helpful adaptation of the senses that avoids undesirable events such as tripping over a curb or colliding with the next runner ahead. For a chromatographer, a long-distance run can be perceived as if moving through a flattened separation column. The race starts out with runners bunched tightly together and finishes with runners distributed according to their abilities. At first glance, this result resembles a chromatography separation, but how true is the likeness?

Long-distance runs and chromatography separations do share some attributes such as a long, narrow course and a chromatography column; runners and solute molecules; segregation of runners into groups and the formation of discrete peaks; timing-chip sensors and a chromatography detector; run completion times and retention times; an organized run start and an injection system. Coincidentally, if the width of a long-distance race course is about 3 m then the length-to-width ratio of a 42.2 km marathon course is similar to that of a 10 m × 0.75 mm gas chromatography (GC) column.

Some chromatography authors have remarked on the parallels between long-distance runs and a chromatography experiment (1,2). The concept of a chromatography theoretical plate can be applied to the statistical distribution of runners finishing a race. For example, the finishing times from a half marathon in 2012 had the distribution shown in Figure 1. The resemblance to a tailing peak is unmistakable, but really this is just the statistical nature of two disparate processes as we will see shortly. The finish-time distribution has the equivalent of about 40 total plates on the basis of the time of the distribution maximum and its width at half-height. Blumberg (3) calculated that the 2001 New York City Marathon spread the runners into a distribution with about 70 equivalent plates. Between these two races, the average plate height for a half to full marathon comes to about 560 m. Scaled down to the proportionately sized 10 m × 0.75 mm GC column, that is a plate height of about 130 mm — more than two orders of magnitude larger (worse) than might be expected for this size GC column. I'd send that one back to the manufacturer right away.

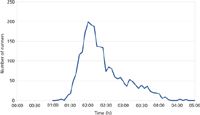
Figure 1: Distribution of finishers in a half marathon from 2012. Total number of runners = 2416; maximum Tmax occurs at 2.0 h; width at half-height wh ≈ 0.75 h; apparent plate number = 5.54
• (Tmax / wh)2 = 40.

Clearly, there are significant similarities and differences between chromatography and long-distance running. This article examines the basic elements of a chromatography experiment — flow, diffusion, and retention — and imagines how a long-distance race might be arranged to better represent chromatography. Along the way I will try to describe rules for a modified long-distance chromatography "fun run" as a challenge to anyone who would like to try it out for a short distance, perhaps as a 5-km (3.1-mile) course.

Flow

First of all, is a normal long-distance race a form of chromatography? Definitely not. The basic definition of chromatography is "a physical method of separation in which the components to be separated are distributed between two phases, one of which is stationary (stationary phase) while the other (the mobile phase) moves in a definite direction" (4). Here are two principal differences between a chromatography experiment and a long-distance race: The former has both mobile and stationary phases, and the latter has neither. Long-distance runners generally do move in a defined direction at least.

This discussion will be limited to column chromatography, in which solutes move through a column under the influence of mobile-phase flow. Thin-layer chromatography (TLC) could be simulated by travel along the length of a football field, but the pace would be quite slow. Sedimentary field-flow fractionation (SFFF) might take the form of a carnival ride, but it would be prohibitively dangerous to the riders acting as the particles being separated.

When not retained by a stationary phase, all solutes move along a chromatography column at the same average speed as the mobile phase. Long-distance runners, in the absence of a mobile phase, move along at individual speeds or paces that depend on their abilities, how they feel that day, what they last ate, the weather, how much sleep they got the night before, and any other number of human factors. The statistical distribution of their finishing times derives from the range and distribution of their speeds and not from a process resembling chromatography. However, a chromatographic process can be simulated by runners with a little creative imagination.

Although it does not seem practical to create a physical equivalent to mobile-phase flow in a long-distance race — imagine thousands of "mobile-phase" runners pushing the true competitors along — major long-distance races do include a similar feature: pace runners. A number of experienced runners who can travel at a near-constant pace are designated as pacers. They can usually be seen with balloons for visibility and a sign that indicates their pace, such as 10:00 min/mile, 9:30, 9:00, 8:30, and faster. It is often difficult for the average runner to maintain a constant pace throughout. Pacers provide a reference for the runners to follow, should they choose to do so. In a chromatography simulation, runners could use the pacers as mobile-phase flow references.

A chromatography column encompasses some complex physical phenomena that are without a clear equivalent in a race. For one thing, mobile-phase velocities in the column are slower near the column wall and faster near the center, an effect caused by shear forces at the wall under laminar flow conditions. At normal GC linear velocities a parabolic laminar flow profile forms, as shown in Figure 2. Solute molecules that reside between the average velocity zone (b) and the column wall move along more slowly, while those that are close to the column center area approach the maximum velocity (a).

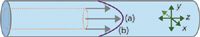
Figure 2: Laminar flow and diffusion: (a) Maximum velocity is at the center of tube; (b) the average velocity defines a cylinder, shown in orange, approximately 65% of the distance to the wall. Velocity at the wall is zero. At an average helium carrier-gas velocity of 40 cm/s in a 10 m × 0.75 mm column at 100 °C, the maximum velocity will be approximately 65 cm/s. x, y, and z are solute diffusion vectors. The y-vector would be zero in a flat footrace.

Here is the first chromatography-related characteristic that can be applied to a modified long-distance race being run more like it was a separation: Conduct it in a way that better reflects mobile-phase flow by instructing all of the pacers to run at the same constant speed while asking the runners to establish the semblance of a laminar flow profile.

Rule 1, Flow: Groups of runners start at gated times and follow a designated pacer; all pacers run at the same constant speed. Individual runners near the center of the course should exceed their pacer's speed by up to 50%; runners halfway between the center and the wall should match the pace; runners close to the sides of the course should reduce their speed to 50% less than the pace or slower. In other words, walk at the course boundaries and run faster in the center.

With the average pace remaining constant in a laminar profile along the length of the course, this arrangement is missing one detail that applies to GC. Gaseous column flows are more complex because the mobile phase is a compressible fluid, as opposed to the noncompressible nature of a liquid chromatography (LC) mobile phase.

The GC mobile phase enters at the column inlet pressure and exits at the outlet pressure, either atmospheric or vacuum. The mobile phase expands down the length of the column and the local linear velocity increases accordingly as a function of the distance along the column in the direction of flow. This gas expansion effect is larger at the higher pressure drops encountered with narrow-bore columns. For a 0.75-mm i.d. wide-bore column running at 10 mL/min, which represents an average linear velocity close to 40 cm/s, the inlet gauge pressure is only about 4.4 kPa (0.64 psig). The velocity at the column entrance will be only several percent lower than at the exit, so it is easy to ignore this effect in the case of a long-distance run simulation. This is fortunate, because otherwise the runners would have to increase their pace by a factor of four or more over the course of the run.

Diffusion

So far our chromatography–race simulation has runners moving along the race course in a roughly parabolic profile. Runners in the center are moving faster than the average pace while runners at the edge are moving much slower. Slower, less-capable runners would naturally prefer positions closer to the outer boundary, and faster runners would favor the center. If undisturbed, this situation would create a very wide distribution of finishing times. Fast runners would run fast throughout the race and slow runners would lag far behind the average pace. It is essentially the same as a real race and would result in a distribution similar to Figure 1.

Solutes have some degrees of freedom when traveling down a chromatography column in the mobile phase; they are free to diffuse in random directions relative to the containing mobile-phase flow. The rate of gas-in-gas diffusion is significant: n-Hexane, for example, diffuses in helium at about 0.5 cm²/s at 130 °C and 101 kPa, therefore, a helium atom could quickly span the width of a 0.75-mm i.d. column if it moved in a straight line.

Diffusion of solute molecules through the mobile and stationary phases plays a pivotal role in any chromatography separation. The diffusion path of a solute can be envisioned as a series of random movements along three vectors: in the longitudinal flow or z-direction, and in the x- and y-directions at right angles to the flow, shown in Figure 2. Constant diffusion through the mobile phase causes a degree of solute band broadening as solutes move both forward and backward relative to the local velocity, as well as back and forth across the velocity gradient between the column center and the inner wall.

In the real long-distance race, runners are not constrained to fixed lanes; they are free to move from the inside to the outside of the course and back again. This movement resembles diffusion, and rule 2 induces them to do the same in the chromatography race, as a simulation of solute diffusion through the carrier gas.

Rule 2, Diffusion: Runners should move at random back and forth across the width of the course while continuing to follow rule 1. While in the running lane, each runner should match the official pace on average.

According to rule 1 they also must speed up at the middle of the course and slow down at the sides. The biggest challenge: To mimic solute diffusion the runners should continuously move across the zones, not just once in a while. They must constantly change directions and speeds during the whole race, while always moving in a net forward direction in a type of fartlek run scheme. (Note: A fartlek run is a training technique in which varying the pace during a run to move between anaerobic and aerobic exercise is intended to develop better speed and endurance.) They will end up covering a longer distance than if they ran in a single linear direction, but that is part of a chromatography long-distance run challenge.

With all runners' average speeds now close to their pacer, the runners will all finish at nearly the same net time. Their distribution at the end should approach a Gaussian shape if their cross-course zone shifting is truly random and the associated forward speed changes are consistent. These runners represent unretained solutes in a simplified chromatography experiment on a column with no stationary phase or packing. Those competitors used to running at a slower pace might be nearly exhausted, while those with faster abilities would be left less taxed and perhaps would wonder what the challenge was.

Retention

Thus far in the chromatography simulation, runners move along the course and, if they follow the rules, find themselves spread in a single peak-like distribution at the finish line. There is nothing in place to differentiate the runners: they all move along at the same average pace. The simulation needs a retention mechanism to separate the runners into multiple groups that represent peaks.

Diffusion delivers solute molecules to the column wall for retention and subsequent release back into the mobile phase. Retained chromatography solutes spend time on or in the stationary phase, in proportion to their affinity for it relative to the mobile phase. A number of retention mechanisms are used in GC, the most common being gas–liquid partitioning in which solute molecules dissolve into the stationary phase in proportion to their solubility. The more soluble molecules spend less time overall in the mobile phase than less-soluble ones and so they are eluted later in the run. The ratio of a solute's concentration in the stationary phase to its concentration in the mobile phase is called the partition coefficient, K.

One potential retention parameter for the runners' simulation is each runner's average pace in a normal long-distance race. Faster runners achieve a lower average pace, which makes pace a good substitute for a partition coefficient. Long-distance runners travel at average paces from around 6:00 min/mile (3:43 min/km) up to about 14:00 min/mile (8:41 min/km). Walkers travel at a slower pace; 15:00 min/mile (9:19 min/km) is considered a brisk walk.

To make the simulation easier to achieve, 8:00 min/mile (4:58 min/km) on average could be set as the pacer speed that represents unretained runners. All runners who can keep an 8:00 min/mile average pace while following the rules are assigned a race number that starts with 08. Slower runners and walkers receive higher race numbers as outlined in Table I.

Table I: Runner pace and retention for a 5-km chromatography simulation. Calculated for a total of 50 stops along the race course.

Chromatographers usually express retention in terms of the retention factor, k, which is a function of retention time normalized to the unretained peak time:

A peak with a retention factor of 1.0 will have taken twice as long to pass through the column as a completely unretained peak. Table I includes the average expected finishing times for each group, as well as their apparent retention factors.

Rule 3, Retention: Runners with race numbers of 1000 or higher receive two small bags. One contains 50 small tokens and the other is empty; both are to be pinned to the runner's bibs. (Optional: place a flag every 100 m along the 5-km course.)

When a course boundary is encountered, runners with tokens will step out of the boundary and out of the way of other runners, then stand in place for the count of the first two numbers of their race bib minus 8 s. While pausing, runners will transfer one token from the full bag to the other. All tokens are to be transferred before the finish line. Throwing tokens on the ground is grounds for disqualification. The 50 flags at 100-m intervals serve as reminders to pause and transfer a token, although it is acceptable to use all of the tokens, one at a time, at random points along the course.

As the race progresses, four groups of runners should develop: the unretained 8 min/mile group, the 10 and 14 min/mile runners, and the 14–22 min/mile run–walkers. For increased accuracy it would be advisable to launch additional 8 min/mile pacers at regular intervals so the retained runners can better judge the correct average linear velocity.

The regime outlined in rule 3 will cause some stress for less-capable runners: each has to be able to travel at an average 8 min/mile pace for about 100 m while in the race course, punctuated by the specified rest periods at the sides. Those assigned to the slowest group won't actually do any walking. Their average pace will work out to the equivalent of a walk, but they will have to run at an 8 min/mile pace on average to each stopping point and then wait for 22 s before continuing. That should be enough time to catch their breath if necessary. All runners will need to move randomly out and across the width of the course while speeding up or slowing down according to the laminar flow and diffusion schemes outlined in rules 1 and 2.

The Finish Line

So, there you have it: a long-distance running version of a GC separation. Average column flow is represented by pace runners, solute diffusion is simulated by the random movement of runners back and forth across the race course, and retention is achieved by runners' pausing at the course-side boundaries for variable periods. Following the scheme in Table I that divides the runners into four categories each with its own retention factor, four distinguishable groups of runners should arrive at the finish line of a 5-km run at average times that represent their retention factors. The degree of resolution of the runner groups is a little difficult to predict since it relies on so many human factors (see the sidebar "Extracourse Contributions to Runners' Bandwidth"), but the plate numbers may be larger than is observed in a standard long-distance race, especially for the last group to finish.

Extracourse Contributions to Runners’ Bandwidth

References

(1) H. Iwase, Curr. Sep. 20(4), 147–149 (2004).

(2) Anonymous, "Continuous Analytical Measurement—Chromatography," update blog entry in http://iamechatronics.com/notes/general-engineering/420-continuous-analytical-measurement-chromatography.

(3) L.M. Blumberg, Temperature-Programmed Gas Chromatography (Wiley-VCH, Weinheim, Germany, 2010), p. 224.

(4) L.S. Ettre, Pure and Appl. Chem., 65(4), 819–872 (1993).

John V. Hinshaw "GC Connections" editor John V. Hinshaw is a Senior Scientist at BPL Global, Ltd., in Hillsboro, Oregon, and a member of LCGC's editorial advisory board. Direct correspondence about this column to the author via e-mail: lcgcedit@lcgcmag.com.

John V. Hinshaw