LC Method Scaling, Part II: Gradient Separations

If scaling isocratic separations is so simple, why is gradient scaling so confusing?

In last month's "LC Troubleshooting" installment (1), we looked at how to scale isocratic separations when the column size or packing particle size is changed. The process is quite simple. First, find a column with approximately the same plate number, then adjust the flow rate to give an acceptable pressure. The most common problems that result from mistakes in this scaling process give somewhat lower resolution than is expected or higher pressures. With gradients, unexpected consequences may occur from the changes that may be relatively unimportant in isocratic methods. In this month's discussion, we turn our attention to the proper scaling of gradient methods.

Resolution and Plate Number

Last month (1) we looked at the fundamental resolution equation for isocratic conditions:

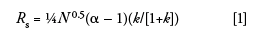
where R_s is resolution, N is the column plate number, α is the separation factor, and k is the retention factor. A similar equation can be stated for gradient separations:

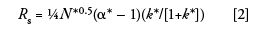
where N* is the effective plate number under gradient conditions, α* is the gradient separation factor, and k* is the gradient retention factor. As with isocratic conditions, we must be careful to keep from changing the chemistry of the system by keeping the same brand and series of column packing, the same mobile phase, and the same column temperature. We'll see below that we have some additional factors to be careful of with gradients. If we keep these things constant, k* and α* (the ratio of k* values for two adjacent peaks) should remain constant. If α* is unchanged, we will obtain the same resolution if we keep the same column plate number.

The column plate number cannot be measured easily under gradient conditions, so we measure it under isocratic conditions. Because the plate number is a characteristic of the column, the use of isocratic conditions is not a problem. We use the same approach we used with isocratic conditions to select a column with an equivalent plate number so that we maintain the same resolution with the scaled method. We saw that we could obtain approximately the same plate number if we kept the column length-to-particle-diameter ratio constant within a range of +50% to -25%. Thus, we can determine the desired column length from

where L₁ and L₂ are the column lengths, and d_p1 and d_p2 are the particle diameters of the original and new column, respectively. So far, nothing is different between the isocratic and gradient scaling process.

Gradient k* Is the Key

With isocratic separation, we saw that the flow rate had no influence on the retention factor, k, and only a minor influence on the plate number, N. So, although we often start the isocratic scaling process by keeping the linear velocity of the mobile phase the same, from a practical standpoint the flow rate is adjusted to maintain an acceptable pressure. With gradients, however, a change in the flow rate without some other compensating change can generate problems. This is because k* is affected by flow rate:

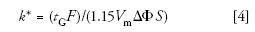
where t_G is the gradient time (minutes), F is the flow rate (milliliters per minute), V_m is the column volume (milliliters), ΔΦ is the gradient range (for example, 5–95% = 0.9), and S is a constant related to the molecular weight and is characteristic of each analyte. For the present discussion on scaling separations, we will not be changing the gradient range or the analytes, so ΔΦ and S will be constant and can be dropped for a simpler relationship:

With isocratic separations, the separation factor, α, is an expression of the peak spacing of two peaks with k values of k₁ and k₂:

As long as we do not change the chemistry of the system (mobile phase composition or column chemistry) or the column temperature, α will remain constant, as will the peak spacing. Isocratic k is calculated as follows:

where t_R is the retention time and t₀ is the column dead time. If t_R is changed by nonchemical or temperature means, t₀ will change in parallel, so k stays constant. This is why we can change the column length, the column diameter, or the flow rate and not affect k in isocratic separation. If k is constant, α will also remain constant. On the other hand, if we do something that changes k, we expect that α will change because it is rare for a variable that changes k for one compound to change k in exactly the same manner for all the other compounds in the separation. Thus, a change in the mobile phase or column temperature usually results in a change in peak spacing — in fact, we count on this effect as a tool for moving peaks relative to each other during method development.

A similar requirement of keeping α* constant holds for gradient separations, where α* is expressed as follows:

As with isocratic separation, chemical or temperature changes are not likely to change k*₁ and k*₂ in the same way, so these variables must be kept constant with gradients too. But with gradients, the added complication is that the variables on the right-hand side of equation 5 must also be kept constant, or they should be changed in a manner that allows α* to stay constant. Next, let's consider how this requirement influences the scaling of gradient methods.

Scaling a Simple Gradient

We'll start with a simple linear gradient of 5–95% acetonitrile over 20 min at 1 mL/min with a 150 mm × 4.6 mm, 5-μm particle diameter (d_p) column. Let's say that we want to get the same separation under ultrahigh-pressure liquid chromatography (UHPLC) conditions on a 1.8-μm d_p column with an internal diameter of 2.1 mm.

The first step is to find a column with approximately the same plate number. We can use equation 3 to help us. The new column length (L₂) should be (150 mm × 1.8 μm)/5 μm = 54 mm long. Because 54-mm columns aren't available, we can use any length between 41 mm (-25%) and 81 mm (+50%) and be within our -25% to +50% guidelines. I would probably choose a 50 mm × 2.1 mm column in the present case.

As with isocratic separations, with gradients it usually is a good idea to start with a flow rate that gives us the same linear velocity. We use the same technique we discussed last month:

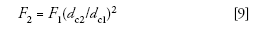
where F is the flow rate and d_c is the column internal diameter for columns 1 and 2. In the present case, the new flow rate should be 1 mL/min × (2.1 mm/4.6 mm)² = 0.208 mL/min; we'll round this to 0.2 mL/min for convenience. So, our new method will run on a 50 mm × 2.1 mm, 1.8-μm d_p column at 0.2 mL/min.

Let's check to see if we are done. Because we want k* to remain constant, we can restate equation 5 as follows:

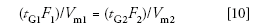
We can calculate each half of equation 10 for the proposed conditions and see how close we are. For the column volume, we'll use the approximation of (Ld_c²), because these are the only two variables that change between the columns. For the original conditions, (20 × 1)/(150 × 4.6²) = 0.00630, and for the proposed conditions, (20 × 0.2)/(50 × 2.1²) = 0.0181. The value for the new conditions is approximately three times too large. Because we've already defined the column size and the flow rate, the only variable we can adjust to fix this problem is the gradient time. If we reduce the gradient time to 7 min, the calculated value drops to 0.0635, which is close enough. So the gradient equivalent to the original conditions is a 7-min gradient at 0.2 mL/min on a 50 mm × 2.1 mm, 1.8-μm d_p column. This means that the switch from the high performance liquid chromatography (HPLC) to the UHPLC column reduces the run time by approximately threefold (20 min to 7 min).

But if we're running under UHPLC conditions, we can operate at higher pressures and because we're using sub-2-μm particles, flow rate will not affect N, so a higher flow rate may help to further speed the separation. We use the same equation to calculate the system pressure as we did last month for isocratic conditions:

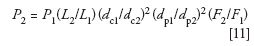
Where P₁ and P₂ are the original and new pressures, respectively; all the other variables were defined above. Let's assume that the original method generated a 2000 psi (140 bar) back pressure. For the proposed conditions, P₂ = 2000 × (50/150) × (4.6/2.1)² × (5/1.8)² × (0.2/1) ≈ 4935 psi. For UHPLC, we probably can tolerate twice this pressure, and the easiest way to do this is to double the flow rate so that a new pressure of ~9875 psi would be expected. Wait! We've changed the flow rate, so equation 5 tells us that this will change k*, and we may find a change in peak spacing — not something we want. So we need to make another compensating change using equation 5 as a guideline. If we double F, we can reduce t_G by the same factor of two and k* will stay constant. Now our method would be a 3.5-min gradient at 0.4 mL/min on a 50 mm × 2.1 mm, 1.8-μm d_p column. This would reduce the original run time by approximately sixfold (20 min to 3.5 min).

What About Segmented Gradients?

The example above used a simple, one-segment linear gradient. Let's add a bit of complication and see how a two-segment gradient is handled. We'll use a 5–50% gradient in 10 min followed by a 50–95% gradient in 5 min, the other conditions (column and flow rate) are the same as before. Let's scale this to the same 2.1-mm i.d., 1.8-μm d_p column. The same column, flow rate, and pressure scaling results will be obtained: scale to a 50 mm × 2.1 mm column operated at 0.2 mL/min resulting in a back pressure of ~4935 psi.

When it comes to scaling the remaining gradient conditions, we proceed in the same manner as with a single-segment gradient, but we need to treat each segment as a separate gradient. Thus, we have two gradients to consider: 5–50%/10 min and 50–95%/5 min. Because we're not changing the gradient range, we can scale the gradient times so that equation 10 is balanced. For the first segment, the original conditions give (10 × 1)/(150 × 4.6²) = 0.00315 and the proposed conditions give (10 × 0.2)/(50 × 2.1²) = 0.00907, approximately three times too large. A change in the gradient time to 3.5 min gives a value of 0.00317. For the second segment, our original gives (5 × 1)/(150 × 4.6²) = 0.00158. We would expect the same gradient time ratio adjustment to hold for the second segment as for the first, so we can use it in our trial calculation. So we'll use (3.5/10) × 5 min = 1.75 min, rounded to 1.7 min for convenience: (1.7 × 0.2)/(50 × 2.1²) = 0.00154, which is close enough. Now our new method will be 5–50% in 3.5 min followed by 50–95% in 1.7 min at 0.2 mL/min on a 50 mm × 2.1 mm, 1.8-μm d_p column.

If we want to increase the flow rate to accommodate the higher pressure capability of a UHPLC system, we use the same technique as discussed above with equation 11, but remember to adjust the gradient time of each segment by a factor of two using equation 5 if you change the flow rate by a factor of two.

Don't Forget to Adjust the Injection Volume

One thing I didn't mention in last month's discussion of isocratic scaling is the potential need to adjust the injection volume when the column size is changed. The same rules hold for both isocratic and gradient conditions. As a general rule, the injection volume should be adjusted in proportion to the column volume. We can use the following relationship as a guideline:

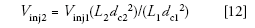
Where V_inj1 and V_inj2 are the injection volumes of the original and new conditions, respectively. If our original method used a 25-μL injection, the new method should use an injection of (25 × 50 × 2.1²)/(150 × 4.6²) = 1.75 μL, which we can round to 2 μL.

Often the injection volume for the original method may not have been optimized, so although equation 12 gives the proper scaling, I suggest using an empirical test to determine the injection volume of the new method. I would try the calculated volume plus additional injections at twice and half the recommended volume. In the present case, I would try injections of 1, 2, and 4 (or 5) μL and observe the chromatogram. If there is no unacceptable degradation of the chromatogram, especially in the first part of the run, it is likely that the injection volume can be tolerated. I like to have a safety margin in my conditions, so the injection volume should not be so large that it is right on the edge of failure. This can be achieved by allowing a safety margin of 50–100%. For example, if in the above example, 5 μL still looked OK, try 8 or 10 μL. If these injection volumes are OK, 5 μL is safe.

Further Cautions

As you can see from the discussion above, when the number of segments in the gradient increases, the work in calculating new conditions increases because each gradient segment must be scaled individually. What happens when there is a curved (convex or concave) gradient? I recommend strongly against using this type of gradient, because they are hard to reproduce from one instrument to another, and very difficult to scale. I don't know of any way to scale such gradients, because they are essentially composed of an infinite number of very small segments with different slopes. So, if you have an existing curved gradient that needs to be scaled, use the single-segment rules and hope that the new gradient works.

Those of you who are familiar with gradients will notice that I ignored the instrument dwell volume (gradient delay volume) in this discussion. The new dwell volume requirements can be calculated if the dwell time (the time to wash out the dwell volume) is considered as a gradient segment. The complication is that the dwell volume is seldom adjustable. Usually when you are moving a method from a conventional column to a small-volume or UHPLC column, you also will be moving from a conventional LC system to a UHPLC or a newer LC system, either of which is likely to have a reduced dwell volume. Dwell volume differences are most likely to affect the peak spacing for peaks eluted early in the gradient, so watch for such changes when the method is scaled. You may need to adjust the initial conditions to compensate for such changes. Dwell volume, of course, is not important in isocratic methods.

Finally, as with the isocratic examples last month, gradient calculations are tedious. You can simplify the process by using one of the on-line calculators to do the work for you. Some of these also calculate scaling of the injection volume and take dwell volume into account. Search the internet for "HPLC method transfer calculator" and you'll find several to choose from. I suggest that you try several and settle on the one that seems like the easiest for you.

References

(1) J.W. Dolan, LCGC North Am. 32(2), 98–102 (2014).

John W. Dolan "LC Troubleshooting" Editor John Dolan has been writing "LC Troubleshooting" for LCGC for more than 30 years. One of the industry's most respected professionals, John is currently the Vice President of and a principal instructor for LC Resources in Walnut Creek, California. He is also a member of LCGC's editorial advisory board. Direct correspondence about this column via e-mail to John.Dolan@LCResources.com

John W. Dolan