Go With the Flow: Thinking About Carrier Gas Flow in GC
LCGC North America
Successful GC analysis requires careful control of carrier gas. Here, we explain how to measure and control flow rate, use constant pressure vs. constant flow, and more.
Mobile phase flow is critically important, and must be carefully controlled. In this installment of “GC Connections,” we discuss carrier gas flow and its importance in successful gas chromatographic analysis. We will begin with a short review of fundamental theories showing why flow is important, and move into a discussion of how flow rate is measured and controlled on modern instruments. Finally, we will discuss the effect of flow-related parameters, including carrier gas choice, the difference between constant pressure and constant flow modes, and some new thinking about flow rate optimization. We conclude with some takeaways that should assist gas chromatographers with effective carrier gas management.
When a sample is injected into a column in any mode of chromatography, its molecules spend time in one of two phases, the stationary phase or the mobile phase, as illustrated in the very familiar equation 1.
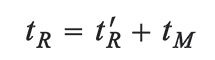
[1]
As we all know, tR represents the total retention time, t’R represents the adjusted retention time, or the time spent sorbed (not moving) in the stationary phase, and tM represents the holdup time, the time spent moving in the mobile phase, also defined as the time required for an unretained substance to traverse the column. The carrier gas flow rate obviously has a very strong impact on tM. We are also very familiar with equation 2, which represents the retention factor.
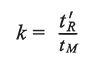
[2]
Most experts in method development will suggest that the best separations occur when k is between about 2 and about 10. The lower limit allows enough total time in the column for effective separation. The upper limit comes from a diminishing return for better resolution as retention times get very long. As k gets smaller, the importance of tM (flow) becomes larger. At k = 2, the analyte spends one third of the retention time in the mobile phase. At k = 10, it spends about 9% (1/11) of the retention time in the mobile phase. In any case, precise flow control is important for retention times, retention factors, and all of the calculations that we base on them to be reproducible.
Equation 3 relates the gas holdup time to the average column volumetric flow rate.
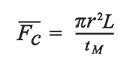
[3]
The numerator of equation 3 represents the total volume of the column (think of it as a long skinny cylinder). Note that the stationary phase film thickness is not considered; it usually does not contribute much to the total volume inside the fused-silica tube. Also, note that this is an average flow rate. In contrast to high performance liquid chromatography (HPLC), in which the liquid mobile phase is essentially non-compressible, the gas mobile phase in gas chromatography (GC) is compressible. According to Boyle’s Law, pressure and volume are inversely proportional. As the gas moves from the higher- pressure column head to the lower pressure outlet, it expands, increasing in volume. Thus, the volumetric flow rate calculated by equation 3 is the average volumetric flow rate and it is usually expressed in units of cm3/min or mL/min.
A related term, average linear gas velocity, which is simply the average speed (usually expressed in cm/s) of the gas flowing through the column, is given in equation 4.
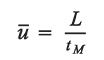
[4]
As we saw above, the gas is expanding as it moves along the column. Since the column constricts the gas flow, the velocity of the gas must also increase as it moves down the column. The average linear gas velocity is most commonly used to examine the effect of flow on peak width and the construction of “van Deemter” plots, the classical method for optimizing the flow rate, discussed below. In a previous “GC Connections” column, Hinshaw provided a detailed description of the relationships between carrier gas flow and velocity (1).
There are several variables that determine the gas holdup time and some complexity in how gas molecules move through the column. Blumberg has provided a detailed description and equations describing the fundamental basis of the gas holdup time (2). The relationships involved are surprisingly complex, but can be summarized by stating that the gas holdup time is a function of column dimensions (length and inside diameter), the inlet and outlet pressures and the viscosity (resistance to flow) of the carrier gas. The viscosity of a gas is a function of temperature, and increases as temperature increases. This is the opposite behavior seen with liquids, where the viscosity decreases with increasing temperature. Some instruments, data systems, and computer simulation programs use equations based on the these relationships to determine gas holdup times from the known variables, lessening the need to physically measure tM. Finally, remember that the calculated flow rate and linear gas velocity are average values, and they assume isothermal operation throughout the run.
Measuring the Gas Holdup Time
In a recent “GC Connections” column, Hinshaw suggested keeping a manual syringe and a butane lighter handy as part of the supplies kit for any gas chromatograph (3). Injecting butane from a lighter serves two very important purposes. First, butane is not retained on most capillary columns at most temperatures used in GC, so it can be used as the analyte to directly measure the gas holdup time, tM. Second, the shape of the butane peak, injected following a column change or other maintenance, provides a confirmation that the column is connected properly and the connections are leak-free. If the butane peak exhibits tailing or a poor peak shape, then there is likely a leak or a problem with the connections. Beware that butane may be retained on some thick film columns, so methane is preferred when using them.
The technique of injecting butane was developed in times when most GC was performed on packed column instruments, with manual injection and a manually operated chart recorder as the data system. Classically, injecting butane or methane (we used Bunsen burner gas collected from the laboratory gas jets or in a small lecture bottle) requires training that may not be commonly known today. Also, if using an autosampler, the autosampler may need to be dismounted from the instrument and a manual method set up in the data system. This makes injecting butane, while it may be a necessity, potentially problematic. Interestingly, this problem was addressed as early as 1959 by Peterson and Hirsch, who developed an equation for determining the holdup time from the retention times of three homologous fatty acid methyl esters (4). As recently as 2013, Wu and associates provided an excellent review and comparison of many techniques that have been used to determine gas holdup times. They correctly pointed out that all substances are retained to some degree, and that variations in gas holdup time measurements may lead to variations in calculations that are based on them, including retention factors, partition coefficients, and retention indexes (5).
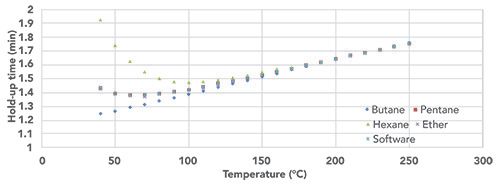
Figure 1 shows the retention time for several analytes injected neat, measured on a common capillary column under isothermal conditions at temperatures ranging from 40 to 250 oC. Butane was injected by drawing about 5 µL of vapor from a lighter (just hold down the handle on the lighter while inserting the syringe needle and moving the plunger). The liquids (pentane, hexane, and ether) were injected by placing about 100 µL of each into a standard 2 mL vial, sealing the vial and injecting 5 µL of the headspace vapor, using a standard syringe and the autosampler. The tM values were also calculated using equation 5 and retention factors for tetradecane provided by the data system.
Figure 1: Gas hold-up time versus temperature for several analytes. The figure shows data plotted for butane, pentane, hexane, ether, and software.
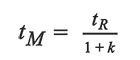
[5]
With all variables other than temperature held constant, the retention time of an unretained substance, the gas holdup time, should increase with temperature, related to the carrier gas viscosity. Viscosity of a gas is related to temperature by an exponential relationship that can approximate to a linear relationship in the temperature ranges used in GC (6). In short, if a substance is unretained, the relationship between retention time and temperature should be linear with a positive slope. In Figure 1, this is seen at all temperatures for butane, and at temperatures above about 150 oC for pentane and diethyl ether. The calculated value also almost exactly overlapped with the butane results over the entire range, indicating agreement between the measured and calculated values. As the temperature decreases, however, the pentane, hexane, and diethyl ether become clearly retained, eventually exhibiting the expected behavior with the retention time becoming longer as the temperature is lowered. When measuring holdup time, take care to ensure a symmetrical peak; if the peak is asymmetrical, the retention time will not be accurate.
Measuring Flow Rates
As we have seen above, the average carrier gas flow rate can be calculated by measuring the gas holdup time, tM. However, the average flow rate is not, by itself, a very useful measure for most practical situations. For example, the split ratio, calculated as the split vent flow divided by the column flow, requires the column flow to be measured at the inlet to be accurate. In packed-column GC, where column flow rates were much larger than with today’s capillary columns, the average and inlet flow rates are calculated from the measured outlet flow rate by applying appropriate correction factors (7).
Also in packed column GC, the column flow rate is most easily measured by attaching a soap-film flowmeter, as seen in Figure 2, to the column outlet. These are inexpensive, and are operated by generating a soap bubble from the bulb at the bottom, and measuring the time required for 1, 10, or 100 mL of gas to flow from the column. This time is converted to a flow rate in mL/min. Soap film flow meters can also to measure split vent flow, as seen in Figure 2, and detector gas flow rates in capillary column systems. Today, fully electronic flow meters are available from a number of vendors. An electronic flow meter and an electronic leak detector are both must-haves in a modern GC laboratory.
Figure 2: Soap film flowmeter connected to the split vent of a classical pneumatic gas chromatograph.

Capillary column flow rates are more difficult to measure than packed column rates, as they are generally much lower, typically on the order of 1–2 mL/min and the measurement is often needed when the system is running. It is not wise to place the inlet of a flowmeter into a heated and running flame ionization detector (FID)! It is impossible to directly measure the outlet flow rate on an mass selective detector (MSD). Today’s electronically controlled systems calculate column flow rates automatically, using the known column dimensions, choice of carrier gas, inlet and outlet pressure, and temperature and relationships, such as those discussed in this article. Users should be certain that the column dimensions are accurately entered into the data system, and should double check that they are correct if the system enters them automatically.
Constant Pressure vs. Constant Flow
Prior to the advent of the solid-state electronic pneumatic controls, used in most new GC instruments today, nearly all commercially available GC systems for both packed and capillary columns operated at constant column head pressure. For packed column systems, this allowed very simple pneumatics, essentially just a flow controller and a pressure regulator. Today’s capillary column systems with electronically controlled pneumatics can operate in two modes: constant pressure and constant flow. Shortly after the advent of electronic control of the pneumatic systems in gas chromatographs, Blumberg, Wilson, and Klee compared column performance characteristics in constant pressure and constant flow with temperature programming (8). They noted that there is little difference in overall column performance between the two options, so other considerations determine the choice of mode.
In constant pressure operation, the column head pressure is constant throughout the run. This is the more common operating condition and is seen is most of the GC literature, especially papers and methods more than 10 years old and in many compendial methods. In a temperature-programmed run the carrier gas viscosity will increase with the temperature, causing a decrease in the flow rate as the run proceeds. This decrease is partly offset by an increase in volume (Charles’ Law) as the temperature increases. Overall, however, in most cases, the volumetric flow rate will decrease as temperature increases in a temperature-programmed run.
In constant flow operation, the electronic flow controller increases the head pressure as the temperature is increased, to maintain a constant flow rate. Figure 3 shows chromatograms of the same sample, run under the same temperature program and same initial column head pressure. The chromatogram on top was run in constant pressure mode and the chromatogram on the bottom was run under constant flow mode. Note that the retention times are slightly shorter in the constant flow chromatogram, however, in agreement with Blumberg and colleagues, the peak widths and spacing are about the same. Not surprisingly, the effect on retention time is greater for the later eluting peaks.
Figure 3: Constant pressure and constant flow chromatograms of a column test mixture.
An article on ChromAcademy, LCGC‘s learning platform, describes fundamentals of setting up gas flows in GC and discusses constant pressure and constant flow (9). Some ideas to consider when choosing which mode to use include:
- Detector performance. Mass-sensitive detectors such as FID and MSD may perform better with constant flow.
- Translating methods. Constant pressure may be preferred when a method is translated between differing column dimensions or stationary phases.
- Speed. Constant flow methods will often be slightly faster than constant pressure.
- Lower temperature. Later eluted peaks will be eluted at lower temperature in constant flow mode.
- Electronic pneumatic control-based techniques for ensuring system-to-system and laboratory-to-laboratory reproducibility, such as retention time locking and method translation often require constant flow mode.
When optimizing, adapting, or attempting to repeat a method from the literature, it is important to note which mode was used for the original method, and either ensure that you are using the same mode, or be prepared to perform additional optimizing.
Choosing a Carrier Gas
Carrier gases for GC must meet several requirements to be useful. The carrier gas must be inert, safe, dry, highly pure, suitable for the detector, and inexpensive. Helium, which readily meets all of these characteristics, has been the carrier gas of choice for most capillary GC work, especially gas chromatography–mass spectrometry (GC–MS), for decades, used in over 90% of recent research articles describing GC methods. Recently, however, several incidents of regional and global shortages have caused helium to be expensive or unavailable at times. As helium becomes less available, hydrogen and nitrogen emerge as leading alternatives, because they meet all of the necessary characteristics. Table I provides a brief comparison of the three gases.
As seen in Table I, each gas has benefits and difficulties. Helium has been the gas of choice, because with moderate cost, it is highly pure, inert, and fast, gives high resolution, and is compatible with nearly all detectors, including mass spectrometers. With the rising cost of helium, many experts are recommending changing to hydrogen, which, given its lower molecular weight and viscosity than helium, can generally provide faster separations with similar or improved resolution. If obtained from cylinders, hydrogen is also moderately expensive, offers better resolution, and is often the carrier gas of choice when extremely narrow bore columns (0.1 mm inside diameter) are used, and for fast GC. Many laboratories are now using hydrogen generators, which offer high purity hydrogen, but with high up-front capital cost, ongoing maintenance, and potential downtime if there is a failure of the generator. If you consider using hydrogen, be sure to consult with your instrument manufacturer, because modifications or adjustments may be needed in order to prevent hydrogen form leaking into the laboratory.
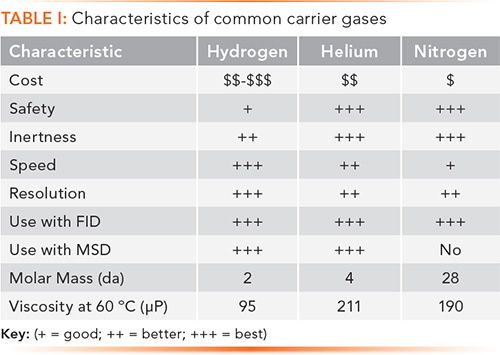
Most experts do not recommend nitrogen for capillary column work. Although the cost is low, it is compatible with most detectors, and the viscosity is similar to helium, the high molecular weight leads to lower resolution through increased diffusion rates for analyte molecules in the gas phase. Furthermore, nitrogen is detected within the mass range for most benchtop mass spectrometers, so it is not generally compatible with MS detection. In December 2018, a helium shortage struck our facility, and for several months we were not able to purchase helium cylinders at any price. Lacking enough capacity in our hydrogen generators to run carrier gas as well as FID gas, we decided to switch to nitrogen and take our chances. Figure 4 shows overlaid FID chromatograms of a simple (C6-C20 n-alkanes) hydrocarbon mixture, using helium (black) and nitrogen (green) with a simple linear temperature program, a split injection, and a common capillary column operated in constant pressure mode.
Figure 4: Overlaid chromatograms of an
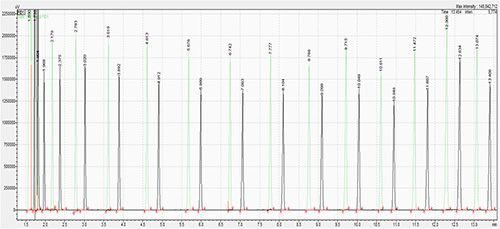
As seen in Figure 4, the two chromatograms are remarkably similar, with the main difference being slightly shorter retention times for nitrogen, due to the lower viscosity of nitrogen versus helium, as seen in Table I. The peak widths and separation numbers (a measure of the spacing between the peaks) are nearly identical. This is not the result that most chromatographers would expect; we would have expected the nitrogen separation to exhibit significantly poorer performance. Remember that most of our common understanding about carrier gas flow and characteristics is based on isothermal analysis. Changing the temperature as the run proceeds impacts nearly every physical parameter that affects transport of molecules along the column. We addressed the overall peak widths seen in both chromatograms in a previous “GC Connections” column (10). In short, Figure 4 leads to the recommendation that, if using helium is becoming problematic, nitrogen should be considered, as it is very abundant, inexpensive to obtain, may give adequate separation performance, and will not damage the instrumentation if it does not work. With more helium shortages looming, there is already a lot of discussion and advertising around alternatives. When choosing an alternative to helium, chromatographers should carefully evaluate both hydrogen and nitrogen, and make the best choice for their own laboratory.
New Directions
Classically, most gas chromatographers optimize the flow rate for a separation by trial and error, by making a Van Deemter or Golay plot of the height equivalent to a theoretical plate (H) vs. the average mobile phase velocity or the average flow rate, or by doing nothing and simply setting a column head pressure they have “always used.” Trial and error and doing nothing are obvious approaches and numerous references that discuss making van Deemter plots are available elsewhere (11). All three of these approaches have limitations; for trial and error and doing nothing, the limitations are obvious. Van Deemter and Golay plots have been heavily used for flow optimization since the early days of GC, and therein lies the limitation. The theory, while illustrative, was developed for the limited conditions of the inlet and outlet pressure ratio near unity and constant temperature (12,13). Quoting Golay, the equations “are applicable to columns of uniform cross-sections in which the input to exit pressure ratio is near unity.” This means that the commonly used Golay equation is only applicable in cases where the pressure drop between the column inlet and outlet is very small. In today’s capillary GC, this places a potentially severe limitation on the actual utility of the plots, given the common use of small-diameter columns and large pressure drops. It is generally not applicable to larger pressure drops and vacuum outlet detectors, such as MSD, common in today’s capillary GC. The Golay equation is also not applicable at all to temperature programming. In a book and a book chapter, Blumberg addresses this problem in detail, but a simplified optimization process to replace van Deemter and Golay plots is still eluding most chromatographers (14,15). Be careful when using classical van Deemter or Golay equation plots to select an optimum flow rate; they may not be applicable to your situation.
Conclusions
Although measuring the carrier gas flow rate in GC is very straightforward, and optimizing it may be as simple as a trial- and-error approach, the underlying principles behind how the carrier gas flows through the column, and how this affects retention time and peak broadening, are not so simple and have been under discussion since the inception of GC. In thinking about carrier gas flow and gas holdup time, some lessons and takeaways become apparent:
- Given that the gas holdup time may be up to half of the time an analyte spends in the column, precise flow control is critical in capillary GC.
- Calculated flow rate and linear gas velocity are average values, and they assume isothermal operation throughout the run.
- When measuring gas holdup time (tM), take care to ensure a symmetrical peak; if the peak is asymmetrical, the retention time will not be accurate, and the asymmetry indicates a problem with the inlet or gas flows.
- An electronic flow meter and an electronic leak detector are both must-haves in a modern GC laboratory.
- Be certain that the column dimensions are accurately entered into the data system, or double check that they are correct if the system enters them automatically.
- When optimizing, adapting, or attempting to repeat a method from the literature, note which mode (constant pressure or constant flow) was used for the original method, and either ensure that you are using the same mode, or be prepared to perform additional optimizing.
- When choosing an alternative to helium, chromatographers should carefully evaluate both hydrogen and nitrogen, and make the best choice for their own laboratories.
- If you consider using hydrogen, be sure to consult with your instrument manufacturer, as modifications or adjustments may be needed in order to prevent hydrogen form leaking into the laboratory.
- Be careful in using classical van Deemter or Golay equation plots to select an optimum flow rate; they may not be applicable to your situation.
References
- J.V. Hinshaw, LCGC North Am.34(3), 198–205 (2016).
- L.M. Blumberg, in Gas Chromatography, C.F. Poole (ed), (Elsevier, Amsterdam, The Netherlands, 2012), pp. 33–36.
- J.V. Hinshaw, LCGC North Am. 37(9), 676–683 (2019).
- M.L. Peterson and J. Hirsch, J. Lipid Res. 1, 132–134. (1959)
- L. Wu, M. Chen, Y. Chen,and Q. Lia, J. Chromatogr. A 1297, 196–203 (2013).
- L.S. Ettre, Chromatographia 18(5), 243–248 (1984).
- H.M. McNair, J.M. Miller, and N.H. Snow, Basic Gas Chromatography (John Wiley and Sons, New York, New York, 3rd Ed., 2019), 234–235.
- L.M. Blumberg, W.H. Wilson, and M.S. Klee, J. Chromatogr. A842, 15–28 (1999).
- “Theory and Instrumentation of GC Gas Supply and Pressure Control” ChromAcademy. http://www.chromacedemy.com. (accessed January, 2020).
- N.H. Snow, LCGC North Am. 37(7), 450–456 (2019).
- H.M. McNair, J.M. Miller, and N.H. Snow, Basic Gas Chromatography (John Wiley and Sons, New York, New York, 3rd Ed., 2019), 25–35.
- J.J.J. van Deemter, F.J. Zuiderweg, and A. Klinkenberg, Chem. Eng. Sci.5, 271–289 (1956).
- M.J.E. Golay, in Gas Chromatography, D.H. Desty, Ed. (Academic Press, New York, New York, 1958), 36–55.
- L.M. Blumberg, in Gas Chromatography, C.F. Poole (ed), (Elsevier, Amsterdam, The Netherlands, 2012), 19–79.
- L.M. Blumberg, Temperature Programmed Gas Chromatography (John Wiley and Sons, New York, New York, 2010).

Sean P. McCann is a graduate student in the Department of Chemistry and Biochemistry at Seton Hall University. He holds a BS degree in Chemistry and a BS degree in Forensic Science from the University of New Haven, CT.

Hetal Rana is a graduate student in the Department of Chemistry and Biochemistry at Seton Hall University. She holds a BS degree in Chemistry from Veer Narmad South Gujarat University in India.

Brittany A. Handzo is an analytical chemist at GQAS&T Pharma and Forensics Department, Bristol Byers Squibb, New Brunswick, NJ. She holds a BS degree in Chemistry from Fairleigh Dickinson University and a MS in Chemistry from Seton Hall University.

Nicholas H. Snow is the Founding Endowed Professor in the Department of Chemistry and Biochemistry at Seton Hall University, and an Adjunct Professor of Medical Science. During his 30 years as a chromatographer, he has published more than 70 refereed articles and book chapters and has given more than 200 presentations and short courses. He is interested in the fundamentals and applications of separation science, especially gas chromatography, sampling, and sample preparation for chemical analysis.His research group is very active, with ongoing projects using GC, GC–MS, two-dimensional GC, and extraction methods including headspace, liquid–liquid extraction, and solid-phase microextraction. Direct correspondence to: LCGCedit@mmhgroup.com

Fundamentals of Benchtop GC–MS Data Analysis and Terminology
April 5th 2025In this installment, we will review the fundamental terminology and data analysis principles in benchtop GC–MS. We will compare the three modes of analysis—full scan, extracted ion chromatograms, and selected ion monitoring—and see how each is used for quantitative and quantitative analysis.
Study Examines Impact of Zwitterionic Liquid Structures on Volatile Carboxylic Acid Separation in GC
March 28th 2025Iowa State University researchers evaluated imidazolium-based ZILs with sulfonate and triflimide anions to understand the influence of ZILs’ chemical structures on polar analyte separation.















