Facts and Legends About Columns Packed with Sub-3-µm Core-Shell Particles

Pellicular particles were invented by Csaba Horváth more than 50 years ago to prepare columns providing efficient high performance liquid chromatography (HPLC) separations of high-molecular-weight compounds of biological origin. However, pellicular particles met with stiff competition from packing materials made with high-quality, fine, fully porous particles. As a result, pellicular particles encountered little commercial success and drifted into oblivion until, in 2007, they were resuscitated as the modern sub-3-µm core–shell particles. This article reports on the performance of columns packed with these new pellicular particles, the kinetic mechanisms that underlie their exceptional performance in the separation of low-molecular-weight compounds, and the reasons for their current success. We demonstrate how a detailed investigation of the band-broadening phenomena taking place in these columns has led us to challenge beliefs that are both erroneous and widespread throughout the HPLC community.

Pellicular particles were invented and pioneered by Horváth in the late 1960s (1–3) with the specific purpose of preparing columns that could provide highly efficient high performance liquid chromatography (HPLC) separations of the constituents of high molecular weight compounds of biological origin. These particles are made of a solid silica core surrounded by a shell of porous silica, a design that leads to short average diffusion paths of analytes across the particles, and, therefore, a low solid-liquid mass transfer resistance (Cu) contribution to the van Deemter plate height equation of the column if diffusion is sluggish (8). Particles of this type were commercialized by Kirkland, who prepared large, 50-µm particles in the 1970s (4,5) and 5-µm particles in the 1990s (6), with the intent to pack columns that allowed for high-efficiency separations of conventional compounds. Those columns were not successful. Apparently, the main reason was the rapid development between 1970 and 1990 of small, conventional, fully porous particles that provided high-efficiency columns (9). In the early 2000s, 1.7-µm fully porous particles became the best performing packing material, providing columns with height equivalent to a theoretical plate (HETP) terms as low as 3.3 µm (10).

In 2007, a brand of 2.7-µm superficially porous particles emerged on the market. These particles were made of 1.7-µm solid silica cores and were covered with a 0.5-µm porous shell. This design solved the problem of the low loading capacity of columns packed with the large, early pellicular particles because 75% of the volume of these particles is porous (11). Most importantly, 4.6-mm i.d. columns packed with these new particles provide efficiencies equivalent to those of columns packed with 1.7-µm classical particles, with the additional advantage of operating at back pressures that are two-to-three times lower (12). Therefore, these particles made it possible to achieve ultrahigh column performance using the standard 400-bar instruments, requiring only that some low-cost modifications be made to the instrument (such as using a smaller detection cell volume and connectors with smaller internal diameters) (13).

In this article, the mass transfer mechanism in this new generation of columns packed with core–shell particles is analyzed for low-molecular-weight compounds. The relative contributions of the longitudinal diffusion (B/u), the eddy dispersion (A), and Cu terms of the van Deemter equation are analyzed and compared to those of columns packed with standard fully porous particles. Chromatographic myths or legends such as the relative impact of the B/u and Cu HETP terms on the overall column performance or the influence of the particle-size distribution (PSD) on column performance are discussed and revisited.

Mass Transfer in Packed LC Columns

Let us briefly recall the basic equations that provide the values of the contributions to band broadening because of the B, A, and C terms of the van Deemter equation in columns packed with core–shell particles. All complementary details required can be found elsewhere (7,14). The reference linear velocity, u, used in this work is the average interstitial linear velocity. The corresponding reduced velocity, υ, is defined by

where d_p is the average particle size and D_m is the bulk molecular diffusion coefficient. Note that the average particle diameter used in this work was simply the "commercial" average particle diameter (2.5, 2.6, and 3.0 µm for Luna [Phenomenex], Kinetex [Phenomenex], and Atlantis [Waters] particles, respectively).

Longitudinal Diffusion Term (B/u)

The reduced longitudinal diffusion HETP term, h_long, accounts for the band broadening contribution because of molecular diffusion in the migrating band. It is caused by the concentration gradient between the band center and its edges and is best written as follows (15):

In this equation, the external obstruction factor, γ_e, is 0.65 for 100 mm × 4.6 mm columns packed with 1.9-µm nonporous silica spheres with a narrow PSD (RSD around 5%) and a bed voidage or external porosity, ε_e, of 42% (16). The variable ρ is the ratio of the solid core to the particle diameter and Ω is the ratio of the sample diffusivity in the porous shell to that in the bulk phase. B is the longitudinal diffusion coefficient. It is derived from a series of peak parking experiments (17). If the average size of the mesopores in the porous shell is around 100 Å, × typically ranges from 0.01 (for nonretained proteins) to 0.15 (for nonretained small molecules) and up to 1.50 (for moderately retained compounds because of surface diffusion) (14).

Solid–Liquid Mass Transfer Resistance Term (Cu)

This HETP term, h_{liquid–solid}, accounts for band broadening because of the velocity difference between the moving eluent and the stationary phase. If we consider spherical particles, this term is written as follows (18):

where k₁ is the zone retention factor (~0.2 and 3.0 for compounds with retention factors of 0 and 2, respectively). C is the solid–liquid mass transfer coefficient. Typically, ε ranges between 0.60 and 0.75 for the different brands of core–shell particles currently available in the field.

Eddy Dispersion Term (A)

The eddy dispersion term, h_eddy, accounts for all the sources of uneven flow velocity distribution in the interstitial mobile phase throughout the cross-section of the column. This contribution may be indirectly assessed by subtracting the previous HETP contributions from the overall reduced plate height, h, which is derived from the first and second central moments of the band after correction of these moments for the extracolumn volume contributions because of the band dispersion along the instrument channels. So, the actual value of the reduced HETP is

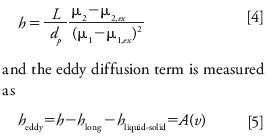
A(υ) is the eddy dispersion HETP term. In contrast with what is generally held, it depends, a priori, on the linear velocity (21).

The determination of the column efficiency from the statistical moments of the elution band is the only correct method for assessing column efficiency. It takes the contribution of peak tailing into proper account. The classical method based on the bandwidth at any fractional peak height is highly inaccurate. Thus, all measurements of HETP values throughout this paper were performed by the moment method (19,20).

Interesting Legends Currently Propagated in HPLC

Several legends are commonly accepted as fundamental concepts in the popular chromatographic literature. Such legends also can be found in part of the scientific literature as well as in brochures provided by column manufacturers. When it became necessary to support or justify the exceptional performance of columns packed with the new generation of sub-3-µm core–shell particles, legends multiplied, as we will demonstrate.

Although important, the common Legend 1 is implied. The contribution of the axial diffusion term to the optimum column HETP is systematically neglected in the scientific literature, although this fact is rarely explicitly mentioned. Yet, the B term usually accounts for nearly 25% of the total band broadening of retained compounds on reversed-phase liquid chromatography columns used at optimum velocity and packed with fully porous particles. Because the volume occupied by the solid cores of shell particles represents about (1 – ε_e)ρ³ ~(1 – 0.4) × 0.73³ , or ~25% of the column volume, one can anticipate a smaller B term and greater column efficiency for columns packed with core–shell particles than for those packed with conventional particles. The measurement of the B term and its quantitative contribution to the overall plate height (at a constant ratio of shell diffusivity to bulk sample diffusion) needs to be revisited.

Legend 2 concerns the impact that an average diffusion pathlength, shorter through core–shell than through fully porous particles, may have on improving the efficiency of columns packed with the former for separations of small molecules. All advertising brochures released for core–shell particles by Agilent, AMT, Phenomenex, Supelco, and Thermo Scientific elaborate on the same theme, summarized as: "This shorter diffusion path allows for faster mass transfer. The result is less band broadening for higher peak efficiency comparable to or better than sub-2-µm porous particles." A case in point is provided in Figure 1. Analysts are tempted to believe that the reduction of the solid–liquid mass transfer coefficient C for core–shell particles explains that plate counts are higher than those seen with columns packed with conventional particles. Although it is true that the average diffusion pathlength is shortened in core–shell particles, this does not prove that the faster mass transfer across these particles is responsible for the higher peak efficiencies observed. Only a quantitative and accurate measurement of the coefficient C could clarify this question.

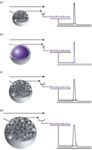
Figure 1: Brochure illustration claiming the importance of the reduction of the average diffusion on kinetic performance: (a) sub-2-µm fully porous, (b) 2.6-µm Kinetex core–shell, (c) 3-µm fully porous, and (d) 5-µm fully porous particles. Adapted from Phenomenex, Inc.

Legend 3 deals with the eddy diffusion term (A) for small molecules. In most textbooks, scientific papers (8), and company reports, this term is assumed to be independent of the eluent velocity, which is in profound contradiction with the general theory of eddy diffusion of Giddings (21). The Giddings theory is 40 years old and, strangely enough, is as universally ignored as its author is well-known and respected. Therefore, it is often believed that the column HETP increases at high velocities because of the C term, for example, to mass transfer resistance between the moving eluent and the stationary phase. Again, no definitive conclusion can be drawn until the coefficients A and C are accurately measured as a function of the reduced velocity for chromatographic columns.

Figure 2: Brochure illustration claiming the importance of the tightness of the particle size distribution on the band broadening taking place in the interstitial moving eluent. Left: Core–shell particles; right: conventional fully porous particles. This illustration suggests an increase of the packing order with decreasing the RSD of the PSD. Adapted from Phenomenex, Inc.

Finally, Legend 4, which is widespread in the chromatographic literature, states that narrow RSDs of the PSD of packing materials provide columns having a small A term and permit less sample dispersion in the interstitial eluent (33). Monosized particles are believed to be the Holy Grail of packing materials, generating more ordered packed beds than those made of particles with wide PSDs. Figure 2 illustrates this myth, which can be found in almost all advertising brochures for core–shell particles (for example, Halo [Advanced Materials Technology], Poroshell [Agilent Technologies], Accucore [Thermo Fisher Scientific], and SunShell [Chromex Scientific]), which have a narrower PSD (RSD ~5%) than that of conventional fully porous particles (~20%). Analysts are encouraged to believe that the exceptional performance of columns packed with core–shell particles is directly linked to the tightness of their PSD. Yet, there is no experimental proof and no theoretical consideration to support this statement.

The Solid Facts That Are Reluctantly Promoted

Legend 1 and the Impact of the B Term

Figure 3 shows the impact of the ratio of the solid core and the packed particle diameters (ρ) on the values of the B coefficient measured for different ratios of shell diffusivity to bulk diffusion (Ω) in reversed-phase LC. The B coefficient is minimal for nonporous particles (such as 1.9-µm nonporous silica) and has a value of approximately 1.3, that is, twice the external obstruction factor γ_e. If the particles are fully porous (for example, 3-µm Atlantis particles), B is maximized. For superficially porous particles (such as 2.6-µm Kinetex particles), the B values are intermediate. For instance, for a retained compound in reversed-phase LC with a typical ratio of Ω = 1.25, the axial diffusion coefficient B increases from 1.3 for nonporous particles to 4.2 for core–shell (ρ = 0.73) and 6.2 for fully porous (ρ = 0) particles.

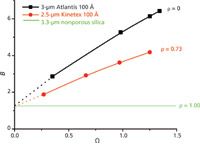
Figure 3: Experimental reduced longitudinal diffusion coefficients (B) plotted as a function of the ratio Ω of the sample diffusivity in the porous particle to that in the bulk. Comparison between core–shell and fully porous particles. Both curves are converging toward twice the external obstruction factor (~2 × 0.65 = 1.3 as expected for nonporous particles) when Ω tends towards zero. Most importantly, note the large impact of the ratio of the core-to-particle diameter, ρ, to the diminution of the B coefficient at constant values of Ω.

The average minimum reduced HETP of six 100 mm × 4.6 mm columns packed with the same batch of 2.6-µm Kinetex particles was found to be 1.7 for naphthalene (k = 3.0, Ω = 1.41) (22). The optimum reduced linear velocity was close to 10 in each case (optimum flow rate around 2.5 mL/min). At the same reduced velocity, the reduced HETP (not necessarily the minimum value) of 100 mm × 4.6 mm columns packed with 3.0-µm fully porous Atlantis particles was 2.1. The diminution of the reduced B term by (6.6 – 4.5)/10 = 0.2 h unit (see Figure 3 and the corresponding B values for Ω = 1.41 at ρ = 0 and ρ = 0.73), contributes significantly (up to 50%) to the decrease in column plate height (from 2.1 to 1.7) when replacing fully porous with core–shell particles. Remarkably, the manufacturers of sub-3-µm core–shell particles rarely point out this fact. Obviously, the impact of a reduction of the B coefficient decreases continuously with increasing analysis speed beyond the optimal velocity, a domain where longitudinal diffusion becomes a secondary factor.

The optimum reduced velocity of columns packed with core–shell particles is markedly higher than that of columns packed with fully porous particles — nearly 50% higher. This is another consequence of the B term's being smaller for the former rather than the latter columns.

Legend 2 and the Impact of the C Term

The average C coefficient of the general reduced HETP equation was measured for the same series of six 100 mm × 4.6 mm columns packed with 2.6-µm Kinetex particles and for naphthalene. This coefficient depends on the external porosity of the column (ε_e = 0.40), the zone retention factor of the analyte (k₁= 3), the structural parameter of the Kinetex particles (ρ = 0.73), and the diffusivity of naphthalene across the porous shell (Ω = 1.4). According to equation 4, C = 0.0037. If the Kinetex particles were fully porous (for example, ε = 0), the C coefficient would have been nearly 2.3 times larger, with C = 0.0084. For small molecules, the largest reduced velocity that could be reached with conventional instruments is typically around υ_max = 20 (maximum flow rate of 4.5 mL/min with 4.6-mm i.d. columns). So, the largest decrease of the reduced plate height caused by the sole reduction of the solid–liquid mass transfer resistance term is (0.0084 – 0.0037)(20) = 0.09. At the optimum velocity (υ_opt = 10), this gain is smaller, approximately 0.05 h unit. In contrast to what is usually advertised for column technology, the exceptional kinetic performance of the new sub-3-µm core–shell particles has very little to do with the faster solid–liquid mass transfer.

We emphasize that this is valid for small molecules with molecular weights smaller than ~500 Da. In contrast, for high-molecular-weight compounds (5 kDa < MW < 500 kDa) the bulk diffusion coefficients is 5–50 times smaller than that of small molecules, and this conclusion could be different because, at the same flow rate, the reduced velocity would be 5–50 times larger. The molecular size of these proteins is typically between 30 and 110 Å. Then, considering widepore core–shell particles (with a pore size up to 300 Å), the reduced plate height would decrease by at least 0.25 to 2.5 h units, a significant improvement in column performance. This observation is consistent with the original work of Horváth, who designed superficially porous particles specifically to separate large proteins or biochemicals (1).

Legend 3 and the Impact of the A Term

Figures 4a and 4b compare the experimental contributions of the three main HETP contributions (longitudinal diffusion, eddy dispersion, and solid–liquid mass transfer) in two different 100 mm × 4.6 mm columns. Figure 4a corresponds to a column packed with fully porous particles (2.5-µm Luna C18, 100 Å) and a small retained sample (naphthalene), eluted with a mixture of acetonitrile and water (65:35, v/v). Figure 4b shows the same data for a column packed with core–shell particles (2.6-µm Kinetex C18, 100 Å). First, these figures confirm that the B term (green curve) is larger for fully porous than for superficially porous particles at a low reduced velocity of υ ≈ 0.5. Yet, the porous shell of Kinetex particles is very similar to the porous Luna C18 (2) in terms of analyte diffusivity because Ω values were measured at 1.41 (Kinetex) and 1.44 (Luna). The B terms are essentially different because of the presence of the solid cores, as previously discussed in Figure 3 and as predicted by equation 3.

Figure 4: Contributions of the longitudinal diffusion (green), eddy dispersion (red), and solid–liquid mass transfer resistance (blue) terms to the overall reduced plate height of 100 mm × 4.6 mm columns packed with 2.5-µm fully porous particles (left) and 2.6-µm core–shell particles (right). Analyte: naphthalene; mobile phase: 65:35 (v/v) acetonitrile–water; temperature = 297 K.

Second, at low velocities (0 < υ < 15), the data demonstrate that the eddy dispersion term (red curve) is not constant, which is consistent with Giddings's general theory of eddy dispersion and falsifies the widespread legend that assumes that A is constant.

Finally, and most importantly, a comparison of Figures 4a and 4b reveals that the lower reduced plate heights measured for the Kinetex columns arise because of a significantly smaller eddy dispersion term. At the highest reduced velocities that are experimentally accessible (υ ≈ 20), A is nearly constant and equal to 1.5 (for core–shell particles) or 2.5 (for fully porous particles), quite a significant difference that explains the superiority of the columns packed with core–shell particles over those packed with fully porous particles.

Legend 4 and the Impact of the PSD on the A Term

Column manufacturers are then correct to claim that the better performance of columns packed with sub-3-µm shell particles results from a decrease in the eddy dispersion term. What requires careful investigation is whether this reduction in the band broadening contribution, which is caused by faster mass transfer in the interstitial mobile phase, originates from the fact that the PSD of core–shell particles (RSD ~3–6%) is narrower than that of conventional particles (RSD of ~10–30%). A wealth of literature has been devoted to this scientific debate (23–27). The literature unambiguously concludes that the RSD of the PSD has no measurable impact on column efficiency unless the RSD exceeds 50%. More recently, a profound theoretical analysis (28) based on the reconstruction of beds packed with particles having narrow PSDs (RSD = 3.4%, like the core–shell 2.6-µm Kinetex particles) and wide PSDs (RSD = 25.3%, like the fully porous 1.7-µm BEH particles [Waters]) supported this experimental conclusion. A posteriori, this makes sense when we remember that particles are randomly distributed in LC columns, regardless of the width of their PSDs. The external porosity of columns packed with conventional porous particles (0.34–0.37) is smaller than that of columns packed with core–shell (0.39–0.42) particles. Therefore, one would expect the eddy dispersion through beds of fully porous particles to be smaller than that through beds of core–shell particles. This demonstrates that the low A term obtained with columns packed with these new particles has nothing to do with their tight PSD. In the next section, we investigate the physical phenomena that account for band broadening in the interstitial mobile phase and explain the exceptional performance of 4.6-mm i.d. columns packed with sub-3-µm shell particles.

Eddy Dispersion in Today's Packed LC Columns (4.6-mm i.d.)

The legend that the eddy dispersion contribution to HETP is constant and should be written as A = 2y₂d_p with y₂ ≈ 0.5 should be revisited. Figure 5 compares the experimental eddy dispersion term measured for naphthalene on 100 mm × 4.6 mm columns packed with 2.6-µm Kinetex and 2.5-µm Luna particles with those predicted by different models (Gunn [29] in green and Giddings [21] in blue) and by a bed reconstruction and mass transfer simulation (30) in pink. In each model, the infinite-diameter column (no wall effects) was assumed, which means that the eddy dispersion term is predicted for the bulk region of the random packing, free from confinement and wall effects. In other words, the velocity biases assumed in these models are limited to those taking place over short scale lengths, either over short interparticle distances (trans channel eddy diffusion) or a few particle diameters (short-range interchannel eddy diffusion).

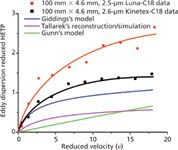
Figure 5: Comparison between experimental data (100 mm × 4.6 mm columns) and theoretical expressions of the reduced eddy dispersion HETP assuming the infinite diameter column.

All these models confirm that for reduced velocities between 0 and 20, the eddy dispersion is not constant but increases with increasing flow rate, in qualitative agreement with the experimental results. There is also no doubt that the experimental eddy dispersion data are larger than the predicted values and that this difference exceeds the experimental errors, which are assessed from the data scattering. This quantitative disagreement demonstrates that there are additional sources of velocity biases that were not considered in the dispersion models. Indeed, the column diameter of actual columns is finite and so is the column aspect ratio (column-to-particle diameter ratio). The presence of the column wall affects the way in which particles are arranged across the column. The packing structure is likely to change from the wall region to the center region and the flow hydrodynamics may slightly differ in these two locations. Therefore, transcolumn velocity biases should be added to the models to account for experimental observations.

We applied the dispersion model developed by Tallarek (30) for the bulk region of packed beds (ε_e = 0.40) and added a third eddy diffusion term for which we derived two new parameters (λ₃and ω₃) by minimizing the sum of the relative residuals between experimental and predicted data for the Kinetex column. This gave the following new A term:

The best values of the adjusted parameters are ω_{3, Kinetex} = 0.31 and λ_{3, Kinetex} = 0.54 for this column. The same fit was performed with the best modified dispersion model of Tallarek but assuming an external porosity of 0.35, corresponding to the external porosity of the Luna column.

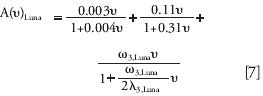
The best values of these parameters become ω_{3, Luna} = 0.54 and λ_{3, Luna} = 1.28 for the Luna column.

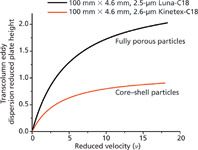
Figure 6: Comparison between the transcolumn reduced eddy dispersion HETP terms isolated semiempirically for core–shell (red) and fully porous (black) particles in 100 mm × 4.6 mm packed columns.

Figure 6 shows plots of the trans-column eddy dispersion term calculated for the Kinetex and the Luna columns. Based on the theory of Giddings, the interpretation for these differences in terms of the importance of the trans-column relative velocity biases (ω_β;,3), the characteristic radial diffusion (ω_α,3), and persistence-of-velocity (ω_λ,3) lengths are necessarily ambiguous. Indeed, ω₃ and λ₃ are written (21):

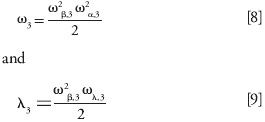
For each column, we have three unknowns, but only two equations. If we first assume that the velocity biases are the same for both columns (ω_β,3,Luna and ω_β,3,Kinetex), we would come to the conclusion that the radial diffusion and the flow-to-persistence length are 32% and 137% larger, respectively, for the Luna than for the Kinetex column. Second, if we assume that the persistence-of-velocity length is unchanged (ω_λ,3,Luna and ω_λ,3,Kinetex), then the velocity bias and the diffusion length would be 54% larger and 14% smaller, respectively, in the Luna than in the Kinetex column. Finally, if the diffusion length is assumed to be constant (ω_α,3,Luna and ω_λ,3,Kinetex), the velocity bias would increase by up to 30% and the flow-to-persistence length would also increase by nearly 40%. Additional experiments are necessary to refine this interpretation. The relative velocity bias, ω_α,3, was measured by local electrochemical detection at the outlet cross-section of a 100 mm × 4.6 mm column packed with 2.6-µm Kinetex C18 particles. It was found that ω_α,3,Kinetex = 1.70 ± 0.2% for six consecutive injections at the very same locations in the wall and center regions of the column (31).

Following a similar experimental approach, the average relative velocity bias (over four different angular positions separated by a 90° angle) measured across a 150 mm × 4.6 mm column packed with 3.0-µm Luna particles was ω_β,3,Luna = 1.76 ± 0.37% [32]. Accordingly, we must conclude that the actual velocity biases are comparable for all 4.6-mm i.d. columns, regardless of the nature of the particles (rough core–shell or smooth fully porous particles) that they are packed with. This seems to indicate that the extent of the packing heterogeneity from the wall toward the center of the column is slightly larger with columns packed with conventional particles than with columns packed with core–shell particles. The radial diffusion length can be estimated easily from equation 8 at 61 Luna particle diameters (153 µm or 6.7% of the column radius) and only 44 Kinetex particle diameters (114 µm or 4.9% of the column radius). In contrast, the flow-to-persistence length estimated from equation 9 is about two times larger along the Luna column (22.1 mm) than along the Kinetex (9.7 mm) and appears to be the main factor responsible for the exceptional performance of columns packed with core–shell particles.

Conclusions

This detailed investigation of mass transfer phenomena in 100 mm × 4.6 mm columns packed with sub-3-µm core–shell and fully porous particles provides a wealth of information regarding which kinetic factors effectively control the efficiency of these columns. First, the success of core–shell particles in the separation of small molecules is not primarily a result of the decrease in the C term, as is often claimed in commercial brochures. Actually, the contribution of this term to the total plate height is already negligible for columns packed with fully porous particles (<5%). Second, the impact of axial diffusion is underestimated in reversed-phase LC for retained analytes at the optimum reduced velocities (υ ≈ 10). The presence of the impermeable cores contributes to increases in the optimum efficiency of columns packed with 2.6-µm particles by about 25,000 plates per meter when the ratio of the core to the particle diameter is 0.7, which is not negligible. Third, and most importantly, the exceptional performance of 4.6-mm i.d. columns packed with core–shell particles is caused by a reduction of the transcolumn eddy dispersion term, which cannot be related to the tightness of the PSD of these particles. It is not yet clear whether this enhanced homogeneity of the packed bed structure across the whole column is a result of smaller transcolumn velocity biases or shorter characteristic distances along which these biases are formed. The origin of such a difference could be the marked roughness of the external surface area of core–shell particles, which would affect the arrangement of the particles differently in the wall and in the central regions of the column during the packing process. Rheological measurements and the determination of the particle-to-particle and particle-to-stainless steel friction coefficients are definitely needed to clarify this issue.

Sub-3-µm core–shell particles are successful for quite unexpected reasons, which were ignored by their earlier manufacturers when they began their research and development efforts. Scientists take advantage of these new columns to refine their understanding of mass transfer phenomena in packed beds. Yet, old questions remain after 50 years of progress in column technology. How can we pack core–shell particles into more efficient and more reproducible narrow-bore columns? Can we find an experimental approach to get rid of the confinement caused by the column wall, which deteriorates column efficiency? Is the concept of external film mass transfer defined by chemical engineers useful in the field of HPLC? Should we not revisit old quantitative estimates of eddy dispersion in packed beds? All these questions should keep us working longer. Other beautiful chromatographic theories could possibly be challenged by new ugly facts.

References

(1) Cs. Horváth, B. Preiss, and S. Lipsky, Anal. Chem. 39, 1422–1428 (1967).

(2) Cs. Horváth and S. Lipsky, Anal. Chem. 41, 1227–1234 (1969).

(3) Cs. Horváth and S. Lipsky, J. Chromatogr. Sci. 7, 109–116 (1969).

(4) J. Kirkland, Anal. Chem. 41, 218–220 (1969).

(5) J. Kirkland, Anal. Chem. 43, 36A–48A (1971).

(6) J. Kirkland, Anal. Chem. 64, 1239–1245 (1992).

(7) G. Guiochon and F. Gritti, J. Chromatogr. A 1218, 1915–1938 (2011).

(8) J. van Deemter, F. Zuiderweg, and A. Klinkenberg, Chem. Eng. Sci. 5, 271–289 (1956).

(9) U.D. Neue, HPLC Columns, Theory, Technology, and Practice (Wiley-VCH, New York, New York, 1997).

(10) J. Mazzeo, U. Neue, M. Kele, and R. Plumb, Anal. Chem. 77, 460A–647A, (2005).

(11) J. Kirkland, T. Langlois, and J. DeStefano, Am. Lab. 39, 18–21(2007).

(12) F. Gritti, A. Cavazzini, N. Marchetti, and G. Guiochon, J. Chromatogr. A 1157, 289–303 (2007).

(13) F. Gritti, C. Sanchez, T. Farkas, and G. Guiochon, J. Chromatogr. A 1217, 3000–3012 (2010).

(14) F. Gritti and G. Guiochon, J. Chromatogr. A 1221, 2–40 (2012).

(15) F. Gritti and G. Guiochon, Chem. Eng. Sci. 66, 6168–6179 (2011).

(16) F. Gritti and G. Guiochon, J. Chromatogr. A 1218, 5216–5227 (2011).

(17) J.H. Knox and L. McLaren, Anal. Chem. 36, 1477–1482 (1964).

(18) K. Kaczmarski and G. Guiochon, Anal.Chem. 79, 4648–4656 (2007).

(19) F. Gritti and G. Guiochon, J. Chromatogr. A 1218, 4452–4461 (2011).

(20) P. Stevenson, F. Gritti, and G. Guiochon, J. Chromatogr. A 1218, 8255–8263 (2011).

(21) J.C. Giddings, Dynamics of Chromatography (Marcel Dekker, New York, New York, 1965).

(22) F. Gritti and G. Guiochon, J. Chromatogr. A in press, http://dx.doi.org/10.1016/j.chroma.2012.05.072

(23) I. Halasz and M. Naefe, Anal. Chem. 44, 76–84 (1972).

(24) J. Done and J. Knox, J. Chromatogr. Sci. 10, 606–615 (1972).

(25) R. Endele, I. Halasz, and K. Unger, J. Chromatogr. 99, 377–393 (1974).

(26) C. Dewaele and M. Verzele, J. Chromatogr. A 260, 13–21 (1983).

(27) F. Gritti and G. Guiochon, J. Chromatogr. A 1218, 8209–8221 (2011).

(28) A. Daneyko, A. Holtzel, S. Khirevich, and U. Tallarek, Anal. Chem. 83, 3903–3910 (2011).

(29) D.J. Gunn, Trans. Instn. Chem. Engrs. 47, T351–T359 (1969).

(30) A. Daneyko, S. Khirevich, A. Holtzel, A. Seidel-Morgenstern, and U. Tallarek, J. Chromatogr. A 1218, 8231–8248 (2011).

(31) F. Gritti, I. Leonardis, J. Abia, and G. Guiochon, J. Chromatogr. A 1217, 3819–3843 (2010).

(32) J. Abia, K. Mriziq, and G. Guiochon, J. Chromatogr. A 1216, 3185–3191 (2009).

(33) J.J DeStefano, T.J. Langlois, and J.J. Kirkland, J. Chromatogr. Sci. 46, 254–260 (2008).

Fabrice Gritti is a Research Scientist at the University of Tennessee in Knoxville, Tennessee. Georges Guiochon is a Distinguished Scientist and Professor at the University of Tennessee. Please direct correspondence to: gritti@ion.chem.utk.edu.