Chromatography Fundamentals, Part III: Retention Parameters of Liquid Chromatography

In Part III of this series, we review different approaches for measuring solute retention in liquid chromatography. The origins of these parameters are described, as well as their significance and applications.

In Part I, we established that chromatographic separations are governed by the distribution coefficient of solutes when they partition between mobile and stationary phases (1). The energetics of this process, described in Part II (2), were shown to depend upon both enthalpic and entropic changes that take place; either of which can dominate, depending upon the separation mechanism. Part III of this series reviews approaches for determining liquid chromatography (LC) retention characteristics of solutes, their derivations, and applications. In this manner, the relationship between solute retention and thermodynamic parameters can be established.

Background

Chromatography is a dynamic process in which solutes are in a state of continuous sorption and desorption from the mobile phase to the stationary phase. Since the retention of solutes by the stationary phase is characteristic of their chemical and structural composition, approaches are needed to accurately measure elution time or volume.

Historically, retention parameters were required for sample identification and characterizing adsorbents and solvents used for elution. These parameters were also deemed useful for studying the separation process, including peak broadening. Different retention parameters have been introduced, depending upon the LC technique employed; each of these approaches are reviewed in detail.

Chromatographic Retention

Retention Factor, k

During elution, solute molecules are in continuous motion, traveling longitudinally through a column, and dancing laterally between stationary and mobile phases. The ratio of the average time a solute molecule spends in the stationary phase (t_s)_i to the time it spends in the mobile phase (t_m)_i, at each elution volume increment i, defines the local retention factor:

Equation 1 represents a snapshot of the activity of a solute at a given instance of time at equilibrium. By integrating these time increments, we can now formulate a measurable quantity:

where t_s is the total time a solute spends in the stationary phase, and t_m is the total time in the mobile phase.

In practice, the measured elution time of a solute t_r is equal to the time spent in the stationary phase plus the time spent in the mobile phase: t_r = t_s + t_m. Furthermore, the time spent in the mobile phase is identical to the elution time of an unretained peak t₀. Equation 2 becomes

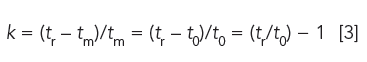
This term was formerly called the capacity factor k', but has been renamed retention factor k. By subtracting unity, as shown in the last part of Equation 3, we uncouple the time or volume a solute spends in the mobile phase. Thus k represents the retention properties of a solute in the stationary phase. Since t_r is normalized with respect to t₀, k is independent of flow rate and column geometry-that is, column length and inner diameter. Because of these properties, k and not retention time should be used for peak identification, reproducibility evaluation, and method validation. Retention time precision, obtained using an unretained peak t₀, however, can be used for assessing flow rate or instrument reliability.

Retention Factor, R_f

Retention is also used in both planar (paper and thin layer) and classical, open-column chromatography, and is still popular among aficionados of these techniques. This definition has the form

where d₀ is the migration distance of the solvent front measured from the bottom of the plate in contact with developing solvent, and d_r is the migration distance of the solute spot on the plate or on paper. In open-column chromatography, the retention is ascertained in the opposite direction, going from column top to bottom, with d_r typically being the length of the packed bed, and d₀ the distance from the top of the bed to the solute band or zone after a set elution time.

R_f values from thin-layer chromatography (TLC) are used for peak identification and evaluating adsorbent–solvent systems. The retention factor is used less frequently for open-column LC because of poor reproducibility. This mode of LC, however, is used mainly for preparative, not analytical, separations.

Retention, R

The next retention parameter R is defined as the ratio of the time a solute spends in the mobile phase t₀ with respect to the total time it remains in the column t_r,

By inference, the ratio is also equivalent to the amount of solute in the mobile phase at any given time to the total amount injected, w_t = w_m + w_s, where w_m and w_s are the solute weight in the mobile and stationary phases, respectively. Thus

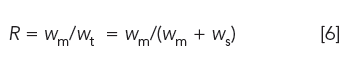
Retention Volume, V_r

Equation 6 allows us to develop a relationship between retention and the distribution coefficient K. This transformation is accomplished by replacing solute weights with corresponding concentrations and volumes (w_m = c_mV_m and w_s = c_sV_s),

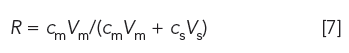
Dividing numerator and denominator by c_m, and letting K = c_s/c_m (see Part I), we arrive at

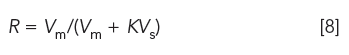
We return to Equation 5, and multiply both numerator and denominator by flow rate F,

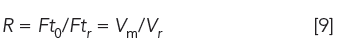
Equation 8 is set equal to equation 9, and we solve for V_r, obtaining

Equation 10 is one of the more important relationships in chromatography. It tells us that the retention a solute is simply the sum of two volumes: the mobile phase V_m, plus some fraction or multiple of the stationary phase, KV_s. The separation mechanism is defined by K and column properties by V_s and V_m.

Equation 10 is the general chromatographic equation valid for every mode of chromatography, with modification as required. For example, if the separation is based on adsorption, the surface area of the packing is used in place of V_s. If the separation is controlled by several mechanisms (an undesirable situation, as described in Part I), additional distribution coefficients must be added, along with the stationary-phase volume (or amount) associated with each distribution coefficient:

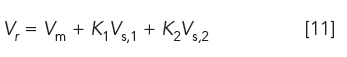
In actual practice, however, it is preferable to optimize the separation, such that a single mechanism dominates to ensure reproducible, high resolution results. If not, mixed-mechanism separations can lead to a metastable LC system.

SEC Elution Volume, V_e

When using size-exclusion chromatography (SEC), Equation 10 must be modified, since the entire SEC separation occurs within the pore volume V_i of the packing (3). Thus, V_m is replaced with V₀ + K_SECV_i, where V₀ is the interstitial volume of the packed column (since there is no partition or adsorption process, K = 0 in Equation 10). The correct distribution coefficient to use is K_SEC, which ranges from zero to unity. For SEC, Equation 10 becomes

Equation 12 is used when constructing SEC calibration plots (log molecular weight versus V_e). Furthermore, K_SEC is usually monitored during SEC method development to ensure the absence of intermolecular interaction between solute and packing. Finally, the proper term to use in SEC is elution volume V_e, rather than retention volume, since there is no retention, K = 0.

Distribution Coefficient, K

We can now establish a relationship between the retention factor k, which can be readily measured, and the distribution coefficient K, a therodynamic parameter. Elution times in Equation 3 are converted to elution volumes by multiplying the numerator and denominator by the flow rate. After rearranging, Equation 3 becomes

Setting Equations 13 and 10 equal to one another and rearranging terms, we arrive at

The V_s/V_m term, called the phase ratio, controls the elution properties of a solute. In effect, the elution properties of a given separation can be adjusted through the phase ratio, provided that the distribution coefficient is kept constant.

It is important to note that k can be used in place of K to establish thermodynamic relationships or trends among compounds, or used to characterize the thermodynamic properties of chromatographic systems.

Conclusions

All possible LC retention parameters are summarized in Table I. These retention parameters are based on the distribution coefficient, which drives the separation process (see Part I).

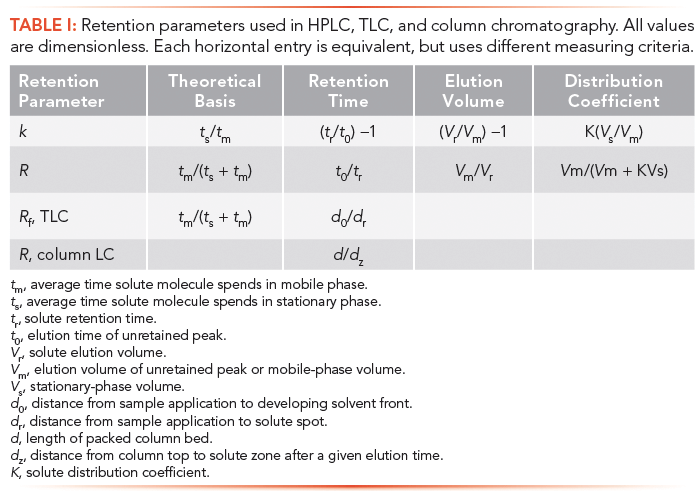
Historically, the parameter R was used to study chromatographic properties of adsorbents and to establish a system of identifying and categorizing elution behavior of compounds. The R_f retention factor was used for identification of solutes with planar chromatographic methods. With the introduction of high performance liquid chromatography (HPLC) more than 50 years ago, the capacity factor k', subsequently renamed the retention factor k, is the most widely used HPLC parameter for characterizing elution properties of solutes, validating LC methods, identifying compounds, and accessing thermodynamic behavior of solutes.

Subsequent articles will discuss the origin, significance, and measurement of theoretical plates and peak broadening in LC.

References

(1) H.G. Barth, LCGC North Am. 36(3), 200–202 (2018).

(2) H.G. Barth, LCGC North Am. 36(6), 394–396, 405 (2018).

(3) H.G. Barth, J. Chem. Educ. (Online early access). http://pubs.acs.org/doi/abs/10.1021/acs.jchemed.8b00171

(4) P.A. Bristow, Liquid Chromatography in Practice (hetp, Cheshire, UK, 1976).

(5) E. Heftmann, Chromatography, 3rd Ed. (Reinhold, New York, 1975).

(6) B.L. Karger, L.R. Snyder, and C. Horvath, An Introduction to Separation Science (Wiley-Intersciences, NY, 1973).

(7) L.R. Snyder, J.J. Kirkland, and J.W. Dolan, Introduction to Modern Liquid Chromatography, 3rd Ed. (Wiley, New York, 2010).

Howard G. Barth is with Analytical Chemistry Consultants, Ltd., in Wilmington, Deleware. Direct correspondence to: howardbarth@gmail.com