uHPLC Teaching Assistant: A New Tool for Learning and Teaching Liquid Chromatography, Part 1

Photo Credit: Torla/Shutterstock.com - Diagram: Courtesy of the Author

Davy Guillarme and Jean-Luc Veuthey, School of Pharmaceutical Sciences, University of Geneva, University of Lausanne, Geneva, Switzerland

The free spreadsheet-based program HPLC Teaching Assistant was developed for effective and innovative learning and teaching of liquid chromatography. This software allows teachers to illustrate the basic principles of high performance liquid chromatography (HPLC) using virtual chromatograms (simulated chromatograms) obtained under various analytical conditions. In the first instalment of this series, we demonstrate the possibilities offered by this spreadsheet to illustrate the concept of chromatographic resolution, including the impact of retention, selectivity, and efficiency; understand the plate height (van Deemter) equation and kinetic performance in HPLC; recognize the importance of analyte lipophilicity (log P) on retention and selectivity in reversed-phase HPLC mode; and manipulate or adapt reversed-phase HPLC retention, taking into account the acido-basic properties (pKa) of compounds and the mobile-phase pH.

High performance liquid chromatography (HPLC) remains one of the most widely used analytical techniques in industry and is taught at universities in analytical chemistry programmes. Because HPLC separation is
based on the partitioning of a solute between a mobile phase and a stationary phase, the technique is particularly difficult to master. There are a significant number of parameters (for example, physicochemical properties and molecular weight of the solutes; nature, composition, temperature, pH, and flow rate of the mobile phase; and chemical nature and dimensions of the stationary phase) that can influence the quality of the separation (retention time, selectivity, efficiency, pressure drop, peak area).

Several commercial HPLC simulators are available on the market, including Drylab (Molnar-Institute) (1), Chromsword (Iris Tech) (2), LC & GC Simulator (Advanced Chemistry Development), and Osiris (Datalys) (3). These simulators are particularly useful for efficiently developing HPLC methods based on a limited number of initial experiments and can also be used to better understand the principles of HPLC. However, these simulators remain difficult to use and expensive to purchase.

To easily understand the principles of chromatography in a relatively inexpensive way, some free or low-cost computer‑based HPLC simulators have also been proposed (4–7). As reported by Boswell and colleagues (8), there are currently six HPLC simulators, but most are not available anymore or are not fully compatible with modern computers. To the best of our knowledge, the most interesting HPLC simulator was released in 2013 and is free (8). The software interface is relatively easy to use and produces a simulated chromatogram that is redrawn when the experimental parameters are changed.

In comparison to this software, the philosophy of our program, entitled “HPLC Teaching Assistant”, is different but complementary. First, our program is a spreadsheet that can be easily used on any computer without the need to install a Java environment. Second, our spreadsheet allows making links between compounds’ physicochemical properties (partitioning coefficient, pKa) and chromatographic behaviour. Last but not least, each spreadsheet of our calculator provides an understanding of a single concept (for example, the effect of the mobile‑phase pH on the chromatographic behaviour). This series of two articles provides the theoretical background of the spreadsheet and gives some practical examples to illustrate the usefulness of this tool (see Figure 1).

Understanding the Concept of Chromatographic Resolution

Theoretical Background: In liquid chromatography (LC), the separation of two peaks is described by their resolution (Rs), which represents the difference in retention times (tR) divided by the average peak widths at baseline (W), according to the following equation:         

[1]

To better understand the impact of analytical conditions on the overall resolution, the fundamental equation of resolution can be used to interpret the chromatograms obtained during method development. Rs can also be expressed based on the retention factor (k), selectivity (α), and plate number (N):

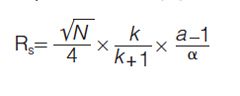
            [2]

Using the “Resolution” Spreadsheet: In the first spreadsheet, entitled “resolution”, the impact of k, α, and N on Rs can be directly visualized. For this purpose, a chromatogram was simulated to show the chromatographic resolution of two molecules when modifying k, α, and N. For this example, the column had a void time of 1.74 min (corresponding to a 150 mm × 4.6 mm column operated at 1 mL/min).

In addition to the simulated chromatogram, three graphics at the bottom of the spreadsheet illustrate the change in resolution with the three individual variables, namely, k, α, and N. The impact of α on the resolution is strong: For α values between 1 and 4, the resolution drastically increases, and a plateau is only attained for very high (inaccessible) α values (α > 4). The retention factor also has a significant impact on resolution for low k values, but its impact becomes moderate for k between 3 and 10 and low for k higher than 10. For k > 10, the analysis time becomes long, and the improvement in the resolution is minor (this can be easily verified by simulating a chromatogram obtained under conditions of very high k values). Finally, because efficiency is expressed as the square root in the resolution equation, its impact on resolution is moderate to low, and N must be dramatically increased to significantly improve Rs.

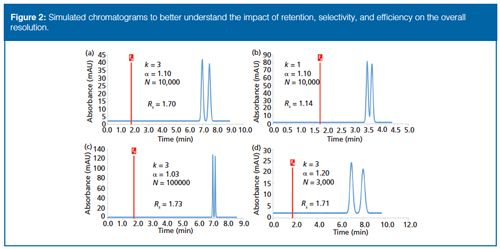
From this spreadsheet, four different conditions were simulated to better illustrate the influence of k, α, and N on Rs, as shown in Figure 2. In Figure 2(a), the selectivity, retention factor, and efficiency were reasonable, leading to an overall resolution of 1.70. Figure 2(b) illustrates the conditions of an insufficient retention factor in LC (k = 1), thereby leading to a significant reduction in resolution (Rs = 1.14). Figure 2(c) shows that a very low selectivity (α = 1.03) can be compensated by a very high plate number (N = 100,000 plates). Finally, Figure 2(d) proves that selectivity is the primary driver for optimizing chromatographic separation in LC. As shown on this simulated chromatogram, when the selectivity is appropriate (α = 1.20), there is no need for a very high plate count (only 3000 plates in this case).

Based on these observations, it is easy to understand why method development follows three successive steps after selecting the appropriate stationary and mobile-phase conditions:

• Select a column with a sufficient plate number considering the complexity of the sample to be analyzed (usually a column generating 10,000 plates is a good starting point).

• Adjust the solvent strength to have a reasonable retention factor (k between 2 and 10).

• Optimize selectivity by tuning different chromatographic parameters.

Understanding the Kinetic Performance and van Deemter Curves 

Theoretical Background: In LC, the column dimensions (length, internal diameter, and particle size), as well as the mobile-phase flow rate and viscosity, have an impact on the plate number (N) and column back pressure (ΔP). In the second spreadsheet, the kinetic performance is shown for a mixture of three compounds with a molecular weight of approximately 100 g/mol. All the calculations were made for a column with a total porosity (ε) of 0.7 and a flow resistance (Φ) of 500. The column temperature was 30 °C, and the mobile phase was a mixture of 30% acetonitrile and 70% aqueous buffer.

To calculate the plate number (N) based on the column dimensions, the following equation was used:

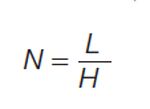
            [3]

where L is the column length (in millimetres) and H is the height equivalent to a theoretical plate (in micrometres). To estimate the H value, the van Deemter equation was considered:

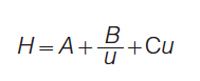
            [4]

In this equation, A, B, and C correspond to eddy dispersion, longitudinal diffusion, and mass transfer, respectively. They represent a different set of constants for a particular solute, column, and mobile-phase conditions. The u value is the linear velocity, which is related to the mobile-phase flow rate (F), column porosity (ε), and column internal diameter (dc) using the following relationship:

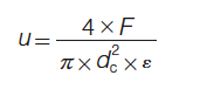
            [5]

To use generic a, b, and c terms (a = 1, b = 4, c = 0.05 in our case), which are independent on the analytical conditions, the van Deemter equation was transformed into its reduced form:

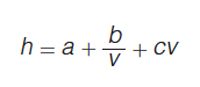
            [6]

where h is the reduced height equivalent to a theoretical plate and ν is the reduced linear velocity. The following two equations provide the definitions for these two reduced parameters.

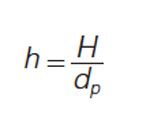
            [7]

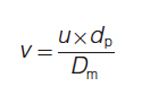
[8]

Here, dp represents the column particle diameter (in micrometres) and Dm is the diffusion coefficient of the solute in the mobile phase, which can be estimated using the Wilke‑Change equation (9).

Finally, the column pressure drop was calculated using Darcy’s law, with η being the mobile-phase viscosity:

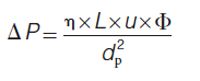
            [9]

In the spreadsheet entitled “Efficiency”, the impact of the column dimensions (Lcol, dcol, and dp) and the mobile‑phase flow rate (F) on N and ΔP can be directly visualized. A simulated chromatogram with three compounds (k = 1.0, 2.6, and 3.0) shows the corresponding chromatogram when modifying the column dimensions and flow rate. In addition, the van Deemter curve, H = f(u), was drawn, as well as the more practical curve representing N = f(F).

Using the “Efficiency” Spreadsheet: The user can modify the column dimensions (Lcol, dcol, and dp), as well as mobile-phase flow rate, to see the impact on a simulated chromatogram at the bottom of the spreadsheet. The corresponding plate count and column pressure drop are also calculated. In addition, users can visualize the corresponding van Deemter curve and evaluate whether the conditions are far from the optimal linear velocity (or flow rate). This ability to visualize the curve could help users determine the maximum plate count achievable on the column under optimal flow rate conditions.

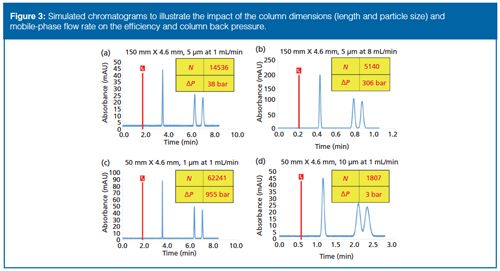
As shown in Figure 3(a), an efficiency of 14,536 plates and a pressure drop of 38 bar were achieved on a 150 mm × 4.6  mm, 5-µm dp column at 1 mL/min, leading to a resolution greater than 1.5 for the chromatogram reported. Increasing the mobile-phase flow rate to 8 mL/min decreased the efficiency to only 5140 plates, which is in agreement with the van Deemter curve (see Figure 3[b]). The pressure drop also increased to 306 bar, as expected from Darcy’s law (equation 9). Next, the impact of particle size was illustrated in Figure 3(c), where the efficiency was drastically improved (N = 62,241 plates) when the particle size was reduced from 5 to 1 µm, but the pressure became incompatible with regular HPLC systems (955 bar). In Figure 3(d), the column length was 50 mm and the particle size was 10 µm. Compared to the chromatogram reported in Figure 3(a), the last two peaks were not baseline resolved because of the poor efficiency (only 1807 plates), which is in agreement with equations 3 and 7. However, with such a short column, the pressure drop was only 3 bar.

Understanding Retention in Reversed-Phase LC

Theoretical Background: In reversed‑phase LC mode, the retention at the surface of the alkyl stationary phase (for example, C18) is related to compound hydrophobicity and is often expressed as the partition coefficient (P). P can be defined as the ratio of the compound concentrations between two immiscible phases (1-octanol and water) at equilibrium. For reasonable partition coefficient values (within a limited range), the logarithm of P should be considered (log P), and its definition is provided below:

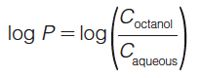
            [10]

As illustrated in Figure 4, when the log P value is less than 0, molecules are considered hydrophilic. Then, the molecule has greater affinity for the hydrophilic mobile phase versus the hydrophobic stationary phase and will therefore be poorly retained. In contrast, when the log P value is greater than 0, the molecule is lipophilic and preferably interacts with the hydrophobic stationary phase, leading to significant retention. A few examples of hydrophilic and lipophilic molecules are given in Figure 4. In regular reversed-phase LC mode (with a C18 stationary phase and acetonitrile–water mobile phase), only compounds with log P values between -1 and +6 can be adequately analyzed.

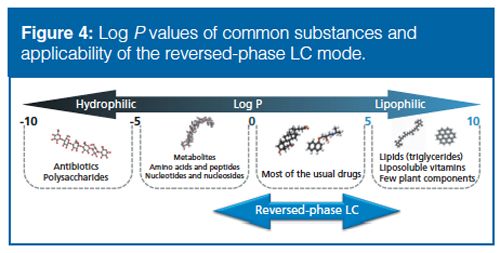
A simulated chromatogram with three compounds with different log P values (set by the user) illustrates the chromatographic behaviour for any mobile-phase composition (methanol percentage). For this spreadsheet, the transformation of log P values into retention times was performed based on the work by Henchoz and colleagues (10), considering a Waters Acquity BEH Shield C18 column and a mobile phase containing methanol and water.

The following empirical equation was used to calculate log kw (the extrapolated retention factor to pure water, mimicking 1-octanol–water partitioning [11]) based on the log P value set by the user (10):

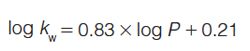
            [11]

Then, log kw was transformed into log k at the mobile-phase composition set by the user using the following equation (12):

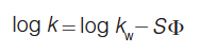
            [12]

where Φ is the volume fraction of the organic solvent (value between 0 and 1) and S is a characteristic constant for each solute, which corresponds to the elution strength of the organic modifier (slope of the logarithmic plot: d(log k)/dΦ). In reversed‑phase LC, the S value varies from approximately 3 (compounds of approximately 100 g/mol) to more than 100 for very large proteins. In this spreadsheet, a generic value of S = 4 was considered because it is representative of low-molecular-weight compounds (<300 g/mol). Finally, the log k values were transformed into tR to construct the final chromatogram, considering the column dead time of a 150 mm × 4.6 mm, 5-µm column at a flow rate of 1 mL/min (column dead time of 1.74 min).

Using the log P Spreadsheet: The user can modify the log P values of three compounds to see the impact on the retention on a simulated chromatogram. In addition, users also have the ability to tune the mobile-phase composition by adjusting the methanol percentage.

As shown in Figure 5(a), when the log P values of the three compounds are between 1 and 1.5, 20% methanol is sufficient to elute all the peaks from the column within a reasonable analysis time of less than 10 min (k values between 1.5 and 4.5). This is because such compounds are not highly lipophilic (intermediate log P values). To speed up the separation, Figure 5(b) shows that the same compounds can be eluted in less than 2.5 min when the mobile-phase composition is increased to 50% methanol. However, under these conditions, the retention was rather low because 50% methanol was too strong of an eluent (k values between 0.1 and 0.3). In Figure 5(c), the compound lipophilicity (log P) was increased in the range of 3.0–3.5. When increasing the log P values by only 2 units, the retention became much higher than Figure 5(a) and the analysis time was 360 min. To achieve a more reasonable analysis time with these lipophilic compounds, the mobile‑phase composition was changed to 50% methanol, and these conditions allow the elution of all three compounds within 25 min.

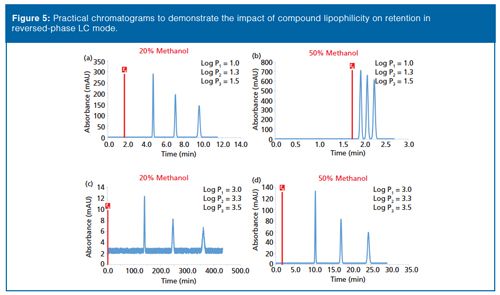
The examples reported in Figure 5 demonstrate the benefits of reversed-phase LC, which can address a wide range of compound lipophilicity using a generic C18 material and simply adjusting the proportion of organic modifier in the mobile phase. If compounds with more diverse lipophilicity have to be analyzed, the isocratic mode would not be valid and gradient elution is used.

Understanding the Impact of Compound Ionization in Reversed-Phase LC

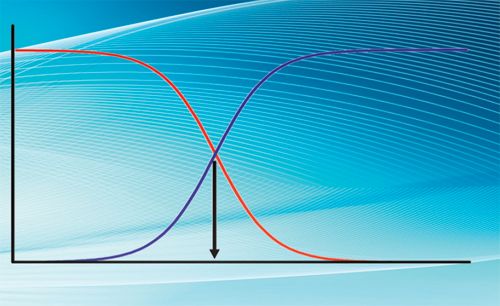
Theoretical Background: An important parameter for tuning retention and selectivity in reversed-phase LC is the mobile‑phase pH. When considering ionizable substances (either acidic or basic), the pH impacts the percentage of neutral and ionized forms. Figure 6 shows the percentages of the ionized form for an acidic (blue sigmoidal curve) and a basic substance (red sigmoidal curve) depending on the buffer pH and analyte pKa.

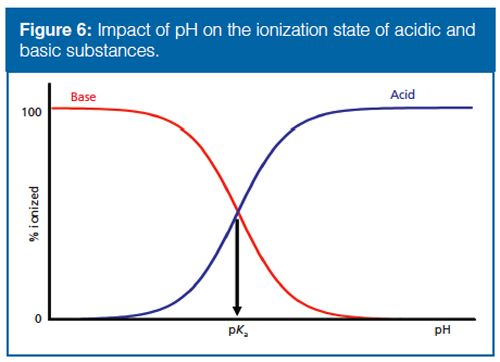
Based on Figure 6, the retention of basic compounds decreases in reversed-phase LC at low pH (pH below the pKa of the molecule) because of the presence of a high amount of ionized form. In contrast, the retention of acidic compounds increases in reversed-phase LC at low pH (pH below the pKa of the molecule) because of the presence of a high proportion of neutral form. Ionized compounds are much more hydrophilic than neutral compounds; therefore, the retention and selectivity can be altered by tuning the mobile-phase pH. In the case of ionizable substances, the partition coefficient (log P) cannot be used and should be replaced by the distribution coefficient (log D) expressed using the following equation for an acid (13):

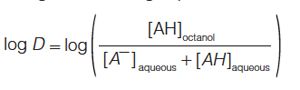
            [13]

For an acid, the percentages of neutral and ionized forms at a given pH are given by the following equations (14):

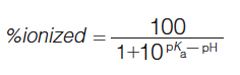
            [14]

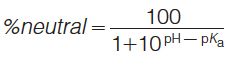
    [15]

For a base, the percentages of neutral and ionized forms at a given pH are given by the following equations (14):

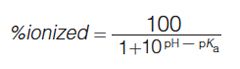
            [16]

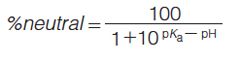
            [17]

All the calculations were made for a 150 mm × 4.6 mm, 5-µm column at a flow rate of 1 mL/min using methanol as the organic modifier. Only two compounds were selected for the simulation to limit the complexity of the sample, and a colour code was used to distinguish the two substances. For these two compounds, the user can set the log P and pKa values, as well as the nature of the compound (acidic or basic). In addition, the user also has to define the mobile‑phase pH and the percentage of methanol in the mobile phase. Based on these inputs, the log D at the pH indicated by the user is calculated using the following two equations for an acid (equation 18) or a base (equation 19) (13):

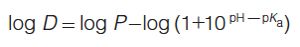
            [18]

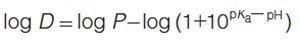
            [19]

For (pH – pKa) values greater than |3.5|, log D was constant. The log D values were then transformed into retention factors using equations 11 and 12.

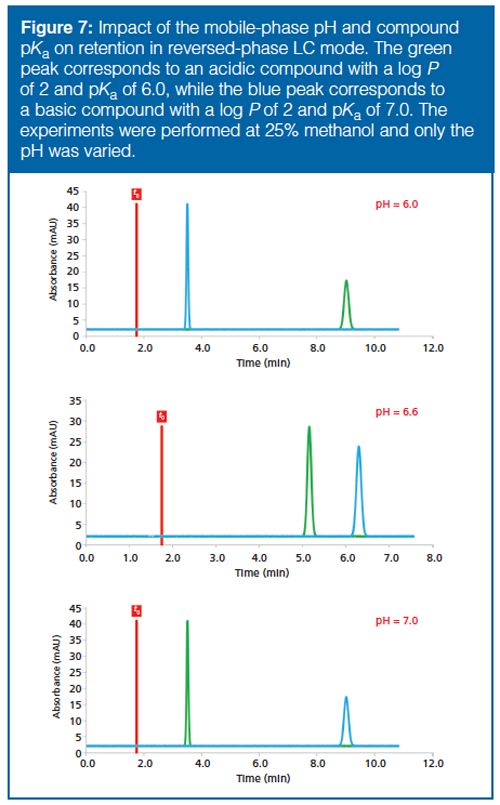
The impact of the mobile-phase pH and compound pKa can be directly visualized. In addition to the simulated chromatogram, the ionization profiles of the two simulated compounds are presented in the upper part of the spreadsheet.

Using the pKa Spreadsheet: Figure 7 illustrates the impact of the mobile-phase pH (modified in a very narrow range around the pKa of the substances) on the chromatographic separation of two species (one acid and one base) with identical log P values. The retention of the acid (green peak) decreases with pH, which is logical because the acid is mostly deprotonated at higher pH (charged compounds are less retained in reversed‑phase LC mode). In contrast, the retention of the base (blue peak) increases with pH because the molecules are less charged at a higher pH. Because the mobile-phase pH was very close to the pKa of the two substances, the impact of a pH change on retention was significant for both compounds. In these three examples, the elution order was completely reversed between pH 6.0 and 7.0.

In the second example (Figure 8), two acids were selected, and the pH was varied in a much wider range (from pH 3.0 to 8.0). The retention was significant at pH 3.0 for these two acidic molecules. The retention factor was approximately 8 on average, and the two peaks were just baseline resolved. When the pH was increased to 6.0, the retention decreased (k between 0.2 and 1.0), but the two peaks were much better resolved. Finally, at more basic pH (8.0), the peaks were not sufficiently retained and were not resolved. Under these conditions, the two acidic molecules were completely deprotonated and unsuitable for reversed‑phase LC at 25% methanol. A lower proportion of methanol would improve the separation quality.

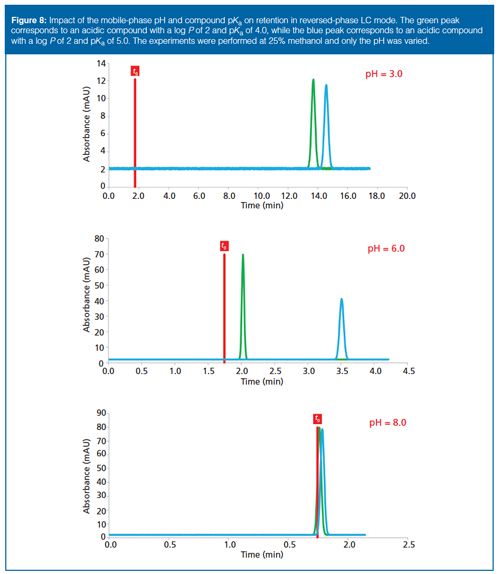
These examples illustrate the impact of the mobile‑phase pH on retention, selectivity, and resolution in reversed‑phase LC mode and the importance of adequately controlling this variable in reversed-phase LC.

Conclusion

In conclusion, the spreadsheet HPLC Teaching Assistant (15) helps in learning and teaching liquid chromatography in an innovative and efficient way, using virtual (simulated) chromatograms obtained under numerous analytical conditions. This tool can be used by academic teachers, as well as company training instructors, who are interested in using innovative technology to better convey information during their courses.

Acknowledgements

The authors wish to thank Dr. Szabolcs Fekete from the University of Geneva for his critical review of the manuscript and his suggestions to improve the spreadsheet.

References

1. I. Molnar, J. Chromatogr. A 965, 175–194 (2002).
2. E.F. Hewitt, P. Lukulay, and S. Galushko, J. Chromatogr. A1107, 79–87 (2006).
3. S. Heinisch and J.L. Rocca, Chromatographia32, 559–565 (1991).
4. R.C. Rittenhouse, J. Chem. Educ. 72, 1086 (1995).
5. I.C. Bowater and I.G. McWilliam, J. Chem. Educ.71, 674–678 (1994).
6. R.A. Shalliker, S. Kayillo, and G.R. Dennis, J. Chem. Educ.85, 1265–1268 (2008).
7. J.C. Reijenga, J. Chromatogr. A 903, 41–48 (2000).
8. P.G. Boswell, D.R. Stoll, P.W. Carr, M.L. Nagel, M.F. Vitha, and G.A. Mabbott, J. Chem. Educ.90, 198–202 (2013).
9. J. Li and P.W. Carr, Anal. Chem.69, 2550–2553 (1997).
10. Y. Henchoz, D. Guillarme, S. Martel, S. Rudaz, J.L. Veuthey, and P.A. Carrupt, Anal. Bioanal. Chem. 394, 1919–1930 (2009).
11. D. Benhaim and E. Grushka, J. Chromatogr. A1217, 65–74 (2010).
12. S. Martel, D. Guillarme, Y. Henchoz, A. Galland, J.L. Veuthey, S. Rudaz, and P.A. Carrupt, in Molecular Drug Properties: Measurement and Prediction, Volume 37, R. Mannhold, Ed. (Wiley‑VCH, 2008), pp. 331–355.
13. R.A. Scherrer and S.M. Howard, J. Med. Chem.20, 53–58 (1977).
14. H.N. Po and N.M. Senozan, J. Chem. Educ.78, 1499–1503 (2001).
15. This spreadsheet can be downloaded for free at: https://epgl.unige.ch/labs/fanal/hplc_teaching:en.

Davy Guillarme and Jean-Luc Veuthey are with the School of Pharmaceutical Sciences at the University of Geneva, University of Lausanne in Geneva, Switzerland.