Tips & Tricks GPC/SEC Particle Size and the Effect of Mixing Columns of Different Particle Size

AlexeyBrin/stock.adobe.com

Compared to other chromatographic techniques, GPC/SEC suffers from a limited resolution. While it is possible to separate individual oligomers up to molar masses of around 1000 Da using suitable columns, it is not possible to resolve individual degrees of polymerization for chains of higher molar masses. Figure 1 shows the chromatogram of a mixture of four polystyrene (PS)standards differing in molar mass While the lowest molar mass standard shows peaks for the individual oligomers (chain lengths), the individual degrees of polymerization of the higher molar mass standards cannot be resolved any longer, due to the logarithmic relation between elution volume and molar mass.

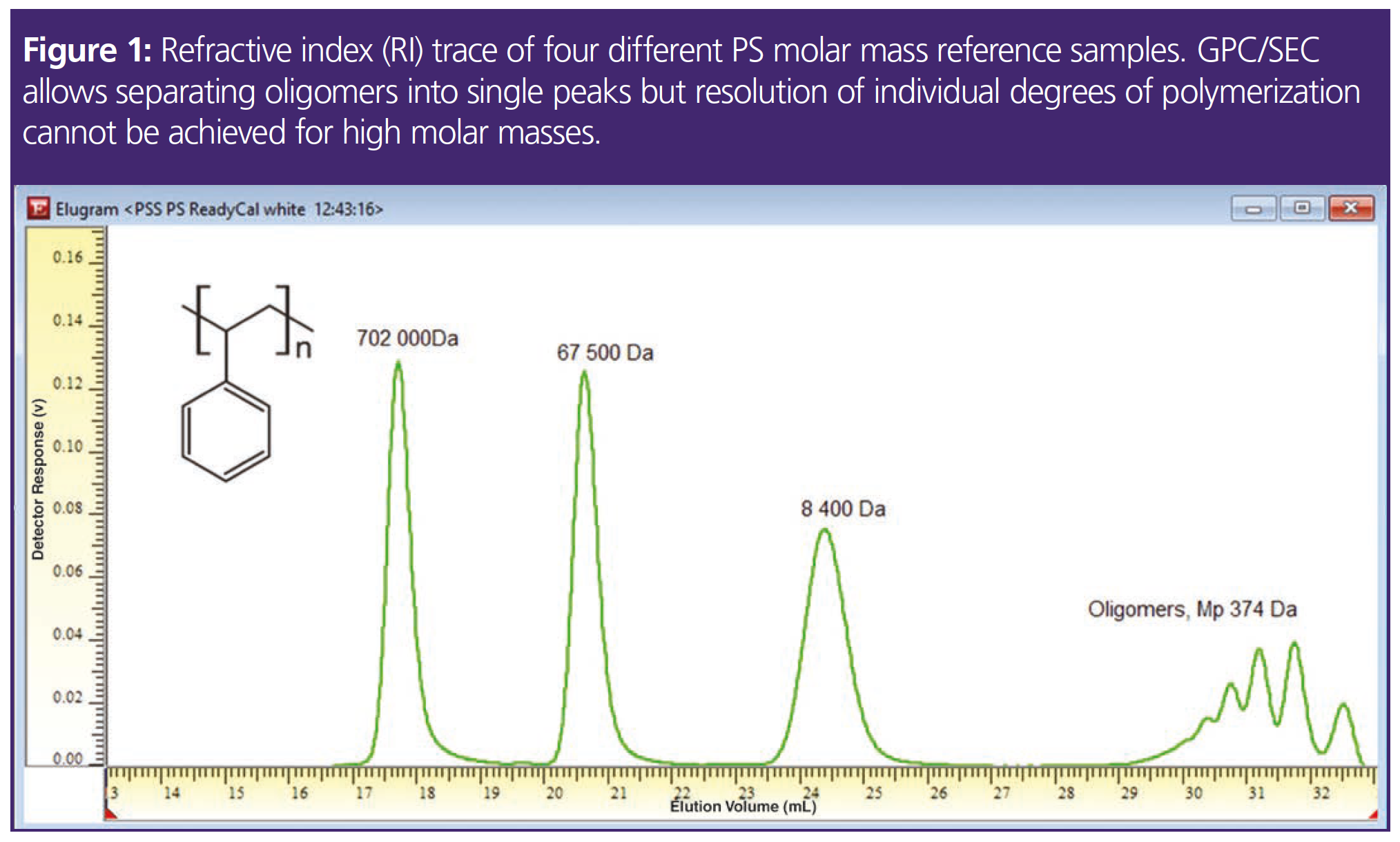
Plate Count

The performance of a chromatographic column, or to be more precise of a chromatographic system, is often characterized by the plate count, N_th (or plate count/m, N_th[m^-1]).

The plate count is a measure for the peak width resulting from band broadening processes when a truly monodisperse sample is run through a chromatographic column. Low plate counts correspond to broad peaks, while columns of high plate numbers produce narrow peaks.

The number of theoretical plates per column can be calculated via:

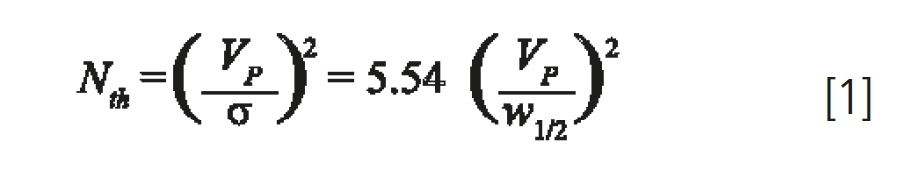
where V_P, σ and w_1/2 denote the peak position, the variance, and the full width at half peak maximum, respectively. To allow for better comparison of columns differing in length, it is useful to define the number of theoretical plates per meter:

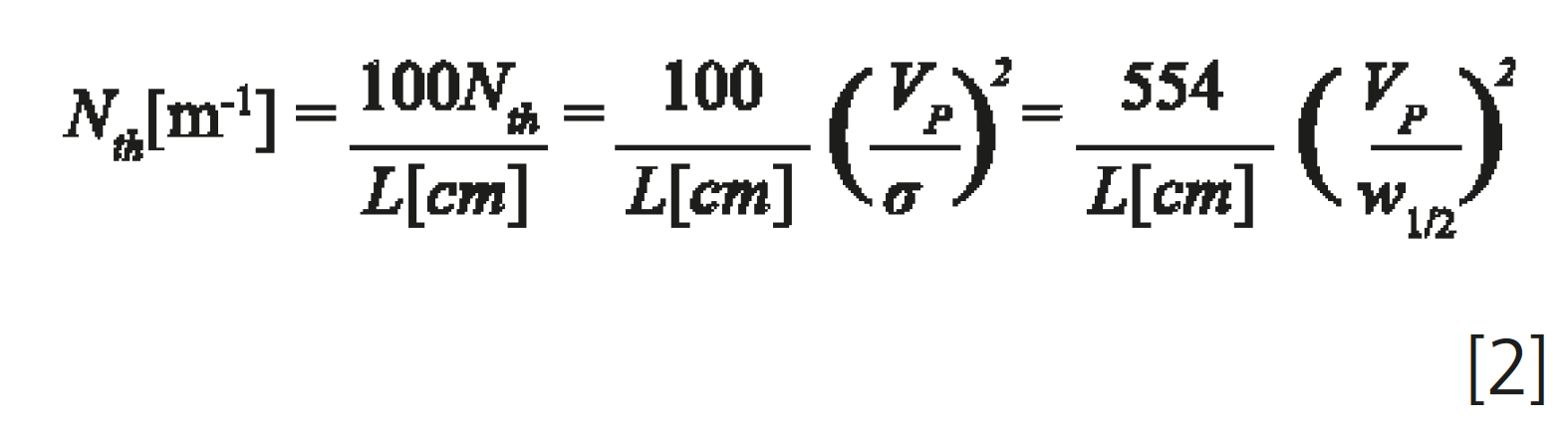
Typically, plate count decreases with the particle diameter. The theoretical possible plate counts range from approximately 80 000 plates/m for 3-µm particles, to approximately 15 000 plates/m columns filled with 20 µm particles (5).

It should be mentioned that plate count does not depend on particle size only but is also affected by other parameters. Ageing or damaging of columns will result in decreased plate counts. Packing quality, detector band broadening, and instrumental peak broadening resulting from finite injection volume and tubing contribute to plate count as well. It is good practice to determine the plate count regularly in order to identify changes of column performance with time (6).

Resolution

While plate counts characterize the general column performance, resolution is the parameter describing the efficiency of a specific separation. The resolution, R_s, between two peaks is calculated by:

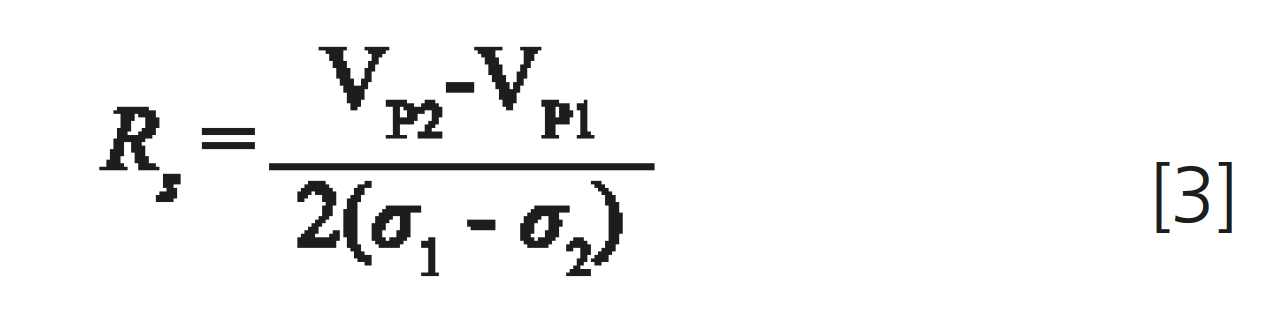
where V_pi and σ_i denote the elution volumes and variances of the two separated peaks, respectively. However, application of this equation to GPC/SEC separations is difficult, as most often GPC/SEC does not result in well separated, but rather in broad unstructured peaks. Therefore, the specific resolution, R_sp, was defined:

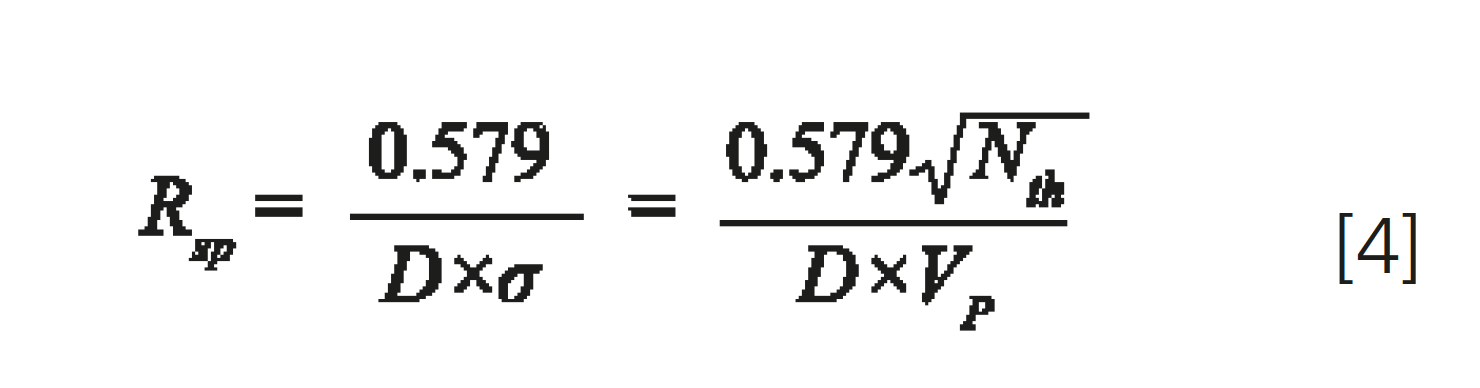
The specific resolution corresponds to the resolution of two (hypothetical) truly monodisperse polymer molecules differing by a factor of 10 in molar mass.

As plate count depends on the particle size, resolution is also affected by particle size.

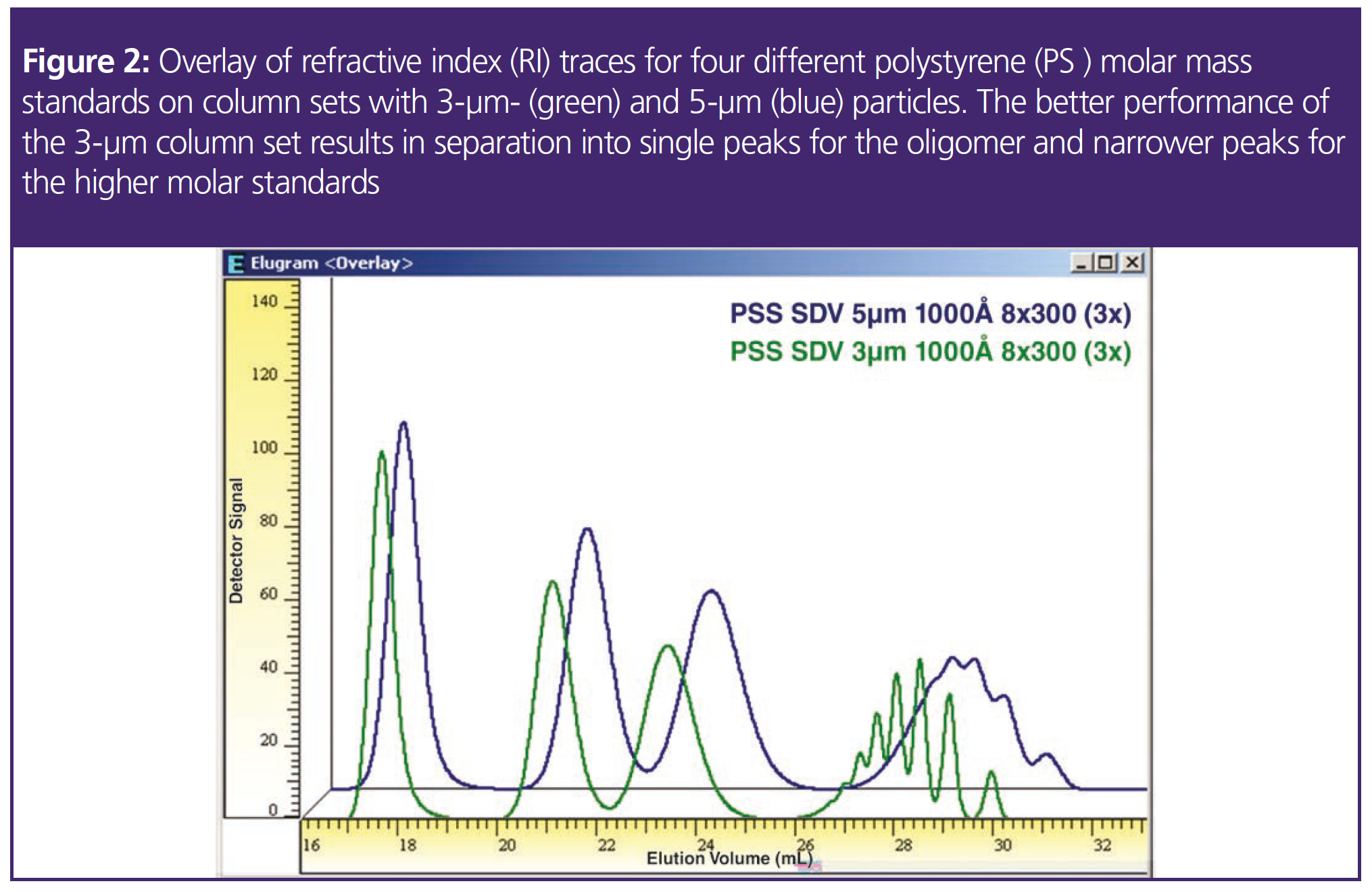
Figure 2 compares two column sets of identical pore sizes but differing in particle size; 3 µm (green) and 5 µm (blue). Again, a PS mixture of four different molar masses has been injected. As results of less band broadening, the column filled with the smaller particles yield a much better resolution for the oligomers.

Combining Columns of Different Particle Size (plate count, resolution)

The effect of combining columns of different particle size and thus performance is schematically depicted in Figure 3.

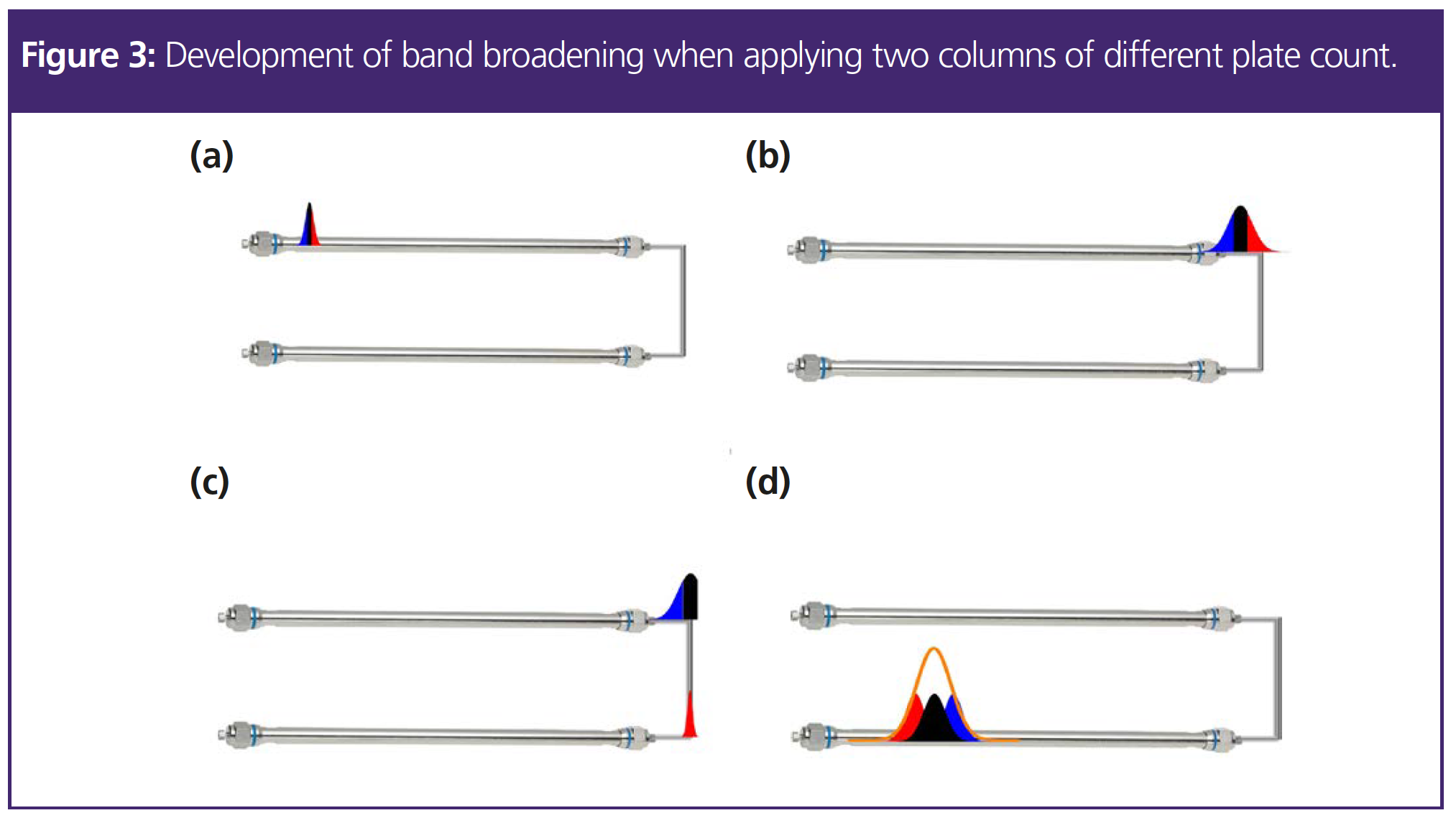
At injection the sample is introduced into the first column as a narrow band of typically 20–100 µL (a) Upon migration through the first column, the narrow band broadens, (b) to an extent depending on the column performance (plate count) of the first column. The transfer of the resulting peak into the second column can be regarded as a consecutive series of injections of narrow bands into the second column (c), where each introduced narrow fraction is subject to additional band, to an extent depending on the column performance (plate count) of the second column. The resulting chromatogram is then the superposition of the chromatograms of the broadened transferred fractions (d).

a) Columns of identical pore size distributions

If both columns are of identical pore size, the peak has the same elution volume on both columns. However, if both columns have different plate counts, the plate count of the column combination is given by:

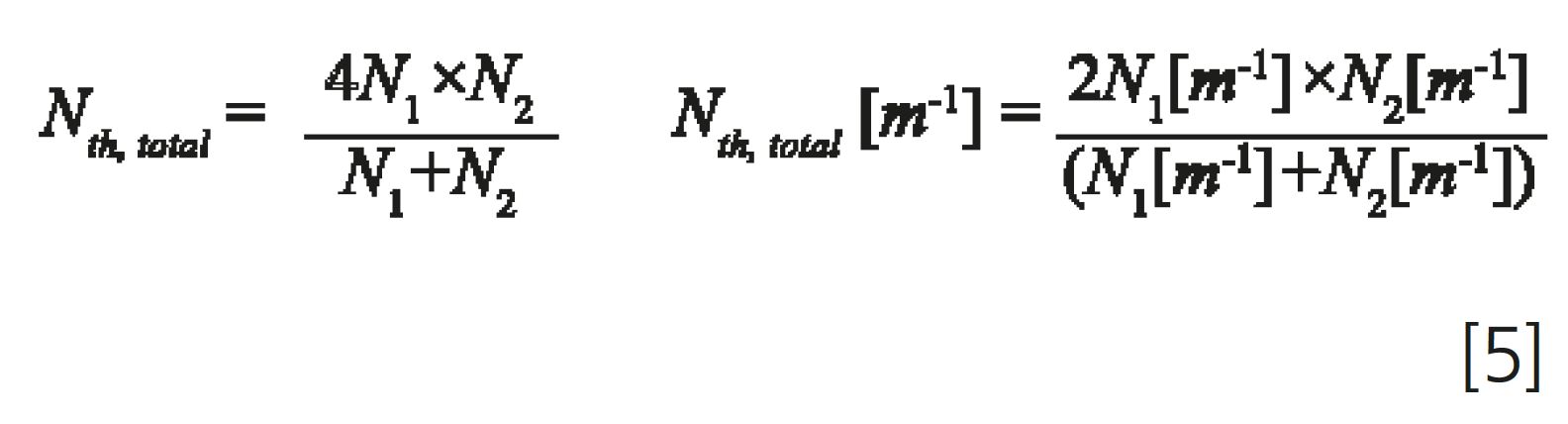
The factor 2 arises from the doubled column length of the column combination, compared with the single column.

If the columns perform very differently, for example, N₁ >>N₂ we find:

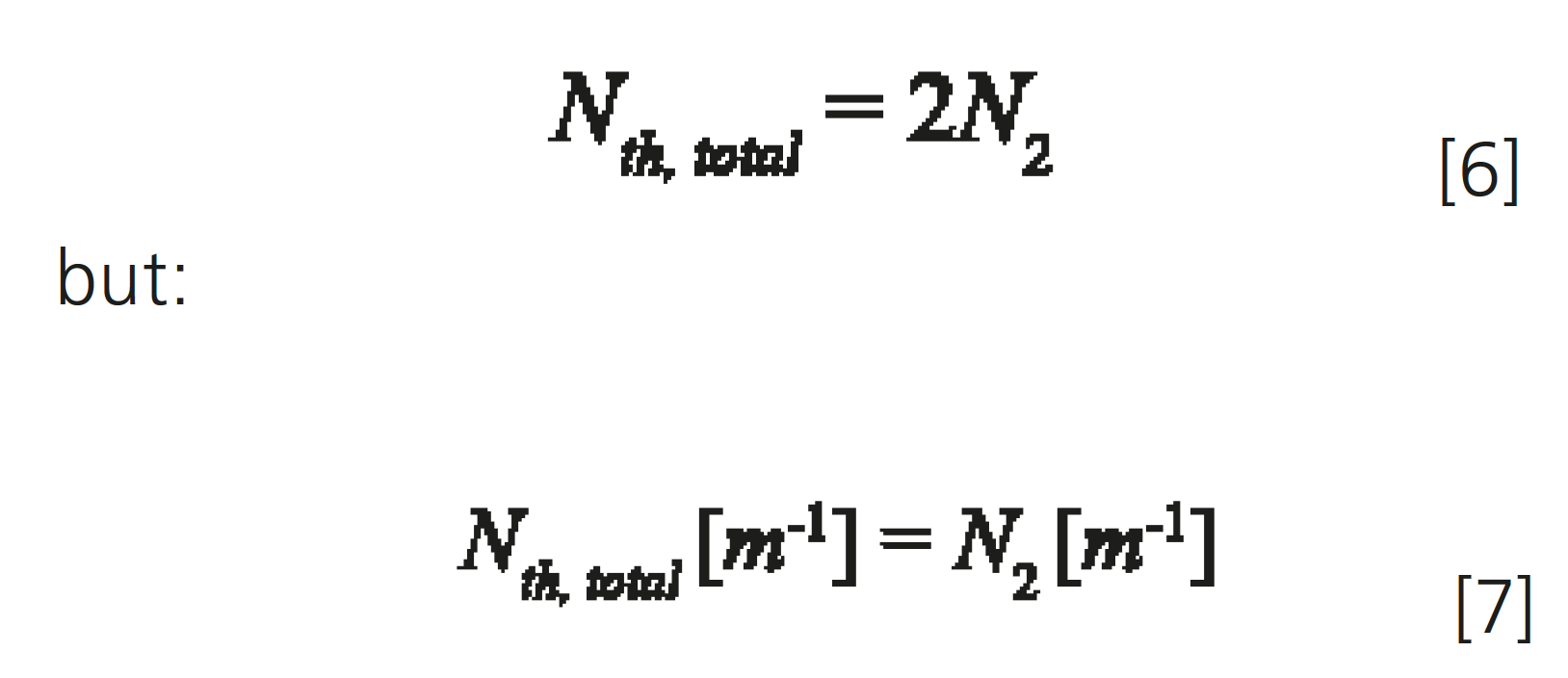
In other words, if the efficiency of one column is much worse than that of the other, the plate count for two columns of identical calibration curves is determined by the plate count of the less-effective column.

Figure 4 shows the dependence of N_total on the plate counts of the less effective column (N₂), for a fixed plate count of the better performing column of N₁ = 50 000 m^-1.

By combining the efficient column (N₁) with a less efficient one (N₂), the plate count of the column combination drops dramatically with decreasing plate count _N2, approaching N_total = N₂ for very low values of N₂. The plate count gain with respect to N₂ due to the combination with a more effective column (N₁) however, is very low.

In other words, the relative loss in efficiency of the “good” column significantly exceeds the gain relative to the less efficient column. The plate count of the less efficient column dominates the plate count of the column combination.

By combining a high plate count column with a column of low plate count, the resolution can even be reduced.

If the plate count for the less efficient column (N₂) is lower than N_1/3, the single column with higher plate count (N₁) achieves a better separation in less time.

b) Columns of different pore size distributions

The above calculations describe identical columns (L, D, V_P) differing only in the number of theoretical plates.

However, in GPC/SEC it is common practice to combine columns with different pore size distributions to extend the separation range.

In these instances, V_P1≠V_P2 and the plate count of the column combination does not only depend on the plate counts of the two columns, but also on the different elution volumes of the analyte in the two columns. Denoting the elution volume contribution of the better performing column γ = V_P1 ⁄ (V_{P1 +} V_P2), and using typical values for the pore volumes of GPC columns the dependence of N_th,total ⁄ N₁ on γ and on the ratio N₂ / N₁ can be calculated and shown in Figure 5.

The number of theoretical plates of the column combination is always significantly lower than the plate count of the more effective one, that is, N_total ⁄ N₁ <1. Thus, by combining columns of different plate counts the high plate count of the more effective column is strongly sacrificed as already mentioned in the case of combining columns of identical V_P. This reduction in plate count is particularly strong, when the peak has a lower elution volume on the less effective column than on the more effective column (γ < 0.5).

Such situations occur when combing a large pore size column of large particle size with a small pore size column of small particle size. Those combinations are sometimes applied when trying to achieve oligomer separations while maintaining a large separation range. However, for such combinations the oligomer separation that can potentially be achieved by the small pore size column is sacrificed by adding the large particle size column to expanding the separation range.

Conclusions

Particle size influences the column plate count and thus the performance of a column.

By combining a highly efficient column (small particle size) with a less efficient column (large particle size), the plate count of the column combination is mainly determined by the less efficient column.

When combining two columns of identical separation range (pore size distribution), enhancement of resolution can only be achieved if the plate count of the less effective column is at least 1/3 the plate count of the more effective one.

Combining a highly effective column for oligomer separations with a less effective column for high molar masses significantly reduces the plate count for oligomers, resulting in loss of oligomer resolution.

References

1. D. Held and K. Oleschko, The Column 16(1), 8–12 (2020).
2. A.M. Striegel, J.J. Kirkland, W.W. Yau, and D.D. Bly, Modern Size-exclusion Liquid Chromatography (John Wiley & Sons, Inc. New Jersey, 2nd ed., 2009).
3. D. Held, The Column 9(22), 21–25 (2013).
4. D. Held, The Column 7(2), 14–16 (2011).
5. P. Kilz, in Column Handbook for Size Exclusion Chromatography, C.-S. Wu, Ed. (Academic Press, 1999), Chapter 9.
6. F. Gores and D. Held, The Column 10(14), 7–10 (2014).

Daniela Held studied polymer chemistry in Mainz (Germany) and is working in the PSS software and instrument department. She is also responsible for education and customer training.

Wolfgang Radke studied polymer chemistry in Mainz (Germany) and Amherst (Massachusetts, USA) and is head of the PSS application development department. He is also responsible for instrument evaluation and for customized trainings.

E-mail: DHeld@pss-polymer.com
Website: www.pss-polymer.com