Retention Time Drift—A Case Study

What should you do if your retention times drop from one injection to the next? A systematic approach to troubleshooting can help to quickly identify the problem source.

For this month’s “LC Troubleshooting,” I’ll look at a problem related to retention time drift that was submitted by a reader. As usual, I will slightly obfuscate the question so the source is difficult to identify to protect proprietary information. If you have a liquid chromatography (LC) question or problem that you’d like help with, feel free to e-mail me at the contact listed at the end of this article. Be aware, though, that I’m not volunteering to do your work for you-rather to help you solve a particular problem or to point you in the right direction to find answers.

Retention Time Drift

Reader Question

I was setting up the LC system for analysis and the usual retention times I get for my current project are 5.2 and 5.4 min. The areas for my standards were satisfactory, but the retention times had decreased, with the two standards now eluted at 4.9 and 5.1 min. I made a second run to confirm the times, and further retention shifts to 4.5 and 4.6 min were observed. A final run was made; this time retention was 4.2 and 4.3 min.

At first I thought the problem was caused by air bubbles in the system, but I realized that would affect the flow rate and cause the retention time to increase. What other factors could have caused the decreased and inconsistent times?

I’m using a type-B, high-purity C18 column (150 mm x 4.6 mm, 5-µm particles) with the column oven set at 30 °C. The mobile phase is 40:60 0.1 M ammonium acetate (pH 4.0)–acetonitrile at a flow rate of 2.0 mL/min. I’m injecting 100 µL of sample diluted in 30:70 water–methanol. The run time is 8 min.

My Response

At a first glance, nothing pops out at me as a major problem here, so let me share how I proceed through the problem-solving process and maybe we can find the root cause. There is no single best way to approach a problem, and my strategy will vary a bit from problem to problem, but there are some common steps to follow. I’m basically lazy, so I tend to favor the “easy versus powerful” approach-that is, I’ll do an easy mental experiment first, even though a more powerful approach might be to run several controlled experiments in the laboratory. In addition, I don’t have the option of doing real experiments for this problem, so I have to work with the data presented. Note that I am assuming that the method has worked well in the past (“the usual retention times . . .”) and is not a new method under development.

Are Retention Factors Reasonable?

First of all, I like to check the overall health of the method. I’ve seen so many problems where the method has been created only with speed in mind, which can result in retention times that are so small that the sample does not have adequate time to interact with the column properly. This is highlighted by retention factors, k, that are too small. For an isocratic method like this, it is desirable to have all the peaks of interest eluted in the range 2 < k < 10, but if this is not possible, 1 < k < 20 usually is acceptable. When the ratio of last–first k values exceeds 20–30, a gradient usually will be required.

Recall that the retention factor (also called the capacity factor) is calculated as follows:

where t_R is the retention time and t₀ is the column dead time (also abbreviated t_M). For the current method, 4.2 ≤ t_R ≤ 5.4 min. The dead time is the time it takes one column volume of mobile phase to pass through the column. The internal volume of a 4.6-mm i.d. column can be estimated (in milliliters) as 1% of its length (in millimeters), so the 150 mm x 4.6 mm column contains approximately 1.5 mL of mobile phase. At 2 mL/min, this means t₀ ≈ 1.5 mL/2 mL/min = 0.75 min. Now we can estimate k for the 4.2-min peak with equation 1: (4.2 – 0.75)/0.75 = 4.6. Similarly, for the 5.4 min peak, k = 6.2. Both of these are well within the 2 < k < 10 target, so it does not look like retention is the problem per se.

Are the Injection Conditions Reasonable?

A second preliminary check that I like to make relates to the injection conditions. Too often I encounter injection conditions that work acceptably, but are not very robust. As a result, some small change in external conditions may degrade the system enough that the injection conditions are no longer appropriate. In particular, I like to make a quick check to be sure the injection volume and injection solvent conditions are reasonable. Injecting too large a volume of sample in too strong of a solvent can affect retention times (as well as peak shape). The guideline I like to use is that if the sample is injected in mobile phase, you can safely inject 15% of the volume of the first peak of interest. Let’s see how to check this. To do this, we need to estimate the volume of the first peak of interest (or if we had a chromatogram in front of us, we could measure it directly).

The peak volume can be estimated from the column plate number, N. A reasonable estimate of N for real samples can be made as follows:

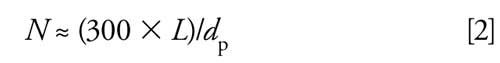
where L is the column length (in millimeters) and d_p is the particle diameter (in micrometers). For the current column we get N ≈ (300 x 150)/5 = 9000. The peak width at baseline, w_b, can be determined by rearranging the plate number equation:

to

For the 4.2 min peak, w_b = (4 x 4.2)/9000^0.5 = 0.18 min. Convert the width to a volume of 0.35 mL = 350 µL by multiplying by the flow rate (2.0 mL/min). (My usual warning applies here: If you repeat my calculations, your results may vary slightly, because I’ve rounded or truncated values for simplicity in presentation.)

Now we can compare the actual injection volume (100 µL) to the desired volume (<15% of the peak volume). The target injection volume would be no more than 350 µL x 15% ≈ 50 µL if the sample is injected in mobile phase. The injection solvent is 30:70 water–methanol, whereas the mobile phase is 40:60 buffer–acetonitrile. Acetonitrile is a bit stronger of a solvent than methanol, so 60% acetonitrile ≈ 70% methanol in terms of elution strength in reversed-phase LC. Although the injection solvent and mobile phase aren’t matched, their relative strength is not very different, so I don’t think the injection solvent is a problem. Ideally, the injection solvent should be buffered to match the mobile phase, but with a mobile phase buffer of 100 mM, sample buffering upon injection should be fast and adequate.

The use of an injection volume that is larger than desired (100 µL versus 50 µL) and the slight mismatch of injection solvent and mobile phase may increase peak widths, resulting in lower plate numbers, but I don’t expect major retention time problems as a result of this combination. Therefore, it is unlikely that injection conditions are the source of the problem, especially because the method seemed to work properly in the past. If I were to modify the method, I would reduce the injection volume and reduce the injection solvent strength. I would also match the injection solvent components to the mobile phase (buffer and acetonitrile) rather than using no buffer and methanol for injection.

What Can Cause Retention Changes?

Next, as requested by the reader, let’s consider the major factors that can affect retention times:

·      flow rate

·      column temperature

·      column chemistry

·      mobile-phase composition

Each of these conditions can influence retention. Let’s look at each of these parameters briefly to see if it is reasonable to suspect one of them over the others.

Flow Rate

Retention in isocratic separation is inversely related to flow rate. That is, if the flow rate is increased, the retention time will decrease. Because the observed retention times decreased, if a flow rate change were the causative factor, flow would need to increase. Air bubbles in the pump and leaks are the most common causes of flow rate changes. But, as the reader concluded, bubbles in the pump would serve to reduce the flow rate and thus increase retention-the opposite of the observed problem. The same conclusion can be made about leaks. The only way I have ever seen the flow rate increase is if the pump setting is changed. Although it is conceivable, a software failure of some kind could increase the flow rate, but I’ve never heard of this happening. So I have to agree with the reader: This is not a flow rate (or leak) problem.

Column Temperature

The temperature of the column will influence retention. Increased column temperature will reduce retention times and decreased temperatures will increase them. A good rule of thumb for isocratic reversed-phase LC separations is that retention changes approximately 2% for each 
1 °C change in column temperature. Because the observed retention times decreased, if temperature were the problem, temperature would have to increase. The retention of each of the two standards decreased by ~20% (5.2 to 4.2 min and 5.4 to 4.3 min) in the reported data. Using our 2%/1 °C rule of thumb, this would have required ~10 °C increase in temperature. Is this a likely situation? I don’t think so. The column oven was in use, so even an extreme change in room temperature of 10 °C would be mitigated through automatic adjustment of the oven controller. If the oven had inadvertently been shut off or had failed during the experiments, the temperature would have gone down, not up. If the oven had been off when the experiments started and the user realized the problem and turned on the oven or if the oven had not been allowed to equilibrate properly before starting, the oven temperature could have increased 10 °C. This, however, could not be the case, because the normal retention times were 5.2 and 5.4 min, so the observation would have started with larger retention times that gradually decreased to these values. The only way I can think of the oven causing the retention problem is if the temperature setting were wrong (and had not equilibrated before starting) or if there were some kind of controller failure. Neither of these scenarios are likely, but they could be checked easily in the laboratory. My conclusion is that column temperature is not the root cause of this problem.

Column Chemistry

The chemistry of the bonded phase and silica support that comprise the column packing certainly influence retention. Changes in retention with column chemistry changes can happen in two ways: step changes and gradual changes. Most of you have seen examples of both of these situations during routine LC operation. Step changes can happen when an old column is replaced by a new one. Although manufacturers of today’s high-purity C18 columns have very good control of column to column variation, it isn’t perfect. So, it is not unusual to see retention change slightly when a new column is installed, but this rarely exceeds a few percent change. The reader did not mention that the observed problems were preceded by a change in the column, and even if the column were changed, I would not expect the magnitude of retention shifts observed or that they would continue past the first few injections. Again, this is an easy problem to check-was the column recently replaced?

A gradual change in the column chemistry is much more common in reversed-phase LC. Over the life of the column, generally 500–2000 or more injections, retention times often will drift a bit. The drift is caused by either gradual loss of the bonded phase or gradual buildup of contaminants on the column surface, or a combination of the two. As a result, such changes typically take place over hundreds of injections, not three or four consecutive injections as observed here. And such changes are more common with real samples, not the reference standards injected in the present case. More rapid deterioration of the bonded phase or silica support can happen if the mobile-phase conditions are extreme, but nearly all modern, high-purity C18 columns are all stable in the 2 < pH < 8 range, so the pH 4.0 mobile phase is unlikely to be attacking the column. I think we can rule out the column as the problem source.

Mobile-Phase Composition

The most significant influencing factors on retention that are contributed by the mobile phase are the buffering conditions, the type of organic solvent, and the percent organic (%B) in the mobile phase.

The buffer should be formulated in its normal buffering range and should be sufficiently strong to adequately buffer the column, the sample, and the injection conditions. The best performance of a buffer is within 1 pH unit of its pK_a. Ammonium acetate, the present buffer, has a pK_a of 4.8, so the pH 4.0 value of the buffer is well within its accepted limits. For conventional LC separations, I usually suggest starting in the 20–30 mM range for the buffer, and the 100 mM concentration here is close to that when diluted 40:60 with acetonitrile, as in the present case. And, as mentioned earlier, although the injection solvent is not buffered, the mobile-phase buffer should be strong enough to correct any pH shifts immediately upon injection. Furthermore, if the method had been working well in the past, it is unlikely that a buffering problem would suddenly show up if the pH and concentration are reasonable. It is unlikely that the buffer is the cause of the present problem. However, if the buffer were prepared improperly, it is possible that buffering could contribute to retention problems. Reformulation of the buffer, paying careful attention to the weighing, dilution, and pH adjustment, should answer any questions in terms of buffer preparation errors.

The type of organic solvent in the mobile phase, acetonitrile, has not changed and is one of the two most common organic solvents in use for reversed-phase LC, so it is unlikely the source of the present problem. More important is the concentration of organic (%B) in the mobile phase. The rule of 2.5 (often simplified to the rule of 3) estimates the influence of %B on retention in reversed-phase separations for small molecules (~500 Da). This states that retention will change approximately 2.5-fold for a 10% change in organic concentration (1). Or stated mathematically as

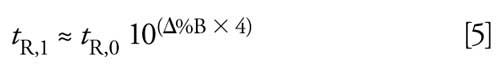
where t_R,1 and t_R,0 are the new and original retention times, respectively, and Δ%B is the change in %B as a decimal (10% change in B = 0.1). From equation 5, you can determine that a 2% change in organic concentration can change retention by ~20%-the approximate change in retention observed in the present case. This cause seems to be the most reasonable source of the present problem.

How could %B change by 2%? The reader didn’t state how the mobile phase was formulated, but I suspect that it is prepared using on-line mixing. This is the most common technique to formulate mobile phases. If some system problem were present that caused the mobile phase mixture to change over time, the observed results might be explained. The easiest way to check this is to hand-mix the mobile phase to 40:60 buffer–acetonitrile and then pump it from a single reservoir. If the retention times stabilize, this step will confirm that the mobile-phase blending is the problem source.

What kind of failure would cause mobile phase proportioning to drift? I suspect the problem is related to the buffer and the buffer reservoir conditions. Using 100 mM ammonium acetate is a very good growth medium for bacteria-it has plenty of nitrogen and carbon, and a pH of 4.0 is conducive to growth. As bacteria grow, they can gradually restrict flow through the mobile-phase inlet frit in the reservoir. With a low-pressure mixing system, proportioning valves for the buffer and organic alternately open to allow a pulse of buffer (A) and then a pulse of organic (B) to be drawn in by the suction of the pump. If the flow of A is restricted by a partially blocked frit, the deficit will be made up with extra B to satisfy the demand of the pump (2.0 mL/min in the present case). Extra B-solvent (acetonitrile) will mean a stronger mobile phase and shorter retention times. A change of just 2% in the mobile-phase ratio could cause the observed problems.

I recommend several practices to minimize the risk of problems related to bacterial growth in the buffer. First, change the buffer regularly. In our laboratory, we had a policy to replace the buffer once a week, although I know many laboratories that use buffer for much longer than this. Second, never refill a buffer reservoir. Instead, use the buffer that is in it, then replace the reservoir with a clean one. By “topping up” the reservoir with fresh buffer, you are adding more food for the colony of bacteria that has started growing in the previous buffer. If you have previously been refilling a reservoir, the frit may already be contaminated, so I would replace it with a new one. I would also perform a “siphon test” to ensure that there are no restrictions in the solvent inlet tubing. To do this, disconnect the tubing at the inlet to the proportioning valve and allow the buffer to flow through the line by siphon action. If the frit (and associated tubing) is not blocked, buffer should flow freely through the tubing. I like to see ~10 times as much siphon flow as I’ll ever need at the pump; in the present case of a 2.0 mL/min, I’d like to see at least 20 mL/min of siphon flow. If the flow is significantly restricted, replace the frit or connecting tubing.

Summary

I’ve used a reader’s problem with drifting retention times to illustrate how to approach a problem of this nature. We found that the rather limited data set still allowed us to examine the method and eliminate many potential causes of the problem. As potential problem sources were discarded, we gradually were pointed to the composition of the mobile phase as the most likely root cause of the problem. Now we are left with a few laboratory experiments to help confirm the problem and correct it. Although this discussion centered on a specific problem, the troubleshooting pattern is useful for a wide variety of LC problems.

Reference

• L.R. Snyder, J.J. Kirkland, and J.W. Dolan, Introduction to Modern Liquid Chromatography, 3rd edition (Wiley, Hoboken, New Jersey, 2010), p. 58.

John W. Dolan “LC Troubleshooting” Editor John Dolan has been writing “LC Troubleshooting” for LCGC for more than 30 years. One of the industry’s most respected professionals, John is currently the Vice President of and a principal instructor for LC Resources in Lafayette, California. He is also a member of LCGC’s editorial advisory board. Direct correspondence about this column via e-mail to John.Dolan@LCResources.com