LC Method Scaling, Part I: Isocratic Separations

What kind of adjustments need to be made when scaling an isocratic method?

Today we are often confronted with many different types of liquid chromatography (LC) methods. These may use conventional 250 or 150 mm × 4.6 mm, 150 mm × 2.1 mm, 50 mm × 2.1 mm, and many other column configurations packed with particles generally ranging from <2 µm to 5 µm, and sometimes even 10 µm in diameter. One of the challenges this variety presents is transferring a method from one column configuration to another and still obtaining the same resulting separation. For example, you may use an ultrahigh-pressure LC (UHPLC) system to develop methods quickly in your research and development (R&D) laboratory, but want to transfer it to a conventional LC system for routine use. Or your conventional method with ultraviolet detection (LC–UV) may need to be transferred to an LC system with mass spectrometry detection (LC–MS). Alternatively, you may want to adjust a pharmacopeial method to use a different column configuration. In many of these cases, the method must be moved from one column size to another, yet maintain the same separation. The conversion is not difficult, but you do have to be careful to make the appropriate adjustments. Isocratic separations, in which the mobile-phase concentration is constant, are simpler to convert than gradient methods, where special care has to be taken to avoid inadvertent chromatographic changes. This month's "LC Troubleshooting" discussion focuses on isocratic separations, and next month we'll look at gradients.

Resolution Is the Key

Equivalent separations require that the resolution stays the same when conditions are changed. In the method development classes we teach, we use what is often referred to as the "fundamental resolution equation" as a guide for the method development process. We can use this same equation to guide us in method conversion:

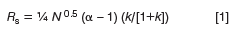
where R_s is resolution, N is the column plate number, α is the separation factor, and k is the retention factor. The first caveat is that the chemistry of the system cannot change when a change in the column or other conditions changes. This means that the mobile phase must remain the same, as well as the column temperature and column chemistry. With today's columns, it is usually valid to assume that the same brand name description of a column (for example, ACE C18 [Advanced Chromatography Technologies Ltd.]) will have the same column chemistry, no matter what the particle size is (2, 3, 5 µm, and so forth). You'll recall that the retention factor is defined as:

where t_R is the retention time of a solute and t₀ is the column dead time (retention time of an unretained peak). If we keep the chemistry of the system constant, the retention time relative to the dead time should stay constant, so k will remain unchanged. Any change in retention because of a change in column length, diameter, or flow rate will have a proportional change for t_R and t₀, so k will stay constant in this case as well. For example, doubling the flow rate will halve t_R and t₀, and k will be unchanged.

The separation factor α is simply the ratio of k values for two adjacent peaks, k₁ and k₂:

So if we keep k constant, as discussed above, α will be unchanged. If k and α are kept constant, to keep R_s constant (equation 1), all that remains is to make sure that the column plate number stays constant.

Keeping the Plate Number Constant

Fortunately, the relationship between the column plate number, N, the column length, L, and particle diameter, d_p, are well defined and the effect of a change in L or d_p can be determined by a simple calculation. And for you purists, yes, flow rate does affect N, but for real samples under real operating conditions, this effect can usually be ignored for a change in flow rate by a factor of two with particles of d_p ≤ 5 µm. N is directly proportional to column length and inversely proportional to particle size, so if L/d_p is kept constant, the plate number should remain constant.

Because the number of available column lengths and particle sizes is somewhat limited, the U.S. Pharmacopeial Convention (USP) (1) suggests that the plate number should be considered equivalent if L/d_p is a constant -25% to +50%. For example, we can consider the following columns to have equivalent plate numbers (all lengths are in millimetres and particle diameters in micrometres):

L/d_p: 150/5 ˜≈ 100/3 ≈˜ 50/1.8

Ratio: (30) (33) (28)

You can see by the ratios shown below each column configuration that each of these columns has a ratio in a range of ±10%, so they can be considered equivalent in terms of plate number. It should be noted that the USP (1) also suggests allowing other combinations of L and d_p to be used as long as N stays within -25% to +50%, as would be the case for core–shell particles, which provide larger plate numbers than their particle diameter might suggest.

Maintaining the Linear Velocity

It is customary to keep the linear velocity (in units of millimetres per second) of the mobile phase constant when the column size is changed. The linear velocity is independent of the column length, but proportional to the cross-sectional area of the column. So,

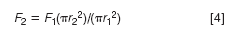
Or

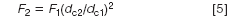
Where F is the flow rate, r is the column internal radius, and d_c is the column internal diameter; subscripts 1 and 2 are for the original and new column, respectively. For a change from a 4.6-mm i.d. column to a 2.1-mm column, equation 5 generates a ratio of (4.6/2.1)² = 4.8 ≈˜ 5. Because this change in diameter is the most common one we encounter, I like to remember the factor of five so I can do the quick mental math. Thus, a 4.6-mm i.d. column operated at 1 mL/min would mean that an equivalent linear velocity would be obtained with a 2.1-mm i.d. column at 0.2 mL/min.

What About Pressure?

Pressure in LC separations is a result of the separation conditions used, and in itself is important only relative to the pressure capability of the instrument. The exception to this generalization is that sometimes selectivity (peak spacing) will change when large changes in pressure occur, such as when switching from conventional LC pressures to UHPLC pressures. Although we generally run conventional LC systems at half to three quarters of their pressure capability, all can generate pressures of 400 bar (6000 psi). UHPLC comprises instruments with a pressure capability of >400 bar, and in some cases up to 1300 bar (19,000 psi), but most workers operate their UHPLC systems at 600–1000 bar (8700–14,500 psi).

Pressure is directly related to column length and inversely related to the column cross-sectional area. It is directly related to the flow rate and inversely related to the square of the particle size. Pressure is also influenced by the mobile-phase viscosity and the column temperature, but for the present discussion, we're assuming that these remain constant so that selectivity is not changed. Combining these factors, we have the following relationship for pressure, P:

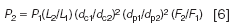
where P₁ and P₂ are the initial and new pressures, respectively. If we want to maintain linear velocity, F₂ will be determined by the column diameter change as in equation 5. If we are not concerned about linear velocity (usually the case for ≤3 µm d_p particles and often for ≤5 µm ones), F₂ may be adjusted to obtain a desired pressure P₂. Examples are discussed below.

And the New Retention Time Is . . .

Many of the changes discussed above will result in a change in the retention time of each peak. Because we're only considering isocratic separations and we have been careful to avoid making any chemical changes to the system, we can calculate what the new retention time will be. We can use an equation similar to equation 6 (but be careful which terms are in the numerator and denominator):

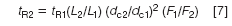
where t_R1 and t_R2 are the original and new retention times, respectively. That is, the same factors affect retention time as those that affect pressure, with the exception that particle diameter does not come into play for retention. An example is included in the discussion below.

For Example

Let's look at two examples. In the first example, let's consider a hypothetical, compendial method that uses a 250 mm × 4.6 mm, 5-µm d_p column operated at 1.0 mL/min, as is typical for many of the older pharmacopeial methods. Let's assume that the retention time of the analyte of interest is 17 min. The current method conditions generate a system pressure of 110 bar (1600 psi), but for the new method we're willing to tolerate 300 bar (4300 psi). To speed things up, let's move the method to a 3-µm d_p column and to save solvent, we'll use a 2.1-mm i.d. column. How do we go about this?

First, to maintain resolution, we want to keep N constant, which means L/d_p should be constant within -25% to +50%. So we can calculate the desired column length and then choose an available one that is close to satisfying this relationship:

or (250 mm × 3 µm/5 µm) = 150 mm. Because 150 mm columns are readily available, the new column's dimensions will be 150 mm × 2.1 mm and it will be packed with 3-µm d_p particles.

Next, we might optionally calculate the flow rate required for a constant linear velocity with the new column. Using equation 5, 1.0 mL/min × (2.1 mm/4.6 mm)² = 0.21 mL/min, so a new flow rate of 0.2 mL/min would keep the linear velocity constant.

Now we can calculate what the new pressure would be using equation 6: 110 bar × (150 mm/250 mm) × (4.6 mm/2.1 mm)² × (5 µm/3 µm)² × (0.2 mL/min/1.0 mL/min) = 175 bar (2550 psi). Because this is an isocratic method, we don't have to be too concerned about linear velocity, so we can increase the flow rate to 0.3 mL/min to speed up the method, which would generate an expected pressure of ~265 bar (3850 psi), well within our desired maximum pressure.

Finally, we can calculate the new retention time using equation 7: 17 min × (150 mm/250 mm) × (2.1 mm/4.6 mm)² × (1.0 mL/min/0.3 mL/min) = 7.1 min. All the other peaks in the chromatogram will change by the same factor, so for a shortcut, use the ratio 7.1 min/17 min = 0.41 as the multiplier to determine the retention times of the other peaks.

As a second example, let's convert an existing conventional method to UHPLC conditions. Our original method uses a 150 mm × 4.6 mm column packed with 5-µm d_p particles operated at 1.5 mL/min. The retention time of the active ingredient is 12 min and the pressure is 150 bar (2175 psi). We want to use a 1.7-µm column with a maximum pressure of 1000 bar (14,500 psi).

First, find the desired column length with the help of equation 8: 150 mm × 1.7 µm/5 µm = 51 mm, so we'll use a 50 mm × 2.1 mm column packed with 1.7-µm d_p particles. For the flow rate conversion, let's just use our factor of five for the 4.6 mm to 2.1 mm i.d. change. This gives us (1.5/5) = 0.3 mL/min as a starting flow rate with constant linear velocity.

The pressure calculation, using equation 6, gives a new pressure of 415 bar. Because we can tolerate up to 1000 bar, we can increase the flow rate to 0.7 mL/min and have a predicted pressure of 970 bar. The predicted retention time using equation 7 will be reduced from 12 min down to 1.8 min. The retention times for the remaining peaks will change by a factor of 1.8/12 = 0.15.

In both of these examples, our next step would be to run the desired new conditions and observe what happens. Because we expect the chemistry to be the same and the same plate number was chosen, the resolution should remain the same. The retention times should also be close to the calculated values. The observed pressure will likely deviate from the calculated one somewhat — for example, because of the additional pressure generated by very small tubing diameters used for UHPLC.

A Simpler Way

The calculations above are not difficult, but they can be tedious to perform on a routine basis. You can do as I've done and put the equations into an Excel spreadsheet, making the calculations simple. Some of the data system software packages now have method conversion calculators built in. Alternatively, there are several of these calculators that are free on the internet. Just use a search term such as "HPLC method transfer calculator," and you will find several choices. I used one of these to double-check my calculations.

Stay tuned for next month's "LC Troubleshooting," where we'll extend the current discussion to include scaling gradient methods.

John W. Dolan is vice president of LC Resources, Walnut Creek, California, USA. He is also a member of LCGC Europe's editorial advisory board. Direct correspondence about this column should go to "LC Troubleshooting" LCGC Europe, 4A Bridgegate Pavilion, Chester Business Park, Wrexham Road, Chester, CH4 9QH, UK, or email the editor-in-chief, Alasdair Matheson, at amatheson@advanstar.com

Reference

(1) General Chapter 621 "Chromatography, Pharmacopeial Forum PF38(2)" in United States Pharmacopeia 35–National Formulary 30 (United States Pharmacopeial Convention, Rockville, Maryland, 2012), www.usppf.com.