Is Golay’s Famous Equation for HETP Still Relevant in Capillary GC? Part 1: A Common View of HETP

In this instalment of “GC Connections”, we take a close look at Golay’s famous equation that most people view as relating height equivalent to a theoretical plate (HETP) to the carrier gas flow rate or average linear gas velocity in a capillary column. We explore the original works of Golay and Keulemans. We also look closely at the original equation, the assumptions involved with its development, and the assumptions (both good and bad) that have been applied to HETP over the years. In part two, we will explore the consequences and relevance of this theory today, with temperature programming, very long (and very short) columns, high pressure drops, and vacuum outlet detectors.

The height equivalent to a theoretical plate (HETP) is one of the most important parameters used to measure column performance. HETP is the length of column required to generate one theoretical plate, with a theoretical plate being one transfer process of an analyte molecule between the mobile phase and the stationary phase. We are all familiar with considering the number of theoretical plates in a column or the number of theoretical plates in a length of column, as these are often stated by column vendors as a measure of column quality. More theoretical plates and more theoretical plates per metre are often considered good, leading to better resolution. A 30 m column with 100,000 theoretical plates is said to have 3333 theoretical plates per metre.

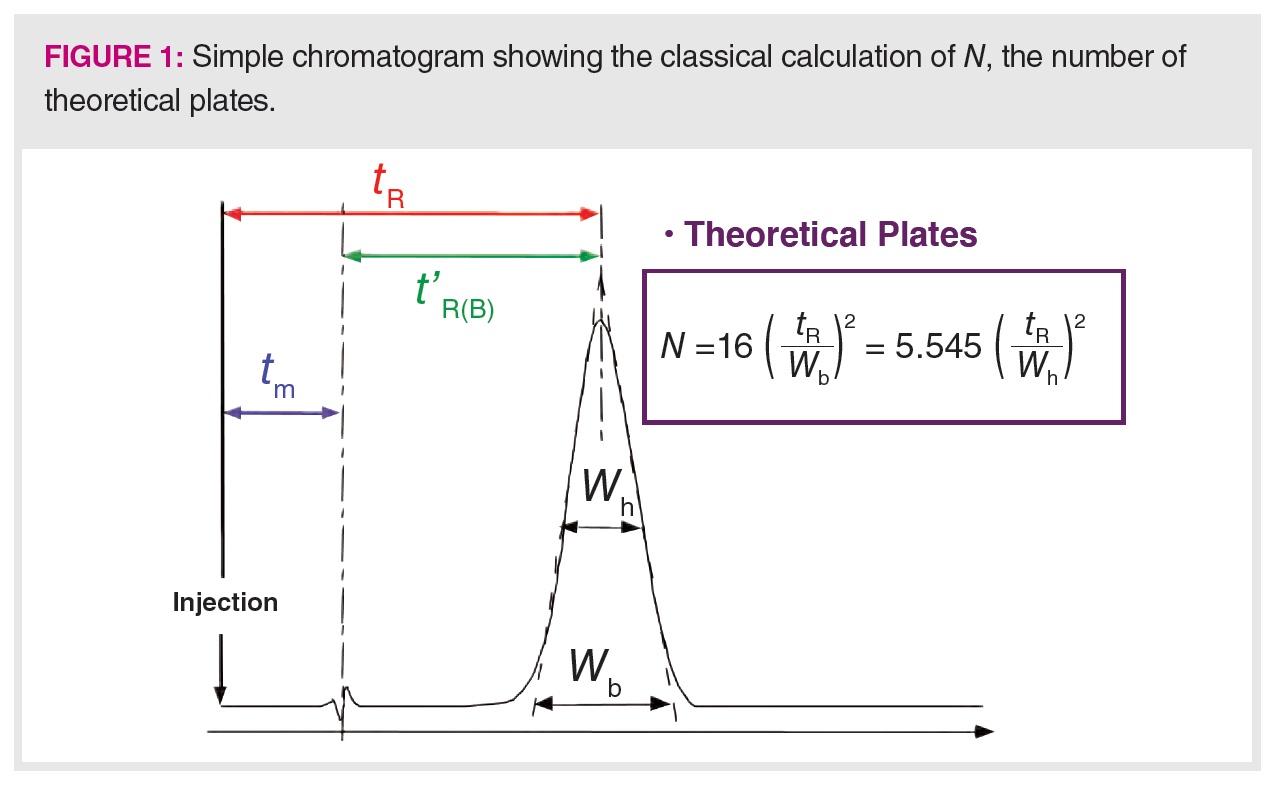
We use a chromatogram to measure theoretical plates (as shown in Figure 1), using equation 1, where N is the number of theoretical plates, t_R is the retention time of the peak and W_b is the peak width at the baseline. N and HETP (as discussed later) are dependent on many parameters, including temperature, carrier gas flow rate, inlet and outlet pressures, column dimensions, and the choice of carrier gas. One challenge in comparing plate numbers between different columns is that the experiments will almost always have been performed under different conditions, making true comparisons difficult.

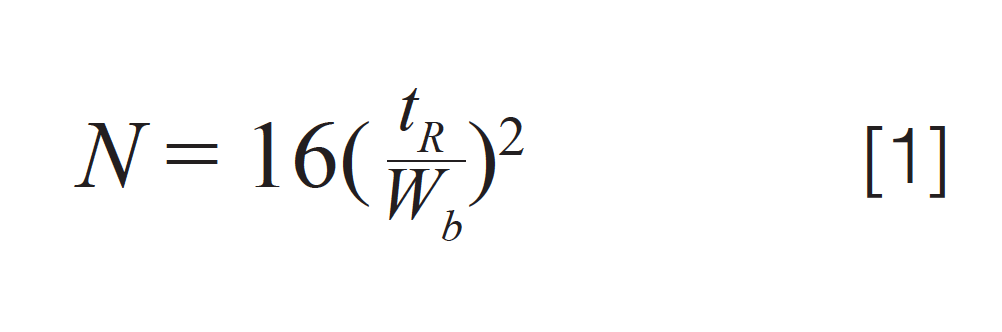
HETP is then calculated using equation 2, where L is the column length and N is the number of theoretical plates calculated using equation 1:

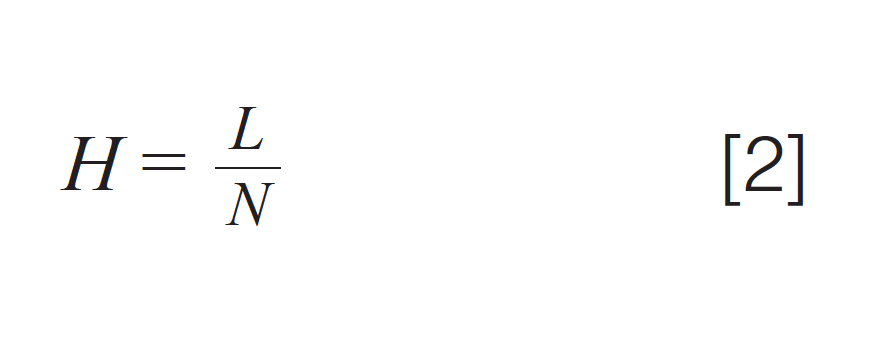
As we will discuss in detail shortly, H, or HETP, is also defined as the rate of band broadening. As we know, the peaks or bands get wider in all chromatographic techniques as the peaks and bands traverse the length of the column. HETP provides a measure of the rate of the broadening, with a smaller HETP indicating slower band broadening, leading to sharper peaks.

Origins of Golay’s Famous Equation

How was HETP as a measure of the rate of band broadening originally defined? This definition goes back to the earliest days of gas chromatography (GC) in the 1950s and the work of A.J.P. Martin and A.T. James, the inventors of GC; J. Van Deemter, who developed the original theory of band broadening for packed columns; Golay, who developed the theory for capillary columns; and Keulemans, who provided a fundamental conceptual definition for a theoretical plate.

Keulemans presented a picture of the separation process in chromatography in a classic text, Gas Chromatography, published in 1959 (1). In the foreword to this short text, A.J.P. Martin described it as “an admirably clear account of all the practical and theoretical aspects of this rapidly growing method”. The clear influence of the text is seen in the highly recognized 1965 text, Basic Gas Chromatography, by McNair and Bonelli (2).

As illustrated in Table 1, Keulemans described each theoretical plate as a liquid–liquid extraction between immiscible solvents. Starting with a flask containing a solution of an analyte dissolved in a solvent, say, water. The solution is mixed with an equal volume of hexane. If the partition coefficient is 1, then equal masses of solute are present in each phase. The hexane layer is then removed and mixed with an equal volume of water in a second flask and fresh hexane is added to the first flask. Each flask now contains half of the original analyte, equally distributed between the two phases. This process can be repeated with a third, fourth, fifth, and up to any number of flasks, ultimately resulting in a distribution of analyte among all the flasks (seen in Table 1), calculated for 10 flask theoretical plates, 10,000 molecules, and a distribution constant between the two phases of one.

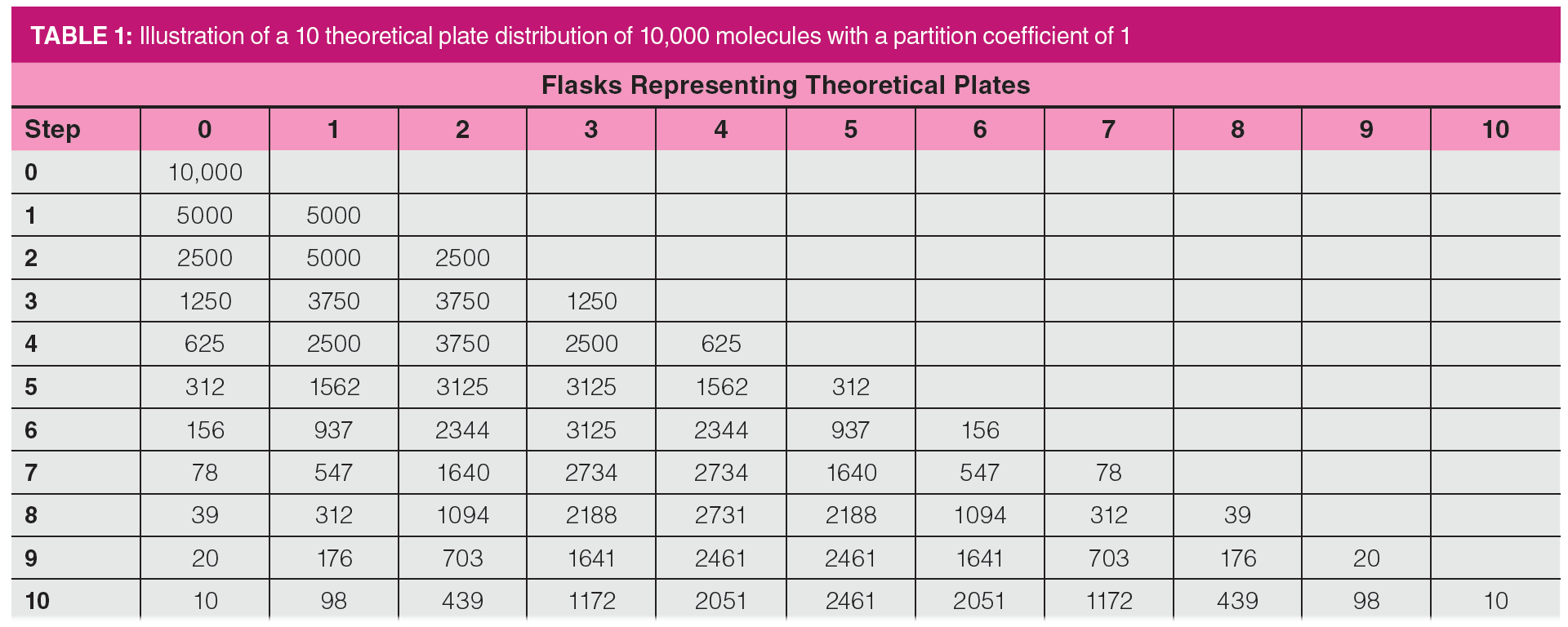
The numbers in Table 1 represent the number of molecules out of the 10,000 in each flask or theoretical plate. We can note immediately that as the number of flasks increases, the number of molecules in each flask decreases, analogous to the chromatographic peak becoming broader and shorter. Also note that there are still some molecules in the initial flask, even after 10 extractions, an often-unappreciated consequence of chemical equilibrium: There are always some molecules left behind.

The description provided above makes several assumptions that, although illustrative of the chromatographic process, do not account for all processes that occur in real columns. First, it assumes that each theoretical plate occurs independently and has no direct contact with the other theoretical plates. Obviously in a column with a mobile phase, this assumption cannot be true as the mobile phase is continuously transporting solutes along the column. Second, it assumes that there are no barriers to mass transfer at the phase interfaces. In a real system, of course, the kinetics of mass transfer across the phase interfaces must be considered. Finally, there is no consideration of diffusion both within and between the two phases. In a column, the solute molecules distribute throughout the mobile and stationary phases. Golay’s equation, described below, uses a description of the chromatographic process like Keulemans, and accounts for these assumptions, plus the dimensions and physical conditions observed within columns.

The “Golay Equation”

Golay provided a thorough description of an equation describing the rate of band broadening in the proceedings of the “Gas Chromatography 1958” symposium organized by the Hydrocarbon Research Group of the Institute of Petroleum and the Koninkiljke Nederlandse Chemische Vreniging in Amsterdam, The Netherlands, in May 1958. The symposium volume was published in 1959, edited by Desty (3). The equation for circular capillary columns is presented as equation 3:

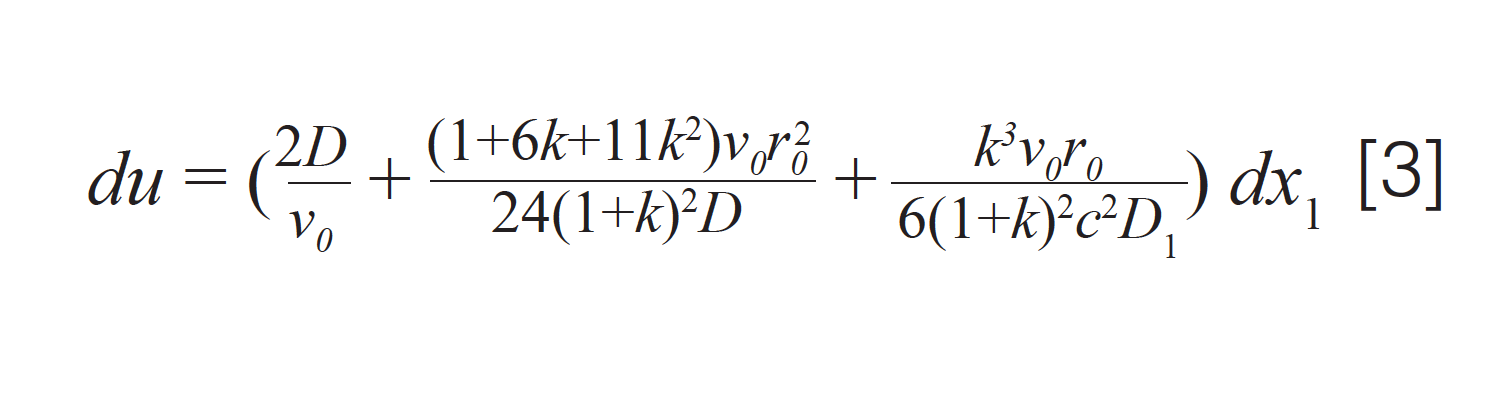
Note that Golay also described a similar equation for rectangular capillaries. At the time, there was considerable discussion about which would be better, and clearly circular capillaries have become far more popular. Interestingly, with recent interest in columns etched into silicon wafers for “laboratory on a chip” applications, rectangular capillaries are increasing in usage and importance. This equation is popularly called the “Golay Equation”, but it combines the work of many early researchers in chromatography.

Definitions of the many variables are discussed in the “deeper dive” below. Interestingly, the proceedings volume includes commentary following the formal text of the talk, in which substantive discussion among the symposium participants was captured. The names of those participating in that discussion read like an all-star lineup of modern chromatography inventors, including Golay, A.J.P. Martin, R.P.W. Scott, I.G. McWilliam, and others. It was traditional in the past to assign scribes to record the discussions. In one especially important exchange between Golay and Martin, they appear to agree, possibly begrudgingly, to use the definition of HETP presented by Keulemans and illustrated above (4).

In the original work, several related forms of the equation are presented, relating to specific situations that may occur in the making of capillary columns; the form used here is equation 31a from that work, chosen for simplicity in discussing the basic principles and consequences. The first thing to note is that this is a differential equation relating to du, a differential of peak width to dx, a differential of length or distance. The terms enclosed in the parentheses provide what we term as HETP or H, the height equivalent to a theoretical plate, so the equation could be conceptually simplified to equation 4. Interestingly, similar to the Keulemans description, the independent variable is the length of the column (dx), as the differential is distance and the dependent variable is the peak width, represented by the differential of the second statistical moment (du).

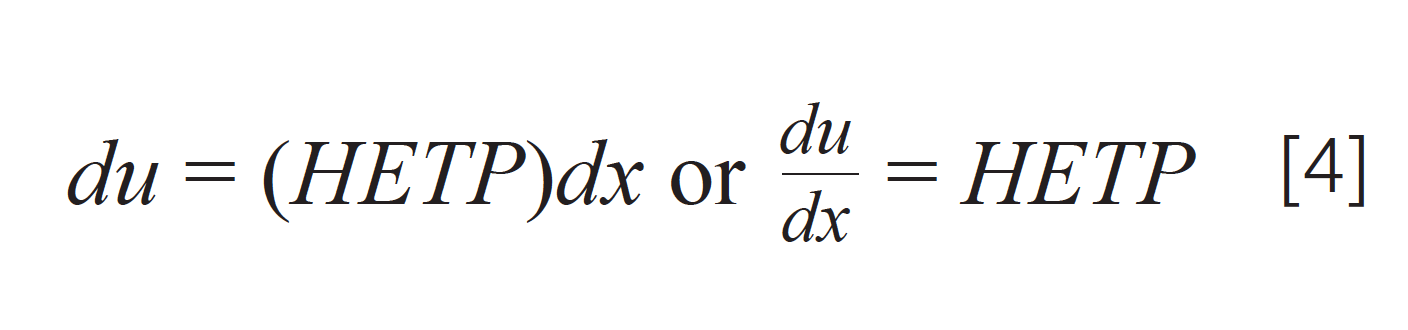
Equation 4 shows us that the rate of band broadening as the band traverses the column is equal to HETP. As we have learned in short courses and textbooks, smaller HETP means a slower rate of band broadening and sharper peaks. In many textbooks and courses, the terms within the parentheses in equation 3 are simplified to the form shown in equation 5. As we will see in Part 2 next month, equation 5 is an oversimplification that can generate confusion and possibly lead to false directions in method development and optimization.

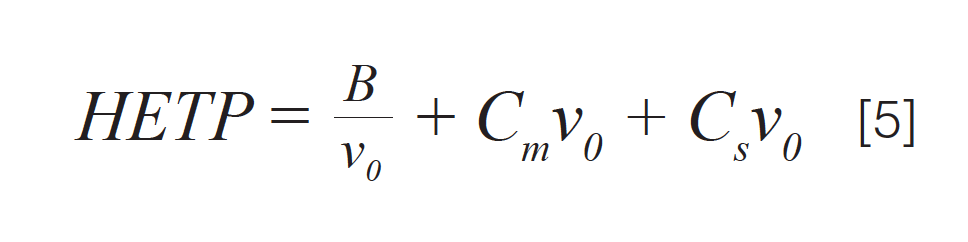
Note the average velocity of carrier gas (v₀) is usually chosen as the independent variable in equations 4 and 5, leading to the “van Deemter” or “Golay” plots often seen in discussions of column performance in the scientific literature. The three terms are often described as representing analyte diffusion in the mobile phase (the “B” term), analyte diffusion in the stationary phase (the “C_s” term), and analyte diffusion in the mobile phase (the “C_m” term). In developing equation 3, Golay noted the similarity in form to the equation of van Deemter, developed a few years earlier.

A Deeper Dive into Golay’s Equation

Whether we perform detailed calculations based on it or not, equation 3 provides a very useful description of the many variables and processes relating to band broadening and, ultimately, to peak widths and resolution. Some important general principles are discussed here; for a detailed description of all variables and how they are used to evaluate column performance, see these book chapters or the discussion in ChromAcademy, LCGC’s online chromatography learning platform (5–7). In short, to minimize HETP, the quantities in parentheses in equation 3 should be minimized.

In the first term within the parentheses, often called the “B” term using the terminology in equation 5, diffusion in the mobile phase is considered. The numerator, D, is the diffusion coefficient of an analyte molecule in the gas phase, which for simplicity can be considered a constant for that analyte at a given temperature in a given carrier gas. Diffusion coefficients in the gas phase are mainly dependent on the identity of the analyte, the solvent gas, and the temperature. The denominator is the average carrier gas velocity in the column. Not surprisingly, this suggests an inverse relationship between HETP and average carrier gas velocity.

The second term, often abbreviated as C_m, relates to mass transfer, also in the mobile phase, and is the major difference between Golay’s equation for capillary columns and the original van Deemter equation for packed columns. Capillary columns usually have much larger phase ratios (volume of mobile phase divided by volume of stationary phase) than packed columns, so mass transfer in the mobile phase becomes important. Note that in this term, the same diffusion coefficient described above is now in the denominator and the average carrier gas velocity (v₀) is in the numerator, suggesting a proportional (linear) relationship between HETP and carrier gas velocity.

Additional variables in the Cm term include the square of the column radius, r², and a complex polynomial of the retention factor (k). Note that all of the variables, except for the column radius are temperature dependent and that the retention factor is also related to the column dimensions and average linear gas velocity.

The third term, C_s, relates to mass transfer in the stationary phase. Similar to the Cm term, the C_s term is linear with the average carrier gas velocity and the square of the column radius (v₀ and r₀²). Also, similar to the C_s term, the denominator includes a diffusion coefficient, this time in the liquid phase, which depends on the specific analyte, liquid phase, and temperature. Additionally, the analyte partition coefficient (c) into the liquid phase is part of the denominator. The term also includes a polynomial function of the retention factor (k).

Taken together, the three terms of the expression describing HETP, as presented by Golay, have numerous dependencies, and many of the variables that make up the equation have their own dependencies. The column radius is fixed and appears in two of the three terms. All the other variables are dependent on temperature. The average carrier gas velocity is dependent on the choice of carrier gas, the column dimensions (length and inside diameter), and temperature. The partition coefficient depends on the chemical nature of the analyte and stationary phase, and the retention factor depends on the partition coefficient, column phase ratio, and is further related to the average carrier gas velocity.

In our next instalment, we will examine these dependencies and the assumptions upon which our traditional view of HETP, based on the “Golay Equation”, are founded. We will also discuss their relevance in today’s capillary GC. The major changes between thinking about GC from the 1950s, when these ideas were first developed, and today include: the nearly universal use of temperature programming, smaller diameter capillary columns, thinner stationary phase films, and high vacuum column outlets, as seen in GC–mass spectrometry (MS). We will see that many of the traditional views that we were all taught (and still teach) about HETP, peak broadening, and peak widths in gas chromatography deserve some new thinking.

References

1. A.I.M. Keuelmans, Gas Chromatography (Reinhold, New York, USA, 1959), pp. 106–139.
2. H.M. McNair and E.J Bonelli, Basic Gas Chromatography (Varian, Palo Alto, USA, 1965).
3. M.J.E. Golay, in Gas Chromatography, D.H. Desty, Ed.(Butterworths, London, UK, 1958), pp. 36–55.
4. C.S.G. Phillips and F. Sjenitzer, in Gas Chromatography, D.H. Desty, Ed.(Butterworths, London, UK, 1958), pp. 65–66.
5. H.M. McNair, J.M. Miller, and N.H. Snow, Basic Gas Chromatography, 3rd. Edition, (John Wiley and Sons, New York, USA, 2019), pp. 15–36.
6. L.M. Blumberg, in Gas Chromatography, 2nd Edition, C.F. Poole, Ed. (Elsevier Amsterdam, The Netherlands, 2021), pp. 48–67
7. “GC Band Broadening”, ChromAcademy, LCGC Magazine’s Online Learning Platform. https://www.chromacademy.com/channels/gc-training-courses/principles/gc-band-broadening/ (accessed December 2021).

About The Author

Nicholas H. Snow is the Founding Endowed Professor in the Department of Chemistry and Biochemistry at Seton Hall University, and an Adjunct Professor of Medical Science. During his 30 years as a chromatographer, he has published more than 70 refereed articles and book chapters and has given more than 200 presentations and short courses. He is interested in the fundamentals and applications of separation science, especially gas chromatography, sampling, and sample preparation for chemical analysis. His research group is very active, with ongoing projects using GC, GC–MS, two-dimensional GC, and extraction methods including headspace, liquid–liquid extraction, and solid-phase microextraction. Direct correspondence to: amatheson@mjhlifesciences.com