How Fast Can A Gradient be Run?

For method development or routine analysis, the minimum gradient time may be a limit of throughput.

When you are developing a new liquid chromatography (LC) method, speed is often the primary interest — both speed in development and speed in terms of run time for the final method. With the expanding interest in Quality by Design (QbD) (1), we would like to know how different variables affect a separation, — both to ensure that a method is robust and to provide information for method adjustment when necessary. Gradient elution is often a first choice for method development experiments because the run times are defined and gradient data can be converted to isocratic conditions with the help of software programs such as DryLab (Molnar Institute, Berlin, Germany).

Because speed is of interest, it is good to know just how fast we can make gradient runs and still have "good" chromatography. After all, we don't want to just blast the sample through the column with no chance of separation. Fortunately, there is a well-developed theoretical basis for gradient elution (2) that allows us to make predictions of conditions that will satisfy our goals.

Selecting a Gradient Time

Before we look at gradients, let's consider isocratic separations, in which the organic solvent (%B) in a reversed-phase (RP) mobile phase is at constant concentration. For isocratic conditions, an ideal separation will have retention factors, k, for all analytes in which 2 < k < 10, but it is sometimes hard to fit all peaks within this range, so we settle for 1 < k < 20 for acceptable chromatographic behavior. For k < 1, there is a risk of interferences from unretained material from the sample matrix and retention is very sensitive to small changes in %B. For k > 20, peaks become broad, and thus harder to detect, and the run time gets inconveniently long.

With gradient separations, the k-value changes as the sample travels through the column. At the beginning of the separation, the %B is low and k is large; as the peak moves along the column, the %B rises and k drops. Thus, k in the isocratic context is a moving target during a gradient run. For this reason, we use k* to represent a single number in gradients; this can be thought of as an average k-value. For gradients, k* is approximately the same for all peaks in the chromatogram and can be estimated as:

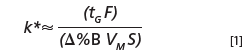
where t_G is the gradient time (in minutes), F is the flow-rate (mL/min), Δ%B is the gradient range (5–95% = 0.9), VM is the column volume (mL), and S is the slope of a plot of isocratic log k vs. %B. S can be estimated as:

where MW is the molecular weight. A value of S = 5 will be used in the present discussion, representing a typical value for compounds of MW < 1000 Da. Because S-values are approximately (but not exactly) the same for a sample mixture, k*-values (Equation 1) will also be approximately the same for all peaks in a run under the same gradient conditions. Like isocratic runs, it is desirable to have 2 < k* < 10 for gradients. If a pair of gradient runs is desired for use with predictive calculations, such as with DryLab, k*-values differing by 2–3-fold are recommended (for example, runs with k* = 3 and 6). For fast runs with "good" chromatography, the topic of this discussion, we'll use k* = 2.

We can rearrange Equation 1 to estimate the gradient time required for various chromatographic conditions:

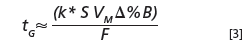
An Example

Let's look at an example using Equation 3 for a separation using a 150 × 4.6 mm, 5-µm particle diameter, d_p, column run at 2 mL/min (F = 2) and a gradient range of 5–100%B (Δ%B = 0.95). An estimate of the column volume is:

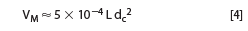
where L is the column length and d_c is the column diameter, 150 mm and 4.6 mm here. (This assumes a total column porosity of ≈65%.) So VM ≈ 1.6 mL for the present example. For k* = 2, we can calculate:

t_G ≈ (2 × 5 × 1.6 × 0.95)/2 ≈ 7.5 min

You can find this summarized in the first line of Table 1. (Note that in this calculation and all those in Table 1, I've rounded the numbers, so if you repeat these calculations, your results may vary slightly.)

It might also be interesting to estimate the pressure, P, under these conditions. We can do this (in psi) with

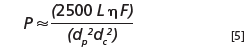
[Equation 2.13a in (3)] where η is the mobile phase viscosity in cP. For gradients, we need to use the highest viscosity during the run. For acetonitrile as the B-solvent and 30 °C, η = 0.9 [Table 1.5 of (3)]. This gives a maximum pressure of ≈ 1275 psi; in my experience, this calculation is a bit low for real columns, so I've multiplied values from Equation 5 by 1.5 for Table 1 to give a better feel of the pressure that is likely to be observed.

Some Other Column Configurations

I've made similar calculations as described above and summarized these in Table 1 for several other popular column configurations. The columns all have approximately the same number of theoretical plates, N ≈ 10 000 (±10%) under realistic conditions, so they should give the same separations in all cases; the exception is the 50 × 2.1 mm, 3-µm column with N ≈ 5000. The flowrates were chosen to give pressures of ≈ 2000–3000 psi (135–205 bar) for conventional LC equipment and 10 000–15 000 psi (680–1020 bar) for ultrahigh-pressure high-performance LC (UHPLC), which represent "typical" method conditions.

You can see in Table 1 that for conventional methods using 100–150 mm columns with 3- or 5-µm particles, gradient times of 7–8 min are used for k* = 2. For a second calibration run for retention modeling software, use a run three-fold longer (20–25 min). For the 50 × 2.1 mm, 3 µm column popular for LC–MS, a gradient of ≈2 min is required.

Under UHPLC conditions with a 2-µm column (last two rows in Table 1), run times of just under 2 min are required. For comparison, I've included a 2.5-µm shell-type column (third line from the bottom of Table 1); these columns have pressures reflected by the particle size, but performance more like a 2-µm particle. In the configuration chosen, gradient times are about the same as UHPLC columns, but with a somewhat lower pressure.

So far so good. It looks like we can get "good" chromatography with runs of 7–8 min on conventional LC equipment and in 2 min for UHPLC conditions. These are fast enough to give us the ability to screen many possible variables in a reasonable amount of time. However, there are a couple additional factors that we'll consider next.

Gradient Delay

One factor to take into account with fast gradients is the gradient delay. This is determined by the dwell volume, V_D, of the system, which comprises the mixer, connecting tubing, sample loop and, for lowpressure mixing systems, the pump head volume. The dwell volume should be measured for each LC instrument; directions for this were given in a previous LC Troubleshooting instalment (4). Typical dwell volumes for conventional highpressure mixing LC systems are in the 1.5–3.0 mL range; their low-pressure mixing counterparts generally are slightly larger, with 2.5–4.0 mL dwell volumes. Highpressure mixing systems that have been tuned up for LC–MS work and UHPLC systems typically have dwell volumes of 0.3–0.5 mL, whereas low-pressure mixing UHPLCs have 1.0–1.5 mL.

The practical impact of the dwell volume on the run time is that it extends the run because the dwell time, t_D — the time it takes for the gradient to reach the column after it is started — is added to the gradient time. This is shown in the third column from the right of Table 1. I've assumed a 2.0-mL dwell volume for conventional LC systems (first three rows) and 0.4 mL for the LC–MS and UHPLC configurations. Note that for the 4.6-mm columns and 1.5–2 mL/min flow-rates (first two lines of Table 1), the added time is 15–20%, but if a 100 × 2.1 mm column is used, the total run time is nearly doubled by the gradient delay. With the 2.1-mm i.d. columns used for LC–MS or UHPLC, the delay can be substantial, 25–40% additional time for the run. For some instruments, it may be possible to delay the injection until the gradient reaches the column, but the dwell time must be paid for sometime — in such cases, it is moved from within the run to between consecutive runs. Additionally, the dwell volume must be thoroughly flushed out when changing the mobile phase from one condition to another during method development.

It is clear that it is desirable to minimize the dwell volume to maximize throughput with gradients. In some cases it may be possible to reduce the dwell volume by changing system plumbing, but there is a minimum dwell volume that is characteristic of each LC system.

Gradient Distortion

Another potential problem related to the dwell volume is gradient distortion. This is illustrated in Figure 1. In each case, the gradient programme is shown with a dashed line and the solid line shows the actual gradient generated by the LC system. In the top case, the gradient is ramped at 3%/min and in the lower run, 14%/min. You can see that in one case, the actual gradient closely follows the intended one, whereas in the steep gradient there is considerable distortion in the actual gradient. Clearly the lower case is undesirable. Such gradients are very instrument-dependent and will be hard to transfer. Based on these data (5), it was concluded that as long as the gradient volume is at least twice as large as the dwell volume, gradient distortion should be minimal. The upper plot has a gradient volume twice the dwell volume, whereas the ratio for the lower one is only 0.3.

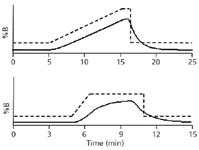
Figure 1: Gradient distortion comparing 3%/min gradient (top) and 14%/min (bottom). Dashed lines show gradient programme; solid lines show actual gradient. Data from (5).

The next-to-last column in Table 1 shows the gradient volume, V_G (= t_G × F), for each gradient condition. The maximum recommended dwell volume (half the gradient volume) is shown in the far right column of Table 1. We can conclude that gradient distortion is not likely to be a big problem with conventional LC equipment used with 4.6-mm i.d. columns or with LC–MS or UHPLC equipment used with 2.1-mm i.d. columns. In either case, however, when smaller diameter columns are used, gradient distortion is a factor that should not be ignored. In any case, it is wise to check to be sure that your LC system will generate a distortion-free gradient under the fastest desired gradient conditions. This can be done in the same manner as the dwell volume measurement discussed above (4), but using the desired gradient conditions.

Table 1: Characteristics of various gradient configurations.

Conclusions

Fast gradients can be generated reliably with conventional, LC–MS and UHPLC-type LC equipment if certain precautions are taken. Fast gradients allow for rapid screening and optimization of several variables during method development and the creation of short run times for routine analysis. It is important to know the dwell volume of each gradient system so that gradient delay can be taken into account. A check should also be made that gradient distortion is not observed, or the transfer of such gradients may be problematic. It should be obvious that longer, larger-volume gradients will be less subject to distortion, so only the shortest gradients anticipated need to be checked for potential distortion problems.

"LC Troubleshooting" editor, John Dolan, is vice president of LC Resources, Walnut Creek, California, USA. he is also a member of LCGC Europe's editorial advisory board. Direct correspondence about this column should go to "LC Troubleshooting", LCGC Europe, 4A Bridgegate Pavillion, Chester Business Park, Wrexham Road, Chester CH4 9QH, UK, or e-mail the editor, Alasdair Matheson, at amatheson@advanstar.com

References

1. International Committee on Harmonization, ICH Q8 Pharmaceutical Development (May 2006).

2. L.R. Snyder and J.W. Dolan, High-Performance Gradient Elution (Wiley Interscience, New York, NY, 2007).

3. L.R. Snyder, J.J. Kirkland and J.W. Dolan, Introduction to Modern Liquid Chromatography (Wiley, New York, NY, 3rd Edition, 2010).

4. J.J. Gilroy and J.W. Dolan, LCGC, 22(10), 982–988 (2004).

5. G. Hendricks, J.P. Franke and D.R.A. Uges, J. Chromatogr. A, 1089, 193 (2005).