Estimating Resolution for Marginally Separated Peaks

How can resolution be determined when peak width cannot be measured?

I have had several reader inquiries lately regarding how to estimate resolution between two peaks in a liquid chromatography (LC) separation when the traditional calculation doesn't work. An example of this is shown for peak pairs A, B, and C in the chromatogram of Figure 1. In each case, the valley between the peaks does not dip below 50% of the height of the smaller peak, making it impossible to measure the peak width at the baseline or half-height. In this month's "LC Troubleshooting" installment, I would like to share a simple technique to estimate resolution that has been in use for many years (for example, see reference 1), but may not be well known because of our dependence on automatic data processing systems today.

Figure 1: An example of poorly resolved peak pairs A, B, and C.

Traditional Measurements

Most of us use the method of equation 1 or 2 to calculate the resolution, R_s, of a pair of peaks with retention times t₁ and t₂:

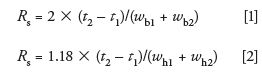
where w_b1 and w_b2 are the baseline peak widths between tangents drawn to the sides of the peaks, and w_h1 and w_h2 are the corresponding peak widths measured at half the peak height. That is, the resolution is the difference in retention times divided by the average baseline peak width (thus the factor of 2 in equation 1). The peak width at the baseline for a Gaussian peak is 4σ (4 standard deviations), whereas at the half-height, it is 2.354σ, so the factor in equation 2 is (2 × 2.354/4) = 1.18. Because the half-height peak width is easier to measure (no tangent drawing involved), most data systems use the half-height method (equation 2) to calculate resolution.

The technique of equations 1 and 2 works well when the peaks are well separated, as with Figure 2a, where R_s = 1.3. When the resolution drops much below this, it will be difficult to measure the baseline peak width, but it may be possible to measure the half-height width. However, as the resolution drops, the valley between the two peaks rises, and at some point it becomes no longer possible to measure the half-width, either (for example, Figure 2b). Unfortunately, as the amount of peak overlap increases, measurement of resolution becomes more important. This is because it is more difficult to accurately measure peak areas when resolution drops below ~1.2, so system suitability tests often have minimum resolution requirements for partially separated peaks.

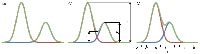
Figure 2: Simulated chromatograms for peak-height ratios of 2:1. (a) Rs = 1.3; (b) Rs = 0.9, h1, h2, and hv are heights of the first peak, second peak, and valley, respectively; (c) Rs = 0.75.

Modeling Peak Overlap

One way to estimate resolution for overlapping peaks is to measure the relative height of the valley between the peaks. The ideal chromatographic peak is Gaussian in shape, so we can generate Gaussian peaks (for example, in Excel) and sum their peak heights across a two-peak chromatogram. By changing the amount of resolution (overlap) and the peak heights, we can generate a simple tool to estimate resolution based on the relative height of the valley.

The Gaussian function is as follows:

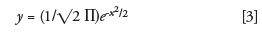
where y is the peak height at point x and x is the retention time measured from the band center (t = 0) in units of the standard deviation of the peak (σ). All the peaks I generated for Figures 2 and 3 range from times of -4σ to +9σ relative to the center of the first peak. Recall that the width of a peak between tangents drawn to baseline is 4σ. The chromatogram for the first peak of each pair is shown in red, the second in blue, and the sum of the two in green. In all cases, the second peak is of constant height, and the height of the first peak is varied (for example, in Figure 2, peak 1 is always twice the height of peak 2). Note that all the chromatograms of Figures 2–4 are computer-generated simulations; real peaks are likely to tail somewhat.

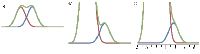
Figure 3: Simulated chromatograms for various peak-height ratios. (a) peak-height ratio = 1:1, Rs = 0.6; (b) ratio = 10:1, Rs = 0.9; (c) ratio = 100:1, Rs = 0.9.

Now, I have the ability to generate chromatograms as in Figures 2 and 3 for any desired resolution and any relative peak height. I have used such data to construct Table I. The resolution (left-hand column) is known from the inputs. From the data in the spreadsheet (not shown) or the chromatograms, I can determine the peak height (which may be increased by overlap from the other peak) as h₁ and h₂ for the two peaks and the peak-height ratio (always h₁:h₂ in Figures 2 and 3 because the second peak is smaller). The height of the valley (green line) is h_v/h₂ (always the smaller of the two peaks). Each column of data is for one peak-height ratio, with the %-height of the valley shown below for each value of resolution. (I have done some rounding of numbers for presentation simplicity, so if you try to repeat my calculations, your results may vary slightly.) It should be obvious that if peak 2 is larger than peak 1, the data of Table I still apply — just remember to use the smaller of the two peaks for the valley-height ratio.

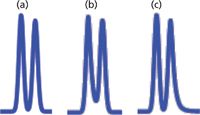
Figure 4: Simulated chromatograms showing effect of peak tailing factors (TF) (for peaks 1 and 2, respectively), on resolution for peak-height ratios of 1:1. (a) TF1 = TF2 = 1.0; (b) TF1 = 1.5, TF2 = 1.0; (c) TF1 = 1.0, TF2 = 1.5.

Application to Example Data

Next, let's see when it is appropriate to use Table I to estimate resolution and when it is not very useful. first, consider the chromatogram of Figure 2a. In this case, R_s = 1.3 and the first peak is twice the height of the second (h₁:h₂ = 2:1). In this example, either the baseline or half-height peak widths can be measured easily, so there is no need to use Table I for help. A calculation using either equation 1 or equation 2 will give better results when one of them can be applied. You can see from Table I (R_s = 1.3, ratio = 2:1) that the height of the valley is 10%. My suggestion is not to use Table I if the valley is less than ~10%, so I have not even shown valley heights < 10%.

For the chromatogram of Figure 2b (R_s = 0.9, ratio = 2:1), the valley height is 54%. This means that it is not possible to measure the peak width, even at the half-height, so the method of Table I will be quite useful.

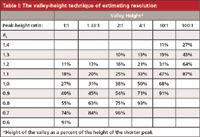
Table I: The valley-height technique of estimating resolution

Table I works quite nicely when the peak-height ratios and the valley heights correspond to values in the table. When this is not the case, some interpolation will be necessary. In the example of Figure 2c, the peak-height ratio is 2:1 and valley is 86% of the height of the second peak. In the 2:1 ratio column of Table I, 86% falls roughly midway between a valley of 75% (R_s = 0.8) and 96% (R_s = 0.7), so we can assign R_s = 0.75 to this chromatogram (which is the resolution I selected to generate the data). Because of the rather coarse nature of the data intervals in Table I and other uncertainties, I suggest that you don't refine your estimates of resolution by more than 0.05 units using this technique.

Some Limitations to the Valley Ratio Technique

I mentioned above that whenever you can measure the peak width at baseline or half-height, you are better off using the traditional resolution calculation of equation 1 or 2. Let's look at some other examples where the valley-height technique is limited. In the chromatogram of Figure 3a, you can see that there is barely any valley between the peaks for the case of equal height peaks and R_s = 0.6 — the valley height is 91%. You can also see that the peak heights (green) of both peaks are greater than the individual peaks (red and blue) because of severe overlap. I don't think it is worthwhile trying to estimate the resolution with the valley-height technique if the valley height is more than ~90%. I have left a few values with valleys > 90% in Table I to help with interpolation with smaller valley ratios.

As the peak height difference between the two peaks gets larger, the valley-height technique still works, but a larger difference in retention times is required to obtain a reasonable valley. For example, in Figure 3b with a peak height ratio of 10:1, R_s = 0.9 is required to get the same 91% valley as with a 1:1 peak height ratio where resolution of only 0.6 was required (Figure 3a). When a peak height ratio of 100:1 is encountered (Figure 3c), R_s = 0.9 produces only a small bump on the tail of the first peak. At some point when the peak size ratio becomes too large, even with symmetric peaks, it will not be possible to observe two peaks, whereas with the same retention times and equal sized peaks, the separation would be obvious. Compare the chromatograms of Figures 2b, 3b, and 3c to see this trend for peaks with R_s = 0.9.

Peak tailing of the first peak of a peak pair will always reduce the resolution, whether the calculations of equations 1 or 2, or the valley-height technique of Table I is used. This is illustrated with Figure 4, where Figure 4a has R_s = 1.5 and both peaks are symmetric, so the tailing factor (TF) is 1.0. In Figure 4b, the peaks have the same retention times, but the first peak has TF = 1.5. My measurements show a valley height of 19%, so for a 1:1 peak height ratio this equates to R_s ≈ 1.1. On the other hand, tailing only for the second peak, as in Figure 4c, will not affect the resolution. It is only the case where the peak tails into the valley that resolution is reduced.

A Real Example

All of the chromatograms in Figures 2–4 are simulated; so what happens when we try to apply the valley-height technique to a real example? Let's go back to the chromatogram of Figure 1, where the reader was unable to determine resolution between peak pairs A, B, and C using direct calculations. I expanded the chromatogram and measured the peak and valley heights for each pair. For peak pair A, the peak height ratio is 1.36:1 and the valley height is 64%. The height ratio is closest to the 1.33:1 data set in Table I, so I would estimate R_s ≈ 0.8. For pair B, the height ratio is 2.17:1 and the valley is 50%. Using the 2:1 column in Table I, R_s ≈ 0.9. For pair C, the peak height ratio cannot be determined, but my guess is that it is at least 100:1 and the valley is 69% of the smaller peak, so this gives R_s ≈ 1.2. You could interpolate more carefully between the data points in Table I, but as I mentioned above, I would not try to get closer than ~0.05 resolution units using Table I. I don't know the nature of the sample in Figure 1, but it is typical of what might be observed for an impurities analysis of a pharmaceutical product. In such cases it may not be essential, or even possible, to separate all peaks to baseline, but it would be nice to be able to put a number on the resolution of the various peak pairs. Using the valley-height technique, we can do just that, when the traditional approach of equations 1 and 2 was not possible.

Conclusions

When chromatographic peaks are well separated, the traditional technique of calculating resolution based on retention time differences and average peak widths, as in equations 1 and 2, is the preferred way to determine resolution. This would generally apply over the range of peak height ratios of approximately 1:1 to 1:10 and resolution of at least 1.3. When resolution drops below ~1.3, it becomes more difficult to determine peak widths, so an estimate of resolution using the valley-height technique is more convenient. When the valley height exceeds ~40% of the height of the smaller peak, even the width at half height cannot be determined with confidence, so the valley-height method is the best choice. In the real chromatogram of Figure 1, we were able to estimate the resolution of three peak pairs using the valley-height technique when it was not possible to make the corresponding calculations with equation 1 or 2.

Peak tailing for the first peak of a peak pair will always reduce resolution. In such cases, the valley-height technique may give more realistic values of resolution, as was illustrated with the chromatograms of Figure 4.

From a system suitability standpoint, the valley-height technique can be useful to assess separation quality when a marginal separation is the best that can be obtained, as in Figure 1 for peak pairs A, B, and C. For the example of Figure 2b, there is very little peak overlap at the peak centers, so peak-height measurements may be more appropriate for quantification; alternatively, peak-area measurements, using a perpendicular drop at the valley to separate the peak areas, should also give acceptable results for this example. You could specify in the system suitability requirements that R_s > 0.9 should be obtained. For routine work, however, it may be more convenient just to set a limit on the valley height that corresponded to R_s = 0.9. For example, you could require that the valley be no more than 55% of the height of the second peak and have the same result without the added step of looking up the resolution in Table I.

There is an interesting paradox here: As chromatographers, we usually are more concerned with peak separation when resolution drops below R_s ≈ 1.5, at which point we must rely more on estimates than more exact calculations. Conversely, when R_s > 1.5, calculations become easier, but once baseline resolution is obtained, accurate measurements of resolution are of less interest. So when accuracy is important, we can't get it, and when it is not as important, it is easy to obtain.

References

(1) L.R. Snyder and J.J. Kirkland, An Introduction to Modern Liquid Chromatography, 2nd edition (Wiley-Interscience, New York, 1979), Chapter 2.

John W. Dolan "LC Troubleshooting" Editor John Dolan has been writing "LC Troubleshooting" for LCGC for more than 30 years. One of the industry's most respected professionals, John is currently the Vice President of and a principal instructor for LC Resources in Walnut Creek, California. He is also a member of LCGC's editorial advisory board. Direct correspondence about this column via e-mail to John.Dolan@LCResources.com

John W. Dolan