Ultrahigh-Efficiency Protein Separations with Submicrometer Silica Using Slip Flow

Professor Mary Wirth and graduate students Bingchuan Wei and Benjamin Rogers from Purdue University demonstrate a quantum leap in protein column efficiency. Using colloidal silica particles of submicrometer diameters (470 nm), they obtained plate heights that were as much as 15-fold lower than the theoretical limit for Hagen-Poiseuille flow. The smallest plate height was 15 nm, 500-fold smaller than previous reports for pressure-driven protein chromatography. This remarkable achievement was attributed to "slip flow," which is explained in this installment. The authors also present a practical example using this new concept.

The plate heights of commercial liquid chromatography (LC) columns have changed little over the last 20 years, following the trend of H_min ≈ 2d_p, where d_p is the particle diameter (1). Simulations have shown that H_min ≈ d_p is the limit for nonporous particles (2). With particle sizes dropping by only a factor of about two during the last 20 years, as such plate heights have improved by the same factor. The reason is not a lack of diligence or creativity; instead, it is because of the fundamental limits of mass transport, with the ultimate limit being the velocity spread of the mobile phase across the spaces between particles. We recently used a nanofluidic phenomenon called slip flow (3–8), which greatly reduces this velocity spread, thereby reducing the plate height dramatically. We demonstrated a plate height as low as 0.03 d_p, which was 15 nm (9). This opens a new horizon for efficiency in ultrahigh-pressure liquid chromatography (UHPLC).

The concept of slip flow is illustrated in Figure 1. Imagine that the walls are the surfaces of two adjacent particles. The familiar Hagen-Poiseuille flow is depicted with its parabolic velocity profile, in which the velocity goes to zero at the walls. This is the realm of chromatography today. The figure also depicts slip flow, in which the velocity does not go to zero at the walls, thereby giving rise to a narrower distribution of velocities in the column. Slip flow occurs when the molecular interactions between fluid and the surface are weak, allowing the fluid at the interface to have a nonzero velocity. The existence of slip flow is common knowledge in the field of fluid dynamics, where it is typically a small correction factor when there are weak fluid–surface interactions, but it becomes a big factor when the channel reaches the scale of tens of nanometers (3–8). Consequently, slip flow always occurs in reversed-phase LC, but it is not distinguishable from Hagen-Poiseuille flow because the dimensions of chromatographic materials are relatively large. The nanoscale channel dimensions are achieved by using submicrometer particles.

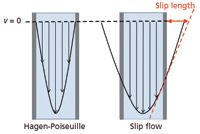
Figure 1: Illustration of how slip flow gives a narrower velocity distribution compared to Hagen Poiseuille flow. The velocity is far from zero at the wall for slip flow.

Experimentally, the parabolic flow profile cannot be directly viewed on the nanoscale, but it can be inferred because one can measure a companion to the narrower flow profile: a faster flow rate. Because slip flow gives a nonzero velocity at the wall, the volume flow rate, Q, is enhanced relative to that for Hagen-Poiseuille flow, Q_HP. In equation 1, for a capillary of radius r, the term L_s is the slip length, which is defined as the ratio of the velocity to shear rate, each at the wall.

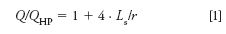
For water in contact with various hydrocarbon surfaces, the slip lengths are all under 100 nm (3,5,7,8); therefore, channel radii less than 100 nm will give noticeable slip flow. Having r in the denominator explains that the smaller the channel, the greater the enhancement from slip flow.

Figure 2 shows experimental results for volume flow rates we have reported for a capillary packed with a 2-cm plug of 470-nm particles (9). These particles formed colloidal crystals inside the capillary (10,11). They were then chemically modified to have a mixed self-assembled monolayer of butyl groups and methyl groups (12). For varying mobile-phase composition, the flow rates are normalized by mobile-phase viscosity after accounting for its small pressure dependence over this range (13). These lines would all coincide for Hagen-Poiseuille flow; instead, they are quite different from one another. Furthermore, the slopes increase in order of immiscibility with alkanes, which is consistent with the idea that weaker interactions between the fluid and the surface give increased slip flow. The data show that the slope for toluene coincides with the line calculated for Hagen-Poiseuille flow (dashed line), whereas the slope for water is five-fold higher. The reason is that slip flow is negligible for toluene because it interacts well with the hydrocarbon surface, whereas slip flow dominates for water because of its very weak interactions with hydrocarbons. The toluene data confirm that the material does not have parasitic flow from cracks, wall gaps, or other defects that would enhance flow in an undesirable way.

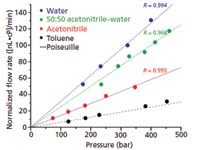
Figure 2: Plot of flow rate vs. pressure, normalized for viscosity, illustrating that slip flow increases with solvent immiscibility in hydrocarbon. The dashed line is calculated for Hagen-Poiseuille flow; other lines are linear fits to the flow data. L = 2 cm. Adapted from reference 9 with permission.

In Figure 3, chromatograms for a commercial monoclonal antibody are compared for a silica colloidal crystal (Figure 3a) like the one used for Figure 2, and a 5-cm-long commercial wide-pore UHPLC column (Figure 3b) (9). The silica colloidal crystal can separate the antibody from its aggregates in 1/10 the time and at room temperature. The peak sharpness enables the use of low retention conditions to resolve these analytes at room temperature. Because elevated temperature promotes antibody degradation and aggregation, slip flow allows operation under conditions that minimally alter the protein. Fluorescence imaging was used to sample the sharp peak of Figure 3a with high point density, revealing that the plate height is 70 nm for the monoclonal antibody peak (9). An example of fluorescence imaging is illustrated in Figure 3c for the lowest plate height we have observed, which is bovine serum albumin (BSA). Over-labeling yields two peaks because of one versus two dye labels on the protein. Figure 3c shows an image of the two BSA peaks as they approach the exit of a 2-cm-long capillary. From Gaussian fitting of the peak profiles, shown in Figure 3d, the standard deviation of the earlier eluted peak is 16 μm, which corresponds to a plate height of 15 nm for the separation distance of 17.5 mm, thus approaching the diffusion limit (9). This plate height is a factor of 500 times smaller than the plate height of the protein chymotrypsin, which was measured for 1.5-μm nonporous silica particles in reversed-phase UHPLC (14). The number of plates for this separation distance of 17.5 mm is 1.2 × 10⁶ plates. The only other example of a plate number exceeding 1 million was a separation of aromatic compounds using silica monolith of L = 8.5 m and P = 340 bar, where the separation time was 1480 min (24 h) (15). This is more than 700 times longer than the 2-min separation time for the BSA sample for essentially the same pump pressure. This illustrates that the flattening of the Poiseuille flow profile under slip conditions has a very large effect on the column length needed to obtain a high separation efficiency (9).

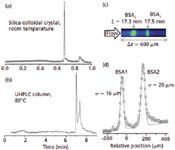
Figure 3: Chromatograms for monoclonal antibody separation obtained using (a) slip flow (isocratic 45:55 acetonitrile–water, 0.1% trifluoroacetic acid; P = 460 bar; L = 1.45 cm) and (b) UPHLC (Waters Acquity C4, 1.7 μm; gradient: 10–70% acetonitrile over 10 min; flow rate: 1 mL/min). (c) Imaged peaks for BSA, with second peak caused by double labeling (isocratic 25:75 acetonitrile–water, 0.1% trifluoroacetic acid; P = 330 bar; L = 1.75 cm). (d) Gaussian fit of image data. Adapted from reference 9 with permission.

Something remarkable happens when separations approach the diffusion limit: Length no longer contributes to resolution. Of course, for extremely short lengths other factors will eventually enter in, such as injected width. But for reasonable lengths, the equation for plate height, H, in the diffusion limit simplifies to just the B term in the van Deemter equation, as given in equation 2:

In this case, only the diffusion coefficient, D, and the obstruction of diffusion by the limited column porosity, ε, contribute to H for a given mobile-phase velocity, ν. The velocity is determined by the pressure, P, the mobile-phase viscosity, η, and other factors described by the Kozeny-Carman relation of equation 3, for which we added the flow enhancement factor, E.

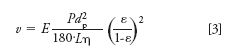
Because the number of plates, N, is L/H, it is straightforward to show that this maximum number of plates under the diffusion limit, N_max, is dictated by pressure and is independent of length.

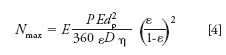
This situation is analogous to capillary electrophoresis, where the plate number is proportional to the applied voltage, but not length (16). Ultimately, injected width and heating impose an upper limit to N in capillary electrophoresis (16). For UHPLC, these limitations will not be reached as easily: Injected width is minimized in chromatography by stacking at the head of the column, and heating is minimized because pumping brings in fresh fluid, and solids are better heat conductors than liquids. Radial homogeneity of the packing has been a problem in chromatography (17), but this is not the case for colloidal crystals (10,11). A method for rapid homogeneous packing with negligible eddy diffusion is the subject of a pending patent (18). The independence of plate height on separation length explains why it is possible to achieve such high plate numbers for such short separation distances of L < 2 cm.

Using equation 4 to calculate the maximum possible plate number under the diffusion limit, for a typical medium sized protein, D = 1.0 × 10^-6 cm²/s, and for the separation parameters of d_p = 470 nm, ε = 0.28, a flow enhancement of five, and P_max = 800 bar, the result is N_max = 1.5 × 10⁶. It is important to note that when 1.5 × 10⁶ plates are observed in 1 cm, for example, one cannot claim this corresponds to 1.5 × 10⁸ plates/m. The calculation also illustrates why the plate heights are so low for proteins: Their diffusion coefficients are an order of magnitude or more lower than those of small molecules.

Future Directions in Slip Flow Separations

Can slip flow be used to advance separations of peptides and small molecules? Reversed-phase LC is widely used for both; therefore, it is worth considering. Smaller particles would be needed for sufficient retention factors. Equation 4 shows that N decreases rapidly with d_p². Fortunately, this is offset by an important aspect of slip flow: flow enhancement increases for smaller particles, as per equation 1. This reduces the steep penalty in back pressure as particle diameter decreases. As particle diameters become less than 0.2 μm, the calculated ratio of surface area to mobile-phase volume reaches that of porous silica, offering the capacity that has always been lacking for nonporous particles. With the faster analyte diffusion coefficients, equation 4 shows that N_max would be limited to ~100,000 for an 800-bar pump. These media could thus be quite advantageous in efficiency and even more so for speed. We are beginning to explore this avenue.

Acknowledgment

This work was supported by National Institutes of Health (NIH) under grant R01GM101464.

Conflict of Interest Statement

The senior author has a financial interest in a company that has licensed this technology.

References

(1) R.W. Brice, X. Zhang, and L.A. Colón, J. Sep. Sci. 32, 2723–2731 (2009).

(2) M.R. Schure, R.S. Maier, D.M. Kroll, and H.T. Davis, J. Chromatogr., A 1031, 79–86 (2004).

(3) C.-H. Choi, K.J.A. Westin, and K.S. Breuer, ASME Conference Proceedings 2002, 557–564 (2002).

(4) M. Majumder, N. Chopra, R. Andrews, and B.J. Hinds, Nature 438, 44 (2005).

(5) X.C. Qin, Q.Z. Yuan, Y.P. Zhao, S.B. Xie, and Z.F. Liu, Nano Lett. 11, 2173–2177 (2011).

(6) S. Sinha, M.P. Rossi, D. Mattia, Y. Gogotsi, and H.H. Bau, Phys. Fluids 19, 013603 (2007).

(7) J.A. Thomas and A.J.H. McGaughey, Nano Lett. 8, 2788–2793 (2008).

(8) M. Whitby, L. Cagnon, M. Thanou, and N. Quirke, Nano Lett. 8, 2632–2637 (2008).

(9) B. Wei, B.J. Rogers, and M.J. Wirth, J. Am. Chem. Soc. 134, 10780–10782 (2012).

(10) D.S. Malkin, B. Wei, A.J. Fogiel, S.L. Staats, and M.J. Wirth, Anal. Chem. 82,2175–2177 (2010).

(11) B. Wei, D.S. Malkin, and M.J. Wirth, Anal. Chem. 82, 10216–10221 (2010).

(12) R.W.P. Fairbank, Y. Xiang, and M.J. Wirth, Anal. Chem. 67, 3879–3885 (1995).

(13) J.W. Thompson, T.J. Kaiser, and J.W. Jorgenson, J. Chromatogr., A 1134, 201–209 (2006).

(14) N.J. Wu, Y.S. Liu, and M.L. Lee, J. Chromatogr., A 1131, 142–150 (2006).

(15) K. Miyamoto, T. Hara, H. Kobayashi, H. Morisaka, D. Tokuda, K. Horie, K. Koduki, S. Makino, O. Nunez, C. Yang, T. Kawabe, T. Ikegami, H. Takubo, Y. Ishihama, and N. Tanaka, Anal. Chem. 80, 8741–8750 (2008).

(16) A.W. Moore and J.W. Jorgenson, Anal. Chem. 65, 3550–3560 (1993).

(17) K.D. Patel, A.D. Jerkovich, J.C. Link, and J.W. Jorgenson, Anal. Chem. 76, 5777–5786 (2004).

(18) US patent application 6⅓21,098. Inven-tors: M.J. Wirth, C. Yerneni, S. Ranas-inghe, R.A. Birdsall.

This month's guest authors:

Benjamin J. Rogers is a fourth year doctoral graduate student in the analytical chemistry program at Purdue University, where he works in the Wirth group. He earned his B.S. in chemistry at Queen's University in Ontario, Canada.

Benjamin J. Rogers

Dr. Bingchuan Wei received his PhD in chemistry from Purdue University in 2011, and he has continued in the Wirth group as a post-doctoral fellow. He earned his B.S. in chemistry at Peking University in Beijing, PRC.

Dr. Bingchuan Wei

Dr. Mary Wirth is the W. Brooks Fortune Distinguished Professor in the Department of Chemistry at Purdue University, where she has established a research program combining chemical separations and material science.

Dr. Mary Wirth

The editor of Column Watch:

Ronald E. Majors "Column Watch" Editor Ronald E. Majors is a Senior Scientist in the Columns and Supplies Division at Agilent Technologies (Wilmington, Delaware), and is a member of LCGC's editorial advisory board. Direct correspondence about this column to lcgcedit@lcgcmag.com.

Ronald E. Majors