Temperature Programmed GC: Why Are All Those Peaks So Sharp?

Temperature programming is used for most separations in capillary gas chromatography (GC) today. Despite this, many of the principles by which we understand temperature-programmed capillary column separations are based on ideas developed using packed columns and isothermal conditions. This instalment of “GC Connections” dives into temperature programming. First, the differences in peak widths and retention times between temperature programmed and isothermal chromatograms are examined. Why are all the peaks so sharp in temperature programmed GC, yet they get broader (and shorter) in isothermal GC? Next, we explore some early ideas about temperature programming and peak broadening that explain why the peaks are so sharp in temperature-programmed GC, and why the peak spacing is different from isothermal GC. Finally, we examine an important consequence of our ability to program temperature: the need for temperature programming in splitless and other injections that use “solvent effects” and other peak focusing mechanisms. These points are illustrated using several historical figures and chromatograms from the early days of GC.

Nearly every student of gas chromatography (GC) has seen chromatograms like the ones shown in Figure 1. These chromatograms were originally published in 1959, in one of the first papers describing an apparatus for temperature programming (1). Although developed on a handmade packed column with firebrick as the stationary phase, this work shows the same comparison and contrast between isothermal and temperature-programmed GC seen today. Starting from the bottom, Figure 1(c) shows an isothermal separation of normal alkanes. Notice that as the retention times get longer, the peaks get broader, and the last peak appears to exhibit fronting. Also notice that the retention time difference between each peak appears to nearly double with each successive alkane. The difference between C9 and C10 (the last two peaks) is about twice the difference between C8 and C9. Notice also that nearly 30 min is required to separate the six alkanes.

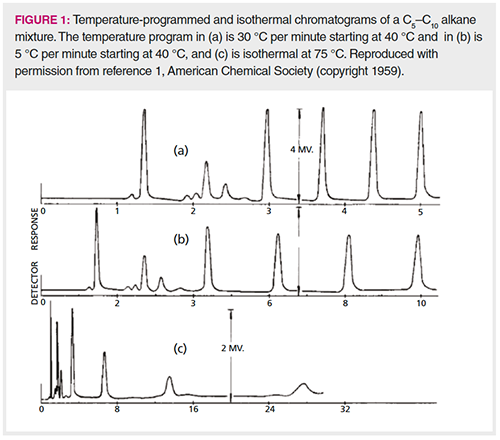
This chromatogram illustrates the main limitations of isothermal GC. First, the range of analytes that can be separated in a reasonable time is relatively small. Second, as the retention times get longer, the peaks get significantly broader (band broadening), and, as a result, they get shorter and harder to detect. If the peak area is constant, as a peak becomes broader, it must become shorter, limiting sensitivity. Third, the fronting of the later peaks is caused by the column temperature being too low for effective adsorption on the surface of the stationary phase. The liquid analyte condenses on the surface, causing some to be evaporated into the mobile phase more quickly and to therefore elute too soon. This is a form of column overload.

Moving up, Figure 1(b) shows a temperature-programmed separation of the same mixture of n-alkanes with a temperature programming rate of 5 °C per min and Figure 1(a) shows the same separation with a rate of 30 °C per min. Note the significant differences from the isothermal separation. First, the run time is reduced from 30 min to 10 and 5 min, respectively. Second, the peaks are spaced evenly. The retention time difference between each successive alkane is about the same. Finally, all of the peaks are sharper (remember this was a handmade packed column); they appear to have about the same peak width and as a result all have about the same peak height, while the isothermal peaks get broader and shorter.

Figure 1 raises two critical questions:

• Why are the retention times evenly spaced in the temperature programmed separation, while the spacing doubles from peak to peak in the isothermal run?

• Why are all the peaks sharp in the temperature-programmed separation, while the later peaks get significantly broader in the isothermal separation?

We will address these questions by drawing some simple pictures of the chromatographic process, followed by descriptions based on theories for both isothermal and temperature-programmed GC that were developed in the first 10 years of GC.

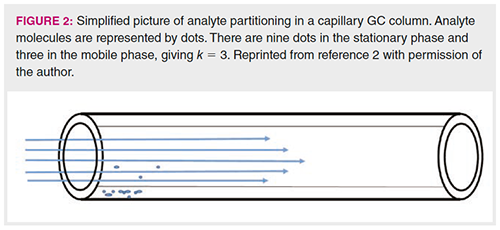
For an analyte to move along a GC column, it must have a vapour pressure of at least a few torr at the operating temperature. Remember that this vapour pressure is affected by both the normal vapour pressure and any change resulting from interactions with the stationary phase. Figure 2 shows a simplified picture of the inside of a capillary column, with the analyte molecules represented as dots (2). This compound has nine dots in the stationary phase and three in the mobile phase, giving a retention factor (k) of 3. A higher value of k indicates that more of the dots, a larger mass of the analyte, will be in the stationary phase, causing the analyte to be retained longer. The carrier gas forces the three dots in the mobile phase to move along the column. When they encounter fresh stationary phase, their attraction to the stationary phase and low (but finite) vapour pressure cause them to condense onto and dissolve in this new region of stationary phase. In isothermal GC, the thermodynamics of the partitioning process between the mobile phase and the stationary phase is governed by the enthalpy of vaporization (the change in heat content) for the analyte from the stationary phase into the mobile phase. For the alkanes seen in Figure 1, the enthalpy of vaporization increases linearly as each -CH₂- unit is added to the carbon chain. Through the Gibbs equation, which relates enthalpy and temperature, this results in an exponential increase in K and k, leading to an exponential increase in retention time. The full theory and thermodynamics are discussed elsewhere in more detail with the relevant equations (3–5).

In temperature programming, the column temperature usually increases linearly with time as the separation proceeds. This has the effect of increasing the vapour pressure and decreasing k of the analytes with time. From general and physical chemistry courses, we know that vapour pressure increases exponentially with temperature, with the linearized form of the relationship expressed by the Clausius-Clapeyron equation:

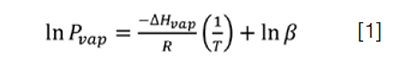
where P_vap is the vapour pressure, ΔH_vap is the enthalpy of vaporization, R is the gas constant, T is the temperature in degrees Kelvin, and β is ΔS/R. Gas chromatographers often use a similar expression, the van’t Hoff equation, which relates the equilibrium constant for a reaction and the temperature, assuming a constant change in enthalpy ΔH, and entropy ΔS:

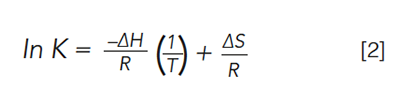
where K is the partition coefficient, which in GC is related to the retention factor (k) by the phase ratio (β), the ratio of the volume of the stationary phase to the volume of the mobile phase. In 1963, less than 10 years after the initial inception of GC, Giddings provided a model for temperature programming, depicted in Figure 3, based on the Clausius-Clapeyron equation, modified for the specific case of GC and on relationships derived previously by Dal Nogare and Harris and Habgood (6–9). One equation for describing temperature programming and relating it to the same thermodynamic quantities as seen in isothermal GC and derived by Harris and Habgood is seen in equation 3.

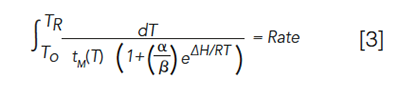
where T_o is the initial temperature of the temperature program, T_R is the elution temperature of the analyte, t_M(T) is the gas hold-up time at temperature T, α = ΔS/R, and β is the column phase ratio. Rate is the slope (°C/min) of the linear temperature program. Equation 3 must be solved numerically for the second integration constant, which provides the elution temperature of the analyte (easily translated into retention time using the starting temperature and programming rate), and provides the basis for several of the computer simulation programs for GC that have been developed over the years (10,11). For computer simulations of GC, ΔH and ΔS are easily measured and have been termed thermodynamic retention indices (12). With knowledge of these for a given analyte and stationary phase, and equation 3, it is possible to predict the retention time of any analyte on that same stationary phase under any conditions.

In Figure 3, the exponential curve describes the rate of zone or peak migration as the column temperature in increased. This exponential curve resembles a vapour pressure curve, and can be approximated as such, with the addition that acceleration of the analyte along the column is faster than predicted by vapour pressure alone, due to expansion of the carrier gas as it travels from the higher pressure at the column inlet to the lower pressure at the outlet. This demonstrates that, as the column temperature is increased, the peak accelerates as it simultaneously travels along the column because its vapour pressure increases and the carrier gas is expanding inside the column as it flows from the inlet to the outlet.

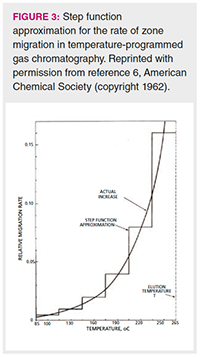
In order to simplify the model, the exponential curve is broken up into six 30 °C steps, a so-called “step approximation” for temperature programming. As seen in Figure 3, with temperature programming conditions, in contrast to isothermal conditions, analytes move slowly when first injected, and accelerate exponentially as the temperature is increased and the chromatographic run proceeds. This exponential acceleration has the practical effect of linearizing the relationship between carbon chain length and retention time for the n-alkanes, as seen in Figure 1.

As an example of using the step approximation, Giddings described a temperature program from 85 to 265 °C, with the steps being six 30 °C intervals. He demonstrated that 50% of a peak’s migration down the column occurs in the final 30-degree segment. In short, the peak travels about half of the column length in the last 1/6 of the retention time, and about 3/4 of the column length in the last 1/3 of the total retention time. Likewise, at the beginning of the run, the peak travels about 1/64 of the column length in the first 1/6 of the retention time, and about 3/32 of the column length in the first 1/3 of the retention time.

From this discussion, we see that retention times in temperature‑programmed GC are based on the same thermodynamic quantities as in isothermal GC. However, in temperature programming, in contrast to isothermal conditions, the relationship between the enthalpy of vaporization and the retention time becomes linear, due to the linear increase in temperature giving rise to an exponential increase in vapour pressure, as seen in the Clausius‑Clapeyron equation, or in K as seen in the van’t Hoff equation. This explains the first aspect of the temperature programmed chromatogram seen in Figure 1: alkane peaks are evenly spaced.

Turning to the second observation about Figure 1, that all of the peaks in the temperature-programmed run are of similar width, the step approximation can help explain that, as well. Giddings developed the step approximation so that the six segments could be further approximated as isothermal segments at the mean temperature of the segment. This allows us to think about each segment as a single isothermal portion of the run, and to apply the Golay equation, shown below in abbreviated form, to the conditions in each segment:

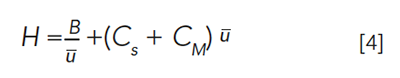
where H is the height equivalent to a theoretical plate, B is related to solute diffusion rates in the mobile phase, C_S and C_M are related to mass transfer rates in the stationary and mobile phases, respectively, and Å« is the average velocity of the carrier gas. A full explanation of the Golay equation and the principles related to the kinetics of band broadening is provided in references 4 and 5. The Golay equation reminds us that the rate of band broadening (expressed by H, the height equivalent to a theoretical plate) is a consequence of diffusion in the mobile phase, mass transfer in the mobile phase, and mass transfer in the stationary phase, as well. Simplified views of diffusion and mass transfer are shown below in Figure 4.

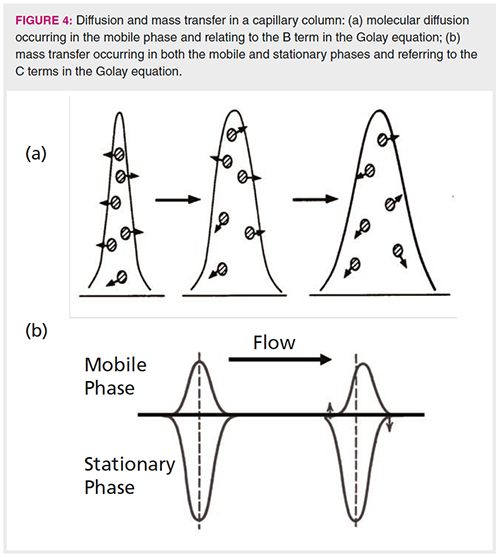
From the Golay equation, we see that band broadening caused by diffusion in the mobile phase, illustrated in Figure 4(a), is inversely related to the average linear carrier gas velocity. Using the step model, we see that, in the first segments immediately following the injection, the bulk of analyte molecules reside in the stationary phase, so they are not affected much by mobile phase diffusion. As the separation proceeds in the later segments, the bulk of analyte molecules are in the mobile phase, and move very rapidly as they approach the column outlet. This minimizes band broadening due to molecular diffusion because of the inverse relationship with the carrier gas velocity.

Next, we turn to band broadening due to mass transfer, which is somewhat more complicated, but can be explained using similar logic. In capillary columns, there are terms related to mass transfer in both the mobile and stationary phases. In this case, the rate of band broadening is directly proportional to the average velocity of the carrier gas, and is related to k and the respective mobile phase and stationary phase diffusion constants, illustrated in Figure 4(b). On the left side of the figure, a symmetrical peak with k = 3 is shown, with its relative portions in both phases. On the right side of Figure 4(b), the peak is seen to distort, or spread, caused by the mass in the mobile phase being shifted down the column (to the right in the figure), followed by the resulting evaporation of new analyte on the left of the figure.

The step approximation is useful here, as well. In the early steps, with the bulk of analyte molecules in the stationary phase, mass transfer is limited by the low temperature at the start of the temperature program. The analyte band is essentially “frozen” in place. In later segments, when the bulk of the analyte molecules are in the mobile phase, the time spent to traverse 50% of the column is short-1/6 of the total retention time-limiting the effect of mass transfer in the mobile phase. For mass transfer in the stationary phase, k gets smaller as the temperature increases, pushing the analyte more and more out of the stationary phase into the mobile phase, also limiting stationary phase mass transfer. In short, the narrow initial band, once it starts to move, accelerates and moves very quickly along most of the column length, minimizing the time for significant mass transfer as it is moving. The Golay equation and the step approximation together explain why the peaks are all sharp and about the same width in temperature-programmed GC.

The step approximation also provides a useful explanation for many practical aspects of temperature-programmed GC beyond the appearance of the chromatogram in Figure 1. Best practice in performing splitless injections provides a good example. The basics of splitless injection were recently reviewed in this column, and are the subject of an excellent review and book by Konrad Grob, so they will not be reviewed again in detail here (13–15). For this discussion, the important principles in splitless injections are that the injection process itself may require up to 60 s to complete, and splitless injection is always used in combination with temperature programming. Despite the long time required for the injection process, the peaks seen in separations that employ splitless injection are often very sharp. The step model of temperature programming can help to explain this phenomenon.

In a splitless injection, there are two peak focusing mechanisms at work once the sample reaches the column: solvent effects, and thermal focusing or “cold trapping”. The step approximation explains how both can work in combination with temperature programming to generate sharp peaks. First, assume a splitless injection in combination with a temperature program that starts at a temperature well below the boiling point of the sample solvent, and even further below the boiling points of the analytes. For example, if using hexane as solvent, which has a normal boiling point of 68 °C, I start my temperature programs at 40 °C. As the sample and solvent transfer into the column, the low initial column temperature causes the solvent to condense as a long plug of liquid at the head of the column. Analytes with higher boiling points or strong affinity for the stationary phase will be strongly retained by the stationary phase. This is cold trapping. Analytes with lower boiling points or stronger affinity to the solvent will be initially retained in the solvent plug, followed by retention as a narrow initial band on the stationary phase as the solvent evaporates. This is the solvent effect. A detailed description of solvent effects is provided in the text and article by Grob (14,15)

The step approximation applies to splitless injections whether the peaks are refocused by solvent effects or by cold trapping. All the peaks are broadened as the splitless injection process proceeds during the initial purge-off time, with the peak width determined by the length of the purge-off time. The cold initial column temperature effectively condenses the analytes into a narrow band at the column head. As the column is heated, the analytes begin to move down the column one by one, determined by their heat of vaporization from the stationary phase to the mobile phase. The process is similar for solvent effects, except that the initial bands are focused by evaporation of the solvent plug during the early stages of the separation. As an example, picture two analytes, one with a retention time of 12 min, and
one with a retention time of 18 min. When the first analyte elutes after 12 min, the second analyte will have travelled about 1/4 of the column length. It will travel the final 3/4 of the column in the remaining 6 min. As discussed above, this process of refocusing, either by cold trapping or solvent effects, followed by temperature programming, keeps all of the peaks in a splitless injection sharp.

Temperature-programmed GC has been in common use for about six decades, and continues to be among the most powerful, yet easy to use, high resolution separation methods available. However, much of the theory of GC was developed assuming isothermal conditions, and continues to be discussed on that basis. The theory of temperature-programmed GC is much more complex than for isothermal GC, but is still based on the same fundamental thermodynamic and kinetic principles. In temperature programmed GC, retention time relates linearly to the enthalpy of vaporization, while in isothermal GC the relationship is exponential. Combined with the high temperature stability of columns, this allows a wide range of analytes to be separated in a single run. The temperature-program step approximation provides a simple means for understanding how the peaks in temperature programmed GC remain sharp throughout the run, based on acceleration of the migration rate along the column as the temperature program proceeds. These principles make temperature-programmed capillary GC still the most powerful chromatographic separation technique available today.

References

1. S. Dal Nogare and J.C. Harden, Anal. Chem.31(11), 1829–1832 (1959).

1. N.H. Snow, LCGC Europe31(11), 616–623 (2018).

1. N.H. Snow, J. Chem. Educ.73(7), 592–597 (1996).

1. L.M. Blumberg, Gas Chromatography, C.F. Poole, Ed. (Elsevier, Amsterdam, The Netherlands, 2012), pp. 19–78.

1. H.M. McNair, and J.M. Miller, Basic Gas Chromatography (John Wiley and Sons, New York, New York, USA, 2nd ed., 2008), pp. 29–52.

1. J.C. Giddings, J. Chem. Educ.39, 569–573 (1962).

1. H.W. Habgood and W.E. Harris, Anal. Chem. 32, 450–453 (1960).

1. H.W. Habgood and W.E. Harris, Anal. Chem.32, 1206 (1960).

1. S. Dal Nogare, Anal. Chem.35, 19R–25R (1960).

1. N.H. Snow and H.M. McNair, J. Chromatogr. Sci.30, 271–275 (1992).

1. Pro EZGC Chromatogram Modeler https://www.restek.com/proezgc (Accessed May 16, 2019).

1. E. Dose, Anal. Chem.59, 2414–2419 (1987).

1. N.H. Snow, LCGC Europe31(7), 378–384 (2018).

1. K. Grob, Split and Splitless Injection for Quantitative Gas Chromatography: Concepts, Processes, Practical Guidelines, Sources of Error (John Wiley and Sons, New York, New York, 4th. ed., 2008).

1. K. Grob, Anal. Chem.66(20) 1009A–1019A (1994).

Nicholas H. Snow is the Founding Endowed Professor in the Department of Chemistry and Biochemistry at Seton Hall University, and an Adjunct Professor of Medical Science. He is interested in the fundamentals and applications of separation science, especially gas chromatography, sampling, and sample preparation for chemical analysis. His research group is very active, with ongoing projects using GC, GCÐMS, two-dimensional GC, and extraction methods including headspace, liquid–liquid extraction, and solid-phase microextraction.

“GC Connections” editor John V. Hinshaw is a Senior Scientist at Serveron Corporation in Beaverton, Oregon, USA, and a member of LCGC’s editorial advisory board. Direct correspondence about this column to the author via e-mail: LCGCedit@mmhgroup.com