HPLC Column Transfer from Fully Porous to Core–Shell Particles in Three Simple Equations

LCGC blogger Tony Taylor describes how to easily transfer column dimensions and particle morphology using three equations.

Photo Credit: Westend51/Getty Images

There are often times in my work when I need to “mess about” with column dimensions and particle morphologies. For “mess about” read improve or transfer.

There are many on-line calculators to do this; however, I never really found one which worked as well or as intuitively as the simple maths which follows here. I actually have this programmed into an Excel spreadsheet (write to enquiries@crawfordscientific.com if you would like a copy). However, it’s great to work through the simple maths in order to really understand what is going on. As I will show you below, this calculation, while derived from chromatographic theory, is actually very easy to use and the variables are all to hand.

On a serious note - when “messing about” with high performance liquid chromatography (HPLC) methods and changing column dimensions and/or particle morphologies (from fully porous to core–shell type columns for example), if one does not change the gradient steepness, then there may be SIGNIFICANT changes not only to retention times but also to the selectivity (and resolution) between peaks within the chromatogram. You therefore absolutely need to use the equations below (or a similar method) in order to get equivalent separations - incidentally you will also need to use our equations below to maintain the eluent linear velocity.   So - here goes. I would like to transfer the following method; C18 fully porous particle column 150 mm × 4.6 mm, 3.5-µm with an eluent flow rate of 1.0 mL/min and a gradient of 20% B to 60% B in 10 min (Figure 1[a]) to a C18 core–shell column 100 mm × 2.1 mm, 2.7-µm using the same eluotropic range (20% B to 60% B) (Figure 1[b]).   Here are the equations we will use to achieve the transfer: (1) Flow rate transfer to maintain eluent linear velocity between columns:  

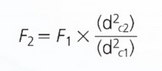
[1]

F

= flow rate (mL/min)

d

_c

= column diameter (mm) 1 = original column parameter 2 = new column parameter     (2) Equation to calculate the new gradient time to maintain relative retention (that is, selectivity):    

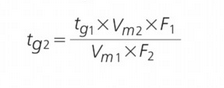
[2]

t

_g

= gradient time (min)

V_m

= interstitial volume of the column (µL)

F

= flow rate (mL/min)   (3) Calculation of the column interstitial (dwell) volume:    

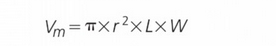
[3]

r

= column radius (mm)

L

= column length (mm)

W

= column % interstitial porosity   The only approximation that you need to know

W

(fully porous materials) ≅68% ≅0.68

W

(core–shell particles) ≅ 55% ≅ 0.55   If you want to be sure of these numbers  contact your column supplier; however, these approximations are usually accurate enough.  

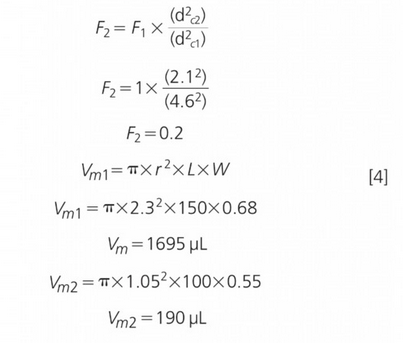
So - lets go with the translation: C18 fully porous particle 150 mm × 4.6 mm, 3.5-µm with an eluent flow rate of 1.0 mL/min. with a gradient of 20% B to 60% B in 10 min to a C18 core–shell column 100 mm × 2.1 mm, 2.7-µm using the same eluotropic range (that is, 20% B  to 60% B)   OK - now we have everything we need for the calculation:  

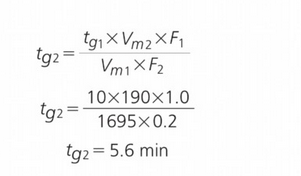
[5] So - the new method is as follows: C18 core–shell column 100 mm × 2.1 mm, 2.7‑µm at an eluent flow rate of 0.2 mL/min with a gradient time (20% B to 60% B) of 5.6 min.   With these very simple equations we have been able to derive new conditions to enable us to achieve a faster or high efficiency separation with a new column dimension and particle morphology.   E-mail: bev@crawfordscientific.com/colin@crawfordscientific.com Website:

www.chromacademy.com