Impact of Particle Size Distribution on HPLC Column Performance

In this article, controlling the particle size distribution (PSD) variable has been examined as a possible route to further improvement in performance of particle-based columns.

Silica particles prepared in tubular column formats dominate high performance liquid chromatography (HPLC), and the technique would not be as important without them. Over the years, silica particles have been optimized in shape, purity, and size. They have steadily become smaller to improve efficiency, which allows for more speed, sensitivity, and resolution at the cost of higher pressure. Knox (1) and others published early papers showing accurate insight into particle and pressure requirements for improving HPLC speed and performance.

When discussing column performance, it is always helpful to start with the van Deemter relationship, which describes three additive processes that influence bandspreading within the column:

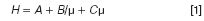
H stands for height equivalent to one theoretical plate (HETP) and is calculated from a measurement of column efficiency, N, and the relationship, H = L/N, where L is column bed length. The μ term is the linear velocity of the mobile phase. A plot of H versus μ is commonly called a van Deemter curve and represents how efficiency behaves as a function of μ (proportional to flow rate). The lower the H value, the greater efficiency a column displays. In equation 1, the A term represents contributions from flow and diffusion processes within the mobile phase flowing around the particles (names include eddy diffusion, eddy dispersion, flow inequality, and multipath term), the B term is total axial (longitudinal) diffusion within mobile and stationary phases, and the C term represents speed of mass transfer between mobile phase and stationary phase that lies mainly within particle pores. If a narrower particle distribution can create more uniform beds, it should show up as a smaller A term, which is not very sensitive to flow velocity compared to the other two terms. The A term dominates in the middle of the velocity curve and controls the lowest value of plate height at which efficiency of a column is highest, while the B term dominates at low velocity and the C term dominates at high velocity. A complete discussion of factors affecting band dispersion is beyond the scope of this article and for an in-depth discussion of van Deemter relationships, refer to Neue (2) and other excellent HPLC books.

Typical van Deemter curves are shown in Figure 1 for several sizes of porous C18 silica to demonstrate the twofold advantage of selecting smaller particles (3). Not only can smaller H (larger N) be achieved by using smaller particles, this better column performance can be sustained even at higher velocities (smaller slope for the C term). Note that Figure 1 uses interstitial linear velocity rather than classical linear velocity, which is more easily obtained from the relationship, μ = L/t₀, where L is column bed length and t₀ is the time for an unretained peak. Neue (2) and other texts provide definitions of different linear velocity terms and other HPLC column fundamentals. Plots of H (or h, called the reduced plate height, H/d_p where d_p is the average particle diameter in the same units as H) against classical linear velocity are usually adequate and will be used in this article. Reduced plate height allows performance to be compared when columns use different particle sizes. Until modern core-type particles were introduced in 2006, the lowest h_min values observed for silica particles were 2–3.

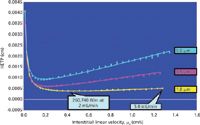
Figure 1: A van Deemter plot for small porous particles. Columns: 50 mm × 4.6 mm Zorbax Eclipse XDB-C18 (1.8-μm column was 30 mm in length); eluent: 85:15 acetonitrile–water; flow rates: 0.05–5.0 mL/min; temperature: 20 °C; sample: 1.0 μL octanophenone in eluent. (Courtesy of Ron Majors and Agilent Technologies).

Although Figure 1 creates the impression that nearly unlimited separation speed might be possible with sub-2-μm particle columns, column resistance and instrument pressure rating quickly become limiting factors. Measurement of H-μ (or h-μ) curves with simple, ideal solutes is very useful in column research; however, real samples contain solutes that may vary greatly in curve shape. When high speed methods are being developed, kinetic performance with H-μ plots should be compared for both ideal and target solutes during column screening. For example, the best performing column with toluene or naphthalene may not yield equally good performance for drug metabolites. Plots of column efficiency, N, and resolution, R, against linear velocity can also be very valuable during the development of a high-speed method. Column stability towards high flow and pressure conditions should also be confirmed. Figure 2 illustrates how well modern HPLC columns can operate at very high linear velocity (circa 20 mm/s) under gradient conditions (4). Gradients are often used in high-throughput assays to clean the column between sample injections. The 20 mm × 2.1 mm C18 column prepared with 5-μm core-type silica particles required a starting system pressure of 172 bar (78 bar from the column and 94 from the ultrahigh-pressure liquid chromatography [UHPLC] instrument).

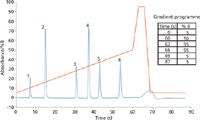
Figure 2: Fast gradient assay with a 5-μm core-type column. Column: 20 mm × 2.1 mm, 5-μm dp Ascentis Express C18; mobile-phase A: water with 0.1% trifluoroacetic acid; mobile-phase B: acetonitrile with 0.1% trifluoroacetic acid; mobile phase: 11:89 A–B; flow rate: 2 mL/min; pressure: 172 bar; temperature: 40 °C; detection: UV absorbance at 254 nm; injection volume: 0.5 μL; flow cell: 1-μL micro. Peaks: 1 = atenolol, 2 = pindolol, 3 = propranolol, 4 = indoprofen, 5 = naproxen, 6 = coumatetralyl. (Courtesy of Advanced Materials Technology).

New insight about van Deemter relationships was provided by Knox (5,6), who suggested that the A term is much more important than previously thought and should receive more attention as a path to improved HPLC column performance. According to Knox, a lower A term will come from better column preparation techniques and more uniform bed structures. In this article, the particle size distribution (PSD) variable has been examined as a possible route to higher performance for particle-based columns.

Experimental

HPLC column preparation can involve a huge number of variables, making it difficult to draw accurate conclusions from experiments with particles unless preparation variables are optimized or at least held constant. Another important variable for studying high performance columns is selecting a suitable instrument that measures true bed efficiency by minimizing extracolumn bandspreading. This article focuses on the impact of PSD and assumes that variables such as column preparation and testing have been controlled and optimized to an acceptable degree.

Different methods for measuring and reporting particle size and distribution are described by Bartle and Myers (7) and Horiba (8). Unless otherwise specified, this article will use the common D_90/10 method for reporting distribution. D_90/10 is defined as the particle diameter (d_p) at 90% of the distribution (a larger number) divided by d_p at 10% of the distribution (a smaller number). Particles used for preparing HPLC columns can range from values less than 1.1 to 1.5 or more. Figure 3 shows distribution data for six spherical porous silica samples taken from commercial HPLC columns. As shown in the next section, column performance with spherical particles in broader size ranges is still quite good and allows the HPLC technique to solve many important analytical problems.

Figure 3: Size distribution for several silica HPLC column particles. The D90/10 value is shown above the profiles. (Unpublished data supplied by Supelco division of Sigma-Aldrich).

Results and Discussion

Important benefits to achieving even more uniform column beds should include providing very consistent column performance and greater operating stability. A near-perfect bed should be resistant to settling or voiding and would presumably fail only because of contamination or loss of stationary phase (a factor of temperature and pH). While progress in silica particle development continues, parallel efforts are underway to accomplish similar performance and stability objectives using monolithic silica beds and fabricated pillar arrays. Results described in this article have been taken from both published and unpublished sources.

Verzele (9) investigated C18 column performance under reversed-phase conditions using spherical silica samples having relatively narrow distribution (D_90/10 = 1.5). He blended samples to deliberately broaden the distribution and measured column performance before and after blending. Verzele did not observe significant differences in h_min between blended and unblended samples, but he did note that particles blended to create broader distribution showed greater flow resistance (pressure drop) and lost performance slightly faster at higher flow velocity (higher slope for C term). Impedance reached a maximum with a 50/50 blend of 8- and 3-μm particles that was about 50% greater than a column prepared with only narrow-distribution 3-μm particles. Verzele recommended that HPLC columns be prepared with D_90/10 distributions of 1.5–2.0. Important conclusions that can be drawn from these early experiments on PSD is that good columns can be prepared with broader distribution samples, but at the price of higher pressure. This is an important point because pressure has become a critical factor in achieving higher separation speed with sub-2-μm particle columns.

Kirkland pioneered core-type silica particles (10–13) and described the first narrow-distribution silica in 2007 (14,15), which showed extremely low h_min values of about 1.5. The particle standard deviations were very narrow at about 5% relative standard deviation (RSD) (equivalent to a D_90/10 value of 1.1) compared to typical values for other HPLC particles at that time of about 15–20% RSD (equivalent to D_90/10 values of about 1.4). Columns with greatly improved performance could be prepared with larger 2.7-μm particles and used with traditional 400-bar instruments, which was welcome news to those faced with changing to sub-2-μm particles and expensive UHPLC instruments. Since that breakthrough in particle design, there has been considerable effort to determine why columns with core-type particles exhibit such high performance compared to columns with porous particles. Core-type particles showed improvements (lower values) over totally porous particles in all three parts of the van Deemter equation, with the most significant advantage being in the A term, which is usually associated with bed uniformity. The much narrower particle distribution might be a factor in creating such a low A term; however, Guiochon (16) proposed that other particle properties such as surface roughness are a more likely cause than narrow PSD for the highly uniform beds.

The impressive performance of core-type particles reopened the debate among academic scientists about the importance of narrow particle size distribution to column efficiency. Desmet (17) studied four porous and three core-type commercial particles having about 3 μm diameter (distribution ranged from about 5% to 25% RSD) and reported strong correlations of about 0.9 between particle distribution and several different measures of column performance. Measurements that Desmet related to bed quality included the following: h_min, the A term constant, and column impedance. Based on fitted-line plots, h_min ranged from 1.5 to 2.5; the A term ranged from about 0.6 to 1.2; and separation impedance ranged from 1000 to 2500. Core-type particles with the lowest RSD had the highest performance, while the porous packing with lower RSD had higher performance within the porous group. Desmet noted that the extra performance of core-type particles could also be influenced by more subtle features such as higher particle density and surface roughness. Desmet suggested that porous particles with the same narrow distribution of core-type particles should be developed and studied. Desmet (18) also examined the impact of adding 3-μm porous particles to narrow-distribution 1.9-μm porous silica and concluded that the addition of larger particles could not be expected to improve column kinetic performance because of pressure elevation; however, he reported that up to 25% of the larger particles could be added without having a major negative impact on band broadening and efficiency. This suggested that narrow PSD alone may not be responsible for the high performance of core-type particles. Guiochon (19) concluded in a different study blending 3- and 5-μm porous particles that, as long as the distribution did not exceed 40% RSD, it did not have a negative impact on band broadening and efficiency. He further proposed that intentionally adding some larger particles to smaller ones to broaden the distribution could actually improve column efficiency for small molecules, but did not discuss the negative impact that a broadened PSD might have on column permeability and kinetic performance.

Barber (20) noted that previous studies into how particle properties can impact column performance had focused on particles having the same shape. He described the blending of narrow PSD (7% RSD) porous silica spheres with twins or dimers to determine how much dimer could be present in the silica process before it negatively impacted column performance. Barber observed that up to 20% of the larger dimer particles could be added without observing a negative impact on efficiency. Contrary to results of previous experiments with blending spheres to broaden distribution, column permeability actually improved when dimers were blended with spheres, suggesting that the presence of some dimers was beneficial.

Figure 4: Performance comparisons for (a) two particles with narrow distributions and one with broader distribution and (b) three porous particles with the same average size and different distributions. (a) Toluene data for 50 mm × 3.0 mm columns with 60:40 acetonitrile–water mobile phase. (b) Test conditions shown in the figure. (Unpublished data supplied by Supelco division of Sigma-Aldrich).

Henry (21) described results for the sub-2-μm porous silica shown in Figure 3 that has the same narrow distribution as core-type particles (D_90/10 = 1.14). Figure 4(a) compares plots of reduced plate height, h, for narrow distribution porous and core-type silica particles to broader distribution porous silica. While sampling only a limited number of commercial columns, a lower overall h value observed for monodisperse, porous silica over polydisperse, porous silica supports the argument that narrow PSD can provide a performance edge (for toluene in this example), whether because of a narrower particle distribution or being easier to prepare into a uniform bed. The narrow PSD porous particle achieved nearly identical performance to the core-type particle in the middle velocity region where the A term (bed uniformity) in equation 1 dominates. The core-type particle showed expected performance advantages over both porous particles at lower velocities (where the B term dominates) and higher velocities (where the C term dominates).

Table 1: Pressure comparison for particles with different distributions: three porous particles with same average size* (courtesy of Supelco division of Sigma-Aldrich).

Figure 4(b) compares a column with narrow-distribution porous silica with two commercial columns that use porous particles with the same spherical shape and average diameter, but broader distribution. As shown, lower h_min values and better performance for three neutral solutes over the entire flow range were observed for the column with narrow-distribution silica. Table 1 also confirms that the column with narrow-distribution silica has higher permeability (lower pressure drop or impedance) than those with broader distribution. Figure 5 demonstrates near-equivalency of narrow distribution, sub-2-μm porous and core-type particles using fast 1-min gradient separations on 50 mm × 3.0 mm columns. Although plate height or efficiency cannot be readily compared under gradient conditions, peak width and resolution results indicate that columns are very comparable in performance.

Figure 5: Comparison of high-speed liquid chromatography–mass spectrometry (LC–MS) gradients with sub-2-μm monodisperse porous and core-type silica columns. Column dimensions: 5 cm × 3.0 mm; mobile-phase A: 0.1% formic acid in 95:5 water–acetonitrile; mobile-phase B: 0.1% formic acid in 5:95 water–acetonitrile; gradient: 35–60% B in 1 min, then 60% B for 0.5 min; flow rate: 0.6 mL/min; column temperature: 35 °C; detection: time-of-flight MS, ESI+, XIC, 100–1000 m/z; injection volume: 2 μL; sample: 300 ng/mL in 97:3 water–methanol. Peaks: 1 = oxazepam glucuronide (463 m/z), 2 = lorazepam glucuronide (497 m/z), 3 = temazepam glucuronide (477 m/z), 4 = oxazepam (287 m/z), 5 = lorazepam (321 m/z), 6 = temazepam (301 m/z). (Unpublished data supplied by Supelco division of Sigma-Aldrich).

DeStefano (22) compared the performance of columns prepared with 2.7- and 4.1-μm core-type particles with narrow distribution (D_90/10 of about 1.1) to a column made with a 50:50 blend by weight. Results agreed with previously published data for porous particles that efficient columns could be made with either narrow PSD or broadened PSD core-type silica; however, pressure drop was higher for a column made with blends. Although it may be possible to achieve about the same column efficiency with a broader particle distribution having the same average diameter, separation speed would be more limited because of higher flow resistance. Results are shown in Figure 6 and Table 2.

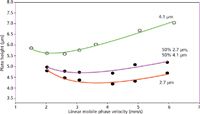
Figure 6: Plate height (H) versus velocity (μ) plots for single and mixed core-type particles in 50 mm × 3.0 mm columns. A 50:50 (w/w) mix of 2.7- and 4.1-μm particles produces plate heights intermediate to the single components. The reduced plate heights are about equal. Data are fitted to the Knox equation. (Unpublished data supplied by Advanced Materials Technology).

Several other sources of valuable information were used (23–26). Clearly, there is still considerable disagreement about the best particle design for preparing optimum HPLC columns. It should become easier to establish ideal particle properties and optimum column preparation methods as narrower particle distributions become available, computer simulation methods improve, and new bed visualization techniques are developed.

Table 2: Comparison of 50 mm × 3.0 mm column performance for single-sized core-type particles and a mixture* (courtesy of Advanced Materials Technology).

Summary and Conclusions

If narrow-PSD particles can be made inexpensively, whether they are porous or core-type, there seem to be advantages to using them. A clear relationship exists between narrower PSD and lower column pressure; however, there is still considerable disagreement about whether narrower distribution leads directly to more uniform beds and better HPLC column efficiency. While steady improvements are being made, it is not clear how much performance comes from better particle design or better column preparation techniques. In the absence of any real negatives, particles for HPLC columns are likely to evolve towards narrower distribution. The following points can be taken from the experiments and data reviewed in this article:

• For the same average particle size and shape, a broader distribution will generate more flow resistance, presumably because smaller particles can fill into gaps between larger ones.

• With good column preparation techniques, broader distribution particles have achieved comparable efficiency in many cases to particles with narrower distribution; however, higher pressure drop will limit those columns to lower flow velocities and be detrimental to kinetic performance. For the same average particle size and comparable column efficiency, a narrow particle distribution results in significantly lower pressure at the same flow velocity.

• Verzele noted a small negative impact on performance of broader distribution particles at high flows; while logical, further studies are needed with all particle types to confirm this behaviour and establish its importance.

• New porous silica particles with the same narrow distribution of core-type particles have shown performance, measured by h_min, that is better than broader-distribution porous particles and comparable to core-type particles when compared by their reduced plate heights or when gradients are used for separation; having these particles for direct comparison should help establish whether unique core-type particle performance is because of narrow PSD, surface roughness, higher particle density, or something else. Whatever the reason for the high performance of core-type particles, they are widely available and have become well established in methods, so they are here to stay.

• A major weakness of preparing beds from loose particles is that they can move and settle during use and thereby lose performance; more studies about the impact of particle distribution on column bed stability are needed.

• Techniques for direct visualization of particle uniformity within the bed will be very valuable in designing ideal particles and standardizing column preparation techniques.

Although much is being learned about designing particles and preparing uniform, efficient particle beds, only part of HPLC column performance can be understood by studying the A term and flow uniformity around particles. Even with a perfect column bed, slow mass transfer processes within the particles and a positive slope for the C term will increase H and reduce overall column performance, especially at high flow velocities. The C term may be even more difficult to optimize than the A term because we do not know very much about particle pore structure or the stationary-phase environment.

Acknowledgements

Private discussions and unpublished data were provided by Joseph DeStefano of Advanced Materials Technology; David Bell, Hugh Cramer, William Campbell, Stacy Squillario, and William Betz of the Supelco division of Sigma-Aldrich; Ron Majors, "Column Watch" and "Sample Prep Perspectives" Editor for LCGC Europe; William Barber of Agilent Technologies; and Peter Myers of the University of Liverpool, UK.

Zorbax is a registered trademark of Agilent Technologies; Fused-Core is a registered trademark of Advanced Materials Technology, Inc.; and Titan is a trademark of Sigma-Aldrich Company, LLC.

Richard A. Henry received his BS in Chemistry from Juniata College (Huntingdon, Pennsylvania, USA) and his PhD in Analytical Chemistry from The Pennsylvania State University (Pennsylvania, USA). After postdoctoral work at Purdue University, he joined DuPont at the Experimental Station in Wilmington, Delaware, USA, and worked with Dr. Jack Kirkland and others in early HPLC column and instrument development. He later became Director of Analytical Laboratories at Penn State University and also founded Keystone Scientific, Inc., to manufacture HPLC column products. Dick remains active teaching short courses on separation technology and as a consultant. Direct correspondence to: hyperlc@comcast.net

References

(1) J.H. Knox and M. Saleem, J. Chromatogr. Sci. 7, 614–622 (1969).

(2) U.D. Neue, HPLC Columns, Theory, Technology and Practice (Wiley-VCH, Weinheim, Germany, 1997).

(3) R.E. Majors, "Directions in HPLC Technology- Post Verzele," presented at the International Symposium on HPLC, Ghent, Belgium, 2007.

(4) S.A. Schuster, T.J. Waeghe, W.L. Johnson, J.J. DeStefano, and J.J. Kirkland, "Larger Superficially Porous Particles," presented at the Eastern Analytical Symposium, Somerset, New Jersey, USA, 2013.

(5) J.H. Knox, J. Chromatogr. A 831, 3–15 (1999).

(6) J.H. Knox, J. Chromatogr. A 960, 7–18 (2002).

(7) K.D. Bartle and P. Meyers, Capillary Electrochromatography, in RSC Chromatography Monographs, R.M. Smith, Series Ed. (Royal Society of Chemistry, 2001), chapter 3.

(8) Horiba Instruments, Inc., A Guidebook to Particle Size Analysis, www.horiba.com/us/particle (2012).

(9) C. Dewaele and M. Verzele, J. Chromatogr. 260, 13–21 (1983).

(10) J.J. Kirkland, Anal. Chem. 41, 218–220 (1969).

(11) J.J. Kirkland, Anal. Chem. 64, 1239–1245 (1992).

(12) J.J. Kirkland, J. Chromatogr. A 890, 3–13 (2000).

(13) J.J. Kirkland, J. Chromatogr. Science 38, 535–544 (2000).

(14) J.J. Kirkland, T.J. Langlois, and J.J. DeStefano, Am. Lab. 39, 18–21 (2007).

(15) J.J. DeStefano, T.J. Langlois, and J.J. Kirkland, J. Chromatogr. Sci. 46, 254–260 (2008).

(16) F. Gritti, I. Leonardis, D. Schock, P. Stevenson, A. Shalliker, and G. Guiochon, J. Chromatogr. A 1217, 1589–1603 (2010).

(17) D. Cabooter, A. Fanigliulo, G. Bellazzi, B. Allieri, A Rottigni, and G. Desmet, J. Chromatogr. A 1217, 7074–7081 (2010).

(18) A. Liekens, J. Billen, R. Sherant, H. Ritchie, J. Denayer, and G. Desmet, J. Chromatogr. A 1218, 6654–6662 (2011).

(19) F. Gritti, T. Farkas, J. Heng, and G. Guiochon, J. Chromatogr. A 1218, 8209–8221 (2011).

(20) W.E. Barber, B.A. Bidlingmeyer, and X. Wang, "Interrelations Between Particle Properties and Chromatographic Performance," presented at the International Symposium on HPLC, Anaheim, California, USA, 2012.

(21) R.A. Henry, W.R. Betz, W.H. Campbell, G. Parmar, W.K. Way, and P. Ross, "Development of a New Monodisperse Porous Silica for UHPLC," presented at the International Symposium on HPLC, Anaheim, California, USA, 2012.

(22) J.J. DeStefano, S.A. Schuster, R.S. Bichlmeir, and W.L. Johnson, "Particle Size Considerations in Superficially Porous Particles," presented at the Eastern Analytical Symposium, Somerset, New Jersey, USA, 2013.

(23) A. Daneyko, A. Holtzel, S. Khirevich, and U. Tallarek, Anal. Chem. 83, 3903–3910 (2011).

(24) S. Bruns, J.P. Grinias, L.E. Blue, J. Jorgenson, and U. Tallarek, Anal. Chem. 84, 4496–4503 (2012).

(25) K.K. Unger, Porous Silica — Its Properties and Use as Support in Column Liquid Chromatography (Elsevier Scientific Publishing Co., Amsterdam, Oxford, New York, 1979).

(26) R.K. Iler, The Chemistry of Silica (John Wiley & Sons, Hoboken, New Jersey, USA, 1979).